Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Aguilar, Konrad
Hartglass, Michael
and
Penneys, David
2022.
Compact quantum metric spaces from free graph algebras.
International Journal of Mathematics,
Vol. 33,
Issue. 10n11,
Aguilar, Konrad
Kaad, Jens
and
Kyed, David
2022.
The Podleś Spheres Converge to the Sphere.
Communications in Mathematical Physics,
Vol. 392,
Issue. 3,
p.
1029.
Aiello, Valeriano
Guido, Daniele
and
Isola, Tommaso
2022.
Spectral triples on irreversible C∗-dynamical systems.
International Journal of Mathematics,
Vol. 33,
Issue. 01,
Austad, Are
Kaad, Jens
and
Kyed, David
2024.
Quantum metrics on crossed products with groups of polynomial growth.
Transactions of the American Mathematical Society,
Vol. 378,
Issue. 3,
p.
1939.
沈, 文涛
2024.
The Lower Semicontinuity of Seminorms on Crossed Product <i>C</i><sup>*</sup>-Algebras of Integer Group.
Advances in Applied Mathematics,
Vol. 13,
Issue. 06,
p.
2943.
Kyed, David
and
Nest, Ryszard
2024.
Finiteness of metrics on state spaces.
Bulletin of the London Mathematical Society,
Vol. 56,
Issue. 1,
p.
288.
Long, Bo Tao
and
Wu, Wei
2024.
Almost Periodic Type Group Actions on Compact Quantum Metric Spaces.
Acta Mathematica Sinica, English Series,
Vol. 40,
Issue. 2,
p.
568.
Klisse, Mario
2024.
Crossed products as compact quantum metric spaces.
Canadian Journal of Mathematics,
p.
1.
AGUILAR, KONRAD
BEHERA, KARINA
OMLAND, TRON
and
WU, NICOLE
2025.
ON THE BURES METRIC,
$C^*$
-NORM AND QUANTUM METRIC.
Bulletin of the Australian Mathematical Society,
p.
1.
 $\ast$-automorphisms of a compact quantum metric space yields a field of crossed product algebras which varies continuously in Rieffel’s quantum Gromov–Hausdorff distance. Finally, we show how our results apply to continuous families of Lip-isometric actions on compact quantum metric spaces and to families of diffeomorphisms of compact Riemannian manifolds which vary continuously in the Whitney
$\ast$-automorphisms of a compact quantum metric space yields a field of crossed product algebras which varies continuously in Rieffel’s quantum Gromov–Hausdorff distance. Finally, we show how our results apply to continuous families of Lip-isometric actions on compact quantum metric spaces and to families of diffeomorphisms of compact Riemannian manifolds which vary continuously in the Whitney 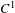 $C^{1}$-topology.
$C^{1}$-topology.