Hostname: page-component-cd9895bd7-gvvz8 Total loading time: 0 Render date: 2024-12-26T00:46:11.412Z Has data issue: false hasContentIssue false
  • English
  • Français

Dynamical degrees of affine-triangular automorphisms of affine spaces

Part of: Complex dynamical systems Affine geometry

Published online by Cambridge University Press:  01 October 2021

JÉRÉMY BLANC  and
IMMANUEL VAN SANTEN
JÉRÉMY BLANC*
Affiliation:
Departement Mathematik und Informatik, Universität Basel, Spiegelgasse 1, CH-4051Basel, Switzerland (e-mail: [email protected])
IMMANUEL VAN SANTEN
Affiliation:
Departement Mathematik und Informatik, Universität Basel, Spiegelgasse 1, CH-4051Basel, Switzerland (e-mail: [email protected])
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We study the possible dynamical degrees of automorphisms of the affine space $\mathbb {A}^n$ . In dimension 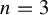$n=3$ , we determine all dynamical degrees arising from the composition of an affine automorphism with a triangular one. This generalizes the easier case of shift-like automorphisms which can be studied in any dimension. 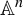We also prove that each weak Perron number is the dynamical degree of an affine-triangular automorphism of the affine space $\mathbb {A}^n$ for some n, and we give the best possible n for quadratic integers, which is either 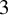$3$ or $4$ .

Keywords

dynamical degreespolynomial endomorphismsmonomial valuations

MSC classification

Primary: 37F10: Polynomials; rational maps; entire and meromorphic functions
Secondary: 14R10: Affine spaces (automorphisms, embeddings, exotic structures, cancellation problem)
Type
Original Article
Information
Ergodic Theory and Dynamical Systems , Volume 42 , Issue 12 , December 2022 , pp. 3551 - 3592
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bassino, F.. Nonnegative companion matrices and star-height of N-rational series. Theoret. Comput. Sci. 180(1–2) (1997), 61–80.CrossRefGoogle Scholar
Bonifant, A. M. and Fornæss, J. E.. Growth of degree for iterates of rational maps in several variables. Indiana Univ. Math. J. 49(2) (2000), 751–778.CrossRefGoogle Scholar
Bisi, C.. On commuting polynomial automorphisms of $\mathbb {C}^k, k\geq 3$ . Math. Z. 258(4) (2008), 875–891.CrossRefGoogle Scholar
Blanc, J.. Conjugacy classes of special automorphisms of the affine spaces. Algebra Number Theory 10(5) (2016), 939–967.CrossRefGoogle Scholar
Bedford, E. and Pambuccian, V.. Dynamics of shift-like polynomial diffeomorphisms of $\boldsymbol{\mathit{C}}^N$ . Conform. Geom. Dyn. 2 (1998), 45–55.CrossRefGoogle Scholar
Brunotte, H.. Algebraic properties of weak Perron numbers. Tatra Mt. Math. Publ. 56 (2013), 27–33.Google Scholar
Bera, S. and Verma, K.. Some aspects of shift-like automorphisms of $\mathbb {C}^k$ . Proc. Indian Acad. Sci. Math. Sci. 128(1) (2018), Art. 10, 48.Google Scholar
Blanc, J. and van Santen, I., Automorphisms of the affine $3$ -space of degree $3$ . Indiana Univ. Math. J., to appear.Google Scholar
Dang, N.-B. and Favre, C.. Spectral interpretations of dynamical degrees and applications. Ann. of Math. 194(1) (2021), 299–359.CrossRefGoogle Scholar
Déserti, J. and Leguil, M.. Dynamics of a family of polynomial automorphisms of ${\mathbb{C}}^3$ , a phase transition. J. Geom. Anal. 28(1) (2018), 190–224.CrossRefGoogle Scholar
Dinh, T.-C. and Nguyên, V.-A.. Comparison of dynamical degrees for semi-conjugate meromorphic maps. Comment. Math. Helv. 86(4) (2011), 817–840.CrossRefGoogle Scholar
Fekete, M.. Über die Verteilung der Wurzeln bei gewissen algebraischen Gleichungen mit ganzzahligen Koeffizienten. Math. Z. 17(1) (1923), 228–249.CrossRefGoogle Scholar
Favre, C. and Jonsson, M.. Eigenvaluations. Ann. Sci. École Norm. Sup. (4) 40(2) (2007), 309–349.CrossRefGoogle Scholar
Favre, C. and Jonsson, M.. Dynamical compactifications of $\boldsymbol{\mathit{C}}^3$ . Ann. of Math. (2) 173(1) (2011), 211–248.CrossRefGoogle Scholar
Fornæss, J. E. and Wu, H.. Classification of degree $2$ polynomial automorphisms of $\boldsymbol{\mathit{C}}^3$ . Publ. Mat. 42(1) (1998), 195–210.CrossRefGoogle Scholar
Furter, J.-P.. On the degree of iterates of automorphisms of the affine plane. Manuscripta Math. 98(2) (1999), 183–193.CrossRefGoogle Scholar
Favre, C. and Wulcan, E.. Degree growth of monomial maps and McMullen’s polytope algebra. Indiana Univ. Math. J. 61(2) (2012), 493–524.CrossRefGoogle Scholar
Gantmacher, F. R.. The Theory of Matrices. Vols. 1 and 2, translated by K. A. Hirsch. Chelsea Publishing Co., New York, 1959.Google Scholar
Guedj, V. and Sibony, N.. Dynamics of polynomial automorphisms of $\boldsymbol{\mathit{C}}^k$ . Ark. Mat. 40(2) (2002), 207–243.Google Scholar
Guedj, V.. Dynamics of polynomial mappings of $\mathbb {C}^2$ . Amer. J. Math. 124(1) (2002), 75–106.CrossRefGoogle Scholar
Guedj, V.. Dynamics of quadratic polynomial mappings of $\mathbb {C}^2$ . Michigan Math. J. 52(3) (2004), 627–648.CrossRefGoogle Scholar
Jung, H. W. E.. Über ganze birationale transformationen der Ebene. J. Reine Angew. Math. 184 (1942), 161–174.CrossRefGoogle Scholar
Jonsson, M. and Wulcan, E.. Canonical heights for plane polynomial maps of small topological degree. Math. Res. Lett. 19(6) (2012), 1207–1217.CrossRefGoogle Scholar
Lind, D. A.. The entropies of topological Markov shifts and a related class of algebraic integers. Ergod. Th. & Dynam. Syst. 4(2) (1984), 283–300.CrossRefGoogle Scholar
Lin, J.-L.. Pulling back cohomology classes and dynamical degrees of monomial maps. Bull. Soc. Math. France 140(4) (2012), 533–549.CrossRefGoogle Scholar
Maegawa, K.. Three dimensional shift-like mappings of dynamical degree golden ratio. Proceedings of the Second ISAAC Congress, Vol. 2 (Fukuoka, 1999) (International Society for Analysis, Applications and Computation, 8). Kluwer Academic Publishers, Dordrecht, 2000, pp. 1057–1062.CrossRefGoogle Scholar
Maegawa, K.. Classification of quadratic polynomial automorphisms of ${\mathbb{C}}^3$ from a dynamical point of view. Indiana Univ. Math. J. 50(2) (2001), 935–951.CrossRefGoogle Scholar
Maegawa, K.. Quadratic polynomial automorphisms of dynamical degree golden ratio of $\mathbb {C}^3$ . Ergod. Th. & Dynam. Syst. 21(3) (2001), 823–832.CrossRefGoogle Scholar
Matsumura, H.. Commutative Ring Theory (Cambridge Studies in Advanced Mathematics, 8), 2nd edn. Translated from the Japanese by M. Reid. Cambridge University Press, Cambridge, 1989.Google Scholar
Meisters, G. H. and Olech, C.. Strong nilpotence holds in dimensions up to five only. Linear Multilinear Algebra 30(4) (1991), 231–255.Google Scholar
Ostrowski, A. M.. Solution of Equations in Euclidean and Banach Spaces, 3rd edn of Solution of Equations and Systems of Equations (Pure and Applied Mathematics, 9). Academic Press [A Subsidiary of Harcourt Brace Jovanovich, Publishers], New York, 1973.Google Scholar
Schinzel, A.. A class of algebraic numbers. Tatra Mt. Math. Publ. 11 (1997), 35–42.Google Scholar
Sibony, N.. Dynamique des applications rationnelles de $\boldsymbol{\mathit{P}}^k$ . Dynamique et géométrie complexes (Lyon, 1997) (Panoramas et Synthèses, 8). Société mathématique de France, Paris, 1999, pp. ix–x, xi–xii, 97–185.Google Scholar
Michael Steele, J.. Probability Theory and Combinatorial Optimization (CBMS-NSF Regional Conference Series in Applied Mathematics, 69). Society for Industrial and Applied Mathematics (SIAM), Philadelphia, 1997.CrossRefGoogle Scholar
Struik, D. J.. A Source Book in Mathematics, 1200–1800. Princeton Paperbacks, Princeton University Press, Princeton, NJ, 1986 (in English) (Reprint of the 1969 edn).CrossRefGoogle Scholar
Sun, X.. Classification of quadratic homogeneous automorphisms in dimension five. Comm. Algebra 42(7) (2014), 2821–2840.CrossRefGoogle Scholar
Ueda, T.. Fixed points of polynomial automorphisms of $\boldsymbol{\mathit{C}}^n$ . Complex Analysis in Several Variables—Memorial Conference of Kiyoshi Oka’s Centennial Birthday (Advanced Studies in Pure Mathematics, 42), Mathematical Society of Japan, Tokyo, 2004, pp. 319–324.Google Scholar
van den Essen, A.. Polynomial Automorphisms and the Jacobian Conjecture (Progress in Mathematics, 190). Birkhäuser Verlag, Basel, 2000.Google Scholar
van der Kulk, W.. On polynomial rings in two variables. Nieuw Arch. Wiskunde (3) 1 (1953), 33–41.Google Scholar
Xie, J.. The dynamical Mordell-Lang conjecture for polynomial endomorphisms of the affine plane. Astérisque 394 (2017), vi+110.Google Scholar