Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-26T00:44:43.934Z Has data issue: false hasContentIssue false
  • English
  • Français

Discontinuity of Lyapunov exponents near fiber bunched cocycles

Published online by Cambridge University Press:  19 September 2016

CLARK BUTLER
CLARK BUTLER*
Affiliation:
Department of Mathematics, The University of Chicago, 5734 S University Ave, Chicago, IL 60637, USA email [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We give examples of locally constant 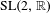$\text{SL}(2,\mathbb{R})$-cocycles over a Bernoulli shift that are discontinuity points for Lyapunov exponents in the Hölder topology and are arbitrarily close to satisfying the fiber bunching inequality. Backes, Brown, and the author [Continuity of Lyapunov exponents for cocycles with invariant holonomies. Preprint, 2015, arXiv:1507.08978] have shown that the Lyapunov exponents vary continuously when restricted to the space of fiber bunched Hölder continuous cocycles. Our examples give evidence that this theorem is optimal within certain families of Hölder cocycles.

Type
Original Article
Information
Ergodic Theory and Dynamical Systems , Volume 38 , Issue 2 , April 2018 , pp. 523 - 539
Copyright
© Cambridge University Press, 2016 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abadi, M. and Vergne, N.. Sharp error terms for return time statistics under mixing conditions. J. Theoret. Probab. 22(1) (2009), 18–37.Google Scholar
Backes, L., Brown, A. and Butler, C.. Continuity of Lyapunov exponents for cocycles with invariant holonomies. Preprint, 2015, arXiv:1507.08978.Google Scholar
Bochi, J.. Genericity of zero Lyapunov exponents. Ergod. Th. & Dynam. Sys. 22 (2002), 1667–1696.Google Scholar
Bocker-Neto, C. and Viana, M.. Continuity of Lyapunov exponents for random 2d matrices. Ergod. Th. & Dynam. Sys., to appear, Preprint, 2010, arXiv:1012.0872.Google Scholar
Tao, T.. Topics in Random Matrix Theory (Graduate Studies in Mathematics, 132) . American Mathematical Society, Providence, RI, 2012.Google Scholar
Viana, M.. Almost all cocycles over any hyperbolic system have nonvanishing Lyapunov exponents. Ann. of Math. (2) 167(2) (2008), 643–680.Google Scholar
Viana, M.. Lectures on Lyapunov Exponents (Cambridge Studies in Advanced Mathematics, 145) . Cambridge University Press, Cambridge, 2014.CrossRefGoogle Scholar