Published online by Cambridge University Press: 21 July 2015
The dynamical degrees of a rational map 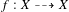 $f:X{\dashrightarrow}X$ are fundamental invariants describing the rate of growth of the action of iterates of
$f:X{\dashrightarrow}X$ are fundamental invariants describing the rate of growth of the action of iterates of 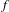 $f$ on the cohomology of
$f$ on the cohomology of 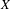 $X$ . When
$X$ . When 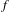 $f$ has non-empty indeterminacy set, these quantities can be very difficult to determine. We study rational maps
$f$ has non-empty indeterminacy set, these quantities can be very difficult to determine. We study rational maps 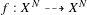 $f:X^{N}{\dashrightarrow}X^{N}$ , where
$f:X^{N}{\dashrightarrow}X^{N}$ , where 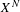 $X^{N}$ is isomorphic to the Deligne–Mumford compactification
$X^{N}$ is isomorphic to the Deligne–Mumford compactification  $\overline{{\mathcal{M}}}_{0,N+3}$ . We exploit the stratified structure of
$\overline{{\mathcal{M}}}_{0,N+3}$ . We exploit the stratified structure of  $X^{N}$ to provide new examples of rational maps, in arbitrary dimension, for which the action on cohomology behaves functorially under iteration. From this, all dynamical degrees can be readily computed (given enough book-keeping and computing time). In this paper, we explicitly compute all of the dynamical degrees for all such maps
$X^{N}$ to provide new examples of rational maps, in arbitrary dimension, for which the action on cohomology behaves functorially under iteration. From this, all dynamical degrees can be readily computed (given enough book-keeping and computing time). In this paper, we explicitly compute all of the dynamical degrees for all such maps 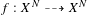 $f:X^{N}{\dashrightarrow}X^{N}$ , where
$f:X^{N}{\dashrightarrow}X^{N}$ , where 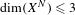 $\text{dim}(X^{N})\leq 3$ and the first dynamical degrees for the mappings where
$\text{dim}(X^{N})\leq 3$ and the first dynamical degrees for the mappings where 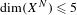 $\text{dim}(X^{N})\leq 5$ . These examples naturally arise in the setting of Thurston’s topological characterization of rational maps.
$\text{dim}(X^{N})\leq 5$ . These examples naturally arise in the setting of Thurston’s topological characterization of rational maps.