Hostname: page-component-586b7cd67f-gb8f7 Total loading time: 0 Render date: 2024-11-24T10:39:48.811Z Has data issue: false hasContentIssue false
  • English
  • Français

Closest integer polynomial multiple recurrence along shifted primes

Published online by Cambridge University Press:  20 September 2016

ANDREAS KOUTSOGIANNIS
ANDREAS KOUTSOGIANNIS*
Affiliation:
The Ohio State University, Department of Mathematics, 100 Math Tower, 231 West 18th Avenue, Columbus, OH 43210-1174, USA email [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Following an approach presented by Frantzikinakis et al [The polynomial multidimensional Szemerédi theorem along shifted primes. Israel J. Math.194(1) (2013), 331–348], we show that the parameters in the multidimensional Szemerédi theorem for closest integer polynomials have non-empty intersection with the set of shifted primes 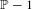$\mathbb{P}-1$ (or, similarly, of 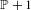$\mathbb{P}+1$). Using the Furstenberg correspondence principle, we show this result by recasting it as a polynomial multiple recurrence result in measure ergodic theory. Furthermore, we obtain integer part polynomial convergence results by the same method, which is a transference principle that enables one to deduce results for $\mathbb{Z}$-actions from results for flows. We also give some applications of our approach to Gowers uniform sets.

Type
Original Article
Information
Ergodic Theory and Dynamical Systems , Volume 38 , Issue 2 , April 2018 , pp. 666 - 685
Copyright
© Cambridge University Press, 2016 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bergelson, V.. Weakly mixing PET. Ergod. Th. & Dynam. Sys. 7(3) (1987), 337–349.CrossRefGoogle Scholar
Bergelson, V. and Håland, I. J.. Sets of recurrence and generalized polynomials. Convergence in Ergodic Theory and Probability (Columbus, OH, 1993) (Ohio State University Mathematical Research Institute Publications) . de Gruyter, Berlin, 1996, vol. 5, pp. 91–110.CrossRefGoogle Scholar
Bergelson, V., Host, B., McCutcheon, R. and Parreau, F.. Aspects of uniformity in recurrence. Colloq. Math. 84/85(2) (2000), 549–576.CrossRefGoogle Scholar
Bergelson, V. and Leibman, A.. Polynomial extensions of van der Waerden’s and Szemerédi’s theorems. J. Amer. Math. Soc. 9 (1996), 725–753.CrossRefGoogle Scholar
Bergelson, V., Leibman, A. and Ziegler, T.. The shifted primes and the multidimensional Szemerédi and polynomial van der Waerden theorems. C. R. Math. Acad. Sci. Paris. 349(3–4) (2011), 123–125.CrossRefGoogle Scholar
Boshernitzan, M., Jones, R. L. and Wierdl, M.. Integer and fractional parts of good averaging sequences in ergodic theory. Convergence in Ergodic Theory and Probability. Eds. Bergelson, V., March, P. and Rosenblatt, J.. de Gruyter, Berlin, 1996, pp. 117–132.CrossRefGoogle Scholar
Frantzikinakis, N.. Multiple correlation sequences and nilsequences. Invent. Math. 202(2) (2015), 875–892.CrossRefGoogle Scholar
Frantzikinakis, N. and Host, B.. Multiple ergodic theorems for arithmetic sets. Trans. Amer. Math. Soc., to appear.Google Scholar
Frantzikinakis, N., Host, B. and Kra, B.. Multiple recurrence and convergence for sequences related to the prime numbers. J. Reine Angew. Math. 611 (2007), 131–144.Google Scholar
Frantzikinakis, N., Host, B. and Kra, B.. The polynomial multidimensional Szemerédi theorem along shifted primes. Israel J. Math. 194(1) (2013), 331–348.CrossRefGoogle Scholar
Furstenberg, H.. Ergodic behaviour of diagonal measures and a theorem of Szemerédi on arithmetic progressions. J. Anal. Math. 71 (1977), 204–256.CrossRefGoogle Scholar
Gowers, W.. A new proof of Szemerédi’s theorem. Geom. Funct. Anal. 11 (2001), 465–588.CrossRefGoogle Scholar
Green, B. and Tao, T.. Linear equations in primes. Ann. of Math. (2) 171 (2010), 1753–1850.CrossRefGoogle Scholar
Green, B. and Tao, T.. The Möbius function is strongly orthogonal to nilsequences. Ann. of Math. (2) 175 (2012), 541–566.CrossRefGoogle Scholar
Green, B., Tao, T. and Ziegler, T.. An inverse theorem for the Gowers U s+1[N]-norm. Ann. of Math. (2) 176(2) (2012), 1231–1372.CrossRefGoogle Scholar
Koutsogiannis, A.. Integer part correlation sequences. Preprint, 2015, arXiv:1512.01313.Google Scholar
Lesigne, E.. On the sequence of integer parts of a good sequence for the ergodic theorem. Comment. Math. Univ. Carolin. 36(4) (1995), 737–743.Google Scholar
Sárközy, A.. On difference sets of sequences of integers, III. Acta Math. Acad. Sci. Hungar. 31 (1978), 355–386.CrossRefGoogle Scholar
Sun, W.. Multiple recurrence and convergence for certain averages along shifted primes. Ergod. Th. & Dynam. Sys. 35 (2014), 1592–1609.CrossRefGoogle Scholar
Walsh, M.. Norm convergence of nilpotent ergodic averages. Ann. of Math. (2) 175(3) (2012), 1667–1688.CrossRefGoogle Scholar
Wierdl, M.. Personal communication, 2015.Google Scholar
Wooley, T. and Ziegler, T.. Multiple recurrence and convergence along the primes. Amer. J. Math. 134(6) (2012), 1705–1732.CrossRefGoogle Scholar