Hostname: page-component-586b7cd67f-2plfb Total loading time: 0 Render date: 2024-11-24T11:13:54.026Z Has data issue: false hasContentIssue false
  • English
  • Français

The boundary of hyperbolicity for Hénon-like families

Published online by Cambridge University Press:  01 August 2008

YONGLUO CAO ,
STEFANO LUZZATTO  and
ISABEL RIOS
YONGLUO CAO
Affiliation:
Department of Mathematics, Suzhou University, 215006, Jiangsu, PR China Institute of Mathematics, Fudan University, Shanghai, 200433, PR China (email: [email protected], [email protected])
STEFANO LUZZATTO
Affiliation:
Department of Mathematics, Imperial College, 180 Queen’s Gate, London SW7 2AZ, UK (email: [email protected])
ISABEL RIOS
Affiliation:
Universidade Federal Fluminense, Niteroi, RJ, Brazil (email: [email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

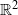We consider C2 Hénon-like families of diffeomorphisms of and study the boundary of the region of parameter values for which the non-wandering set is uniformly hyperbolic. Assuming sufficient dissipativity, we show that the loss of hyperbolicity is caused by a first homoclinic or heteroclinic tangency and that uniform hyperbolicity estimates hold uniformly in the parameter up to the bifurcation parameter and even, to some extent, at the bifurcation parameter.

Type
Research Article
Information
Ergodic Theory and Dynamical Systems , Volume 28 , Issue 4 , August 2008 , pp. 1049 - 1080
Copyright
Copyright © 2008 Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

[1]Bedford, E.. Personal communication, 2005.Google Scholar
[2]Bedford, E. and Smillie, J.. Polynomial diffeomorphisms of C2. VIII. Quasi-expansion. Amer. J. Math. 124(2) (2002), 221–271.CrossRefGoogle Scholar
[3]Bedford, E. and Smillie, J.. Real polynomial diffeomorphisms with maximal entropy: Tangencies. Ann. Math. 160 (2004), 1–26.CrossRefGoogle Scholar
[4]Bedford, E. and Smillie, J.. Real polynomial diffeomorphisms with maximal entropy: II. Small Jacobian. Ergod. Th. & Dynam. Sys. 26(5) (2006), 1259–1283.CrossRefGoogle Scholar
[5]Benedicks, M. and Carleson, L.. The dynamics of the Heńon map. Ann. of Math. 133 (1991), 73–169.CrossRefGoogle Scholar
[6]Benedicks, M. and Viana, M.. Solution of the basin problem for Hénon-like attractors. Invent. Math. 143(2) (2001), 375–434.CrossRefGoogle Scholar
[7]Benedicks, M. and Young, L.-S.. Markov extensions and decay of correlations for certain Hénon maps. Astérisque (261) (2000), xi, 13–56 (English, with English and French summaries). Géométrie complexe et systèmes dynamiques (Orsay, 1995) 0303-1179..Google Scholar
[8]Benedicks, M. and Young, L.-S.. Sinaĭ–Bowen–Ruelle measures for certain Hénon maps. Invent. Math. 112(3) (1993), 541–576.CrossRefGoogle Scholar
[9]Cao, Y., Luzzatto, S. and Rios, I.. A non-hyperbolic system with strictly non-zero Lyapunov exponents for all invariant measures. Disc. & Cont. Dyn. Syst. 15(1) (2006), 61–71.CrossRefGoogle Scholar
[10]Devaney, R. and Nitecki, Z.. Shift automorphisms in the Hénon mapping. Comm. Math. Phys. 67(2) (1979), 137–146.CrossRefGoogle Scholar
[11]Hénon, M.. A two-dimensional mapping with a strange attractor. Comm. Math. Phys. 50 (1976), 69–77.CrossRefGoogle Scholar
[12]Holland, M. and Luzzatto, S.. Stable manifolds under very weak hyperbolicity conditions. J. Differential Equations 221(2) (2006), 444–469.CrossRefGoogle Scholar
[13]Luzzatto, S. and Viana, M.. Parameter exclusions in Hénon-like systems. Russian Math. Surveys 58(6) (2003), 1053–1092.CrossRefGoogle Scholar
[14]de Melo, W. and van Strien, S.. One-Dimensional Dynamics, Vol. 25. Springer, Berlin, 1993.CrossRefGoogle Scholar
[15]Mora, L. and Viana, M.. Abundance of strange attractors. Acta Math. 171(1) (1993), 1–71.CrossRefGoogle Scholar
[16]Oseledec, V. I.. A multiplicative ergodic theorem. Characteristic Ljapunov exponents of dynamical systems. Trans. Moscow Math. Soc. 19 (1968), 179–210.Google Scholar
[17]Palis, J. and de Melo, W.. Geometric Theory of Dynamical Systems. An Introduction. Springer, New York, 1982.CrossRefGoogle Scholar
[18]Pesin, Ya.. Families of invariant manifolds corresponding to non-zero characteristic exponents. Math. USSR. Izv. 10 (1976), 1261–1302.CrossRefGoogle Scholar
[19]Pesin, Ya. B.. Characteristic Lyapunov exponents and smooth ergodic theory. Russian Math. Surveys 324 (1977), 55–114.CrossRefGoogle Scholar
[20]Wang, Q. and Young, L.-S.. Strange attractors with one direction of instability. Comm. Math. Phys. 218(1) (2001), 1–97.CrossRefGoogle Scholar