Hostname: page-component-586b7cd67f-2plfb Total loading time: 0 Render date: 2024-11-24T09:45:23.687Z Has data issue: false hasContentIssue false
  • English
  • Français

Attractive regular stochastic chains: perfect simulation and phase transition

Published online by Cambridge University Press:  03 April 2013

SANDRO GALLO  and
DANIEL Y. TAKAHASHI
SANDRO GALLO
Affiliation:
Departamento de Métodos Estatísticos, Instituto de Matemática, Universidade Federal de Rio de Janeiro, Caixa Postal 68350, CEP 21941-909, Rio de Janeiro, Brasil email [email protected]
DANIEL Y. TAKAHASHI
Affiliation:
Neuroscience Institute and Psychology Department, Princeton University, Greenhall, Princeton, NJ 08540, USA email [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We prove that uniqueness of the stationary chain, or equivalently, of the 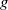$g$-measure, compatible with an attractive regular probability kernel is equivalent to either one of the following two assertions for this chain: (1) it is a finitary coding of an independent and identically distributed (i.i.d.) process with countable alphabet; (2) the concentration of measure holds at exponential rate. We show in particular that if a stationary chain is uniquely defined by a kernel that is continuous and attractive, then this chain can be sampled using a coupling-from-the-past algorithm. For the original Bramson–Kalikow model we further prove that there exists a unique compatible chain if and only if the chain is a finitary coding of a finite alphabet i.i.d. process. Finally, we obtain some partial results on conditions for phase transition for general chains of infinite order.

Type
Research Article
Information
Ergodic Theory and Dynamical Systems , Volume 34 , Issue 5 , October 2014 , pp. 1567 - 1586
Copyright
Copyright ©2013 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Berger, N., Hoffman, C. and Sidoravicius, V.. Nonuniqueness for specifications in ${\ell }^{2+ \epsilon } $. Preprint, 2005, arXiv:math/0312344.Google Scholar
Bramson, M. and Kalikow, S.. Nonuniqueness in $g$-functions. Israel J. Math. 84 (1–2) (1993), 153–160.Google Scholar
Cénac, P., Chauvin, B., Paccaut, F. and Pouyanne, N.. Variable length Markov chains and dynamical sources. Séminaire de Probabilités XLIV (Lecture Notes in Mathematics, 2046). Eds. Donati-Martin, C., Lejay, A. and Rouault, A.. Springer, Berlin, 2012, pp. 1–39.Google Scholar
Chazottes, J.-R., Collet, P., Külske, C. and Redig, F.. Concentration inequalities for random fields via coupling. Probab. Theory Related Fields 137 (1–2) (2007), 201–225.Google Scholar
Comets, F., Fernández, R. and Ferrari, P. A.. Processes with long memory: regenerative construction and perfect simulation. Ann. Appl. Probab. 12 (3) (2002), 921–943.Google Scholar
De Santis, E. and Piccioni, M.. Backward coalescence times for perfect simulation of chains with infinite memory. J. Appl. Probab. 49 (2) (2012), 319–337.Google Scholar
Doeblin, W. and Fortet, R.. Sur des chaînes à liaisons complètes. Bull. Soc. Math. France 65 (1937), 132–148.Google Scholar
Fernández, R., Gallo, S. and Maillard, G.. Regular $g$-measures are not always Gibbsian. Electron. Commun. Probab. 16 (2011), 732–740.Google Scholar
Fernández, R. and Maillard, G.. Chains with complete connections: general theory, uniqueness, loss of memory and mixing properties. J. Stat. Phys. 118 (3–4) (2005), 555–588.Google Scholar
Friedli, S.. A note on the Bramson–Kalikow process. Unpublished, 2010, http://www.mat.ufmg.br/~sacha/textos/BK/plateaux.pdf.Google Scholar
Gallo, S.. Chains with unbounded variable length memory: perfect simulation and a visible regeneration scheme. Adv. in Appl. Probab. 43 (3) (2011), 735–759.Google Scholar
Gallo, S. and Garcia, N. L.. Perfect simulation for locally continuous chains of infinite order. Preprint, 2011, arXiv:1103.2058v4.Google Scholar
Harris, T. E.. On chains of infinite order. Pacific J. Math. 5 (1955), 707–724.Google Scholar
Hulse, P.. Uniqueness and ergodic properties of attractive $g$-measures. Ergod. Th. & Dynam. Sys. 11 (1) (1991), 65–77.Google Scholar
Hulse, P.. An example of non-unique $g$-measures. Ergod. Th. & Dynam. Sys. 26 (2) (2006), 439–445.Google Scholar
Johansson, A. and Öberg, A.. Square summability of variations of $g$-functions and uniqueness of $g$-measures. Math. Res. Lett. 10 (5–6) (2003), 587–601.Google Scholar
Kalikow, S.. Random Markov processes and uniform martingales. Israel J. Math. 71 (1) (1990), 33–54.Google Scholar
Karlin, S.. Some random walks arising in learning models. I. Pacific J. Math. 3 (1953), 725–756.Google Scholar
Keane, M.. Strongly mixing $g$-measures. Invent. Math. 16 (1972), 309–324.Google Scholar
Lacroix, Y.. A note on weak-$\star $ perturbations of $g$-measures. Sankhyā A 62 (3) (2000), 331–338.Google Scholar
Marton, K. and Shields, P. C.. The positive-divergence and blowing-up properties. Israel J. Math. 86 (1–3) (1994), 331–348.Google Scholar
McCullagh, P. and Nelder, J. A.. Generalized Linear Models (Monographs on Statistics and Applied Probability). Chapman & Hall, London, 1983.Google Scholar
Onicescu, O. and Mihoc, G.. Sur les chaînes de variables statistiques. Bull. Sci. Math. 59 (2) (1935), 174–192.Google Scholar
Preston, C.. Random Fields (Lecture Notes in Mathematics, 534). Springer, Berlin, 1976.Google Scholar
Propp, J. G. and Wilson, D. B.. Exact sampling with coupled Markov chains and applications to statistical mechanics. Proceedings of the Seventh International Conference on Random Structures and Algorithms (Atlanta, GA, 1995). Vol. 9. Eds. Karoński, M., Spencer, J. and Ruciński, A.. Wiley, New York, 1996.Google Scholar
Quas, A. N.. Non-ergodicity for ${C}^{1} $ expanding maps and $g$-measures. Ergod. Th. & Dynam. Sys. 16 (3) (1996), 531–543.Google Scholar
Rudolph, D. J.. A mixing Markov chain with exponentially decaying return times is finitarily Bernoulli. Ergod. Th. & Dynam. Sys. 2 (1) (1982), 85–97.Google Scholar
Shields, P. C.. The Ergodic Theory of Discrete Sample Paths (Graduate Studies in Mathematics, 13). American Mathematical Society, Providence, RI, 1996.Google Scholar
Steif, J. and van den Berg, J.. On the existence and nonexistence of finitary codings for a class of random fields. Ann. Probab. 11 (1999), 1501–1522.Google Scholar
Stenflo, Ö.. A note on a theorem of Karlin. Statist. Probab. Lett. 54 (2) (2001), 183–187.Google Scholar