Article contents
Traces on topological-graph algebras
Published online by Cambridge University Press: 02 May 2017
Abstract
Given a topological graph 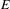 $E$, we give a complete description of tracial states on the
$E$, we give a complete description of tracial states on the 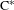 $\text{C}^{\ast }$-algebra
$\text{C}^{\ast }$-algebra  $\text{C}^{\ast }(E)$ which are invariant under the gauge action; there is an affine homeomorphism between the space of gauge invariant tracial states on
$\text{C}^{\ast }(E)$ which are invariant under the gauge action; there is an affine homeomorphism between the space of gauge invariant tracial states on 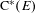 $\text{C}^{\ast }(E)$ and Radon probability measures on the vertex space
$\text{C}^{\ast }(E)$ and Radon probability measures on the vertex space  $E^{0}$ which are, in a suitable sense, invariant under the action of the edge space
$E^{0}$ which are, in a suitable sense, invariant under the action of the edge space 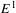 $E^{1}$. It is shown that if
$E^{1}$. It is shown that if  $E$ has no cycles, then every tracial state on
$E$ has no cycles, then every tracial state on 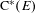 $\text{C}^{\ast }(E)$ is gauge invariant. When
$\text{C}^{\ast }(E)$ is gauge invariant. When 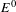 $E^{0}$ is totally disconnected, the gauge invariant tracial states on
$E^{0}$ is totally disconnected, the gauge invariant tracial states on 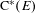 $\text{C}^{\ast }(E)$ are in bijection with the states on
$\text{C}^{\ast }(E)$ are in bijection with the states on 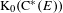 $\text{K}_{0}(\text{C}^{\ast }(E))$.
$\text{K}_{0}(\text{C}^{\ast }(E))$.
- Type
- Original Article
- Information
- Copyright
- © Cambridge University Press, 2017
References
- 1
- Cited by


