Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Kokubu, Hiroshi
and
Naudot, Vincent
1997.
The existence of infinitely many homoclinic doubling bifurcations from some codimension 3 homoclinic orbits.
Journal of Dynamics and Differential Equations,
Vol. 9,
Issue. 3,
p.
445.
Sterling, D.
Dullin, H.R.
and
Meiss, J.D.
1999.
Homoclinic bifurcations for the Hénon map.
Physica D: Nonlinear Phenomena,
Vol. 134,
Issue. 2,
p.
153.
Homburg, Ale Jan
2000.
Numerical Methods for Bifurcation Problems and Large-Scale Dynamical Systems.
Vol. 119,
Issue. ,
p.
185.
Homburg, Ale Jan
2000.
Singular Heteroclinic Cycles.
Journal of Differential Equations,
Vol. 161,
Issue. 2,
p.
358.
Homburg, Ale Jan
2001.
Ergodic Theory, Analysis, and Efficient Simulation of Dynamical Systems.
p.
271.
Naudot, Vincent
2002.
A strange attractor in the unfolding of an orbit-flip homoclinic orbit.
Dynamical Systems,
Vol. 17,
Issue. 1,
p.
45.
Morales, C. A.
Pacifico, M. J.
and
Martin, B. San
2005.
Expanding Lorenz Attractors through Resonant Double Homoclinic Loops.
SIAM Journal on Mathematical Analysis,
Vol. 36,
Issue. 6,
p.
1836.
Martens, Marco
Naudot, Vincent
and
Yang, Jiazhong
2005.
A cubic Hénon-like map in the unfolding of degenerate homoclinic orbit with resonance.
Comptes Rendus. Mathématique,
Vol. 340,
Issue. 11,
p.
843.
MARTENS, M.
NAUDOT, V.
and
YANG, J.
2006.
A STRANGE ATTRACTOR WITH LARGE ENTROPY IN THE UNFOLDING OF A LOW RESONANT DEGENERATE HOMOCLINIC ORBIT.
International Journal of Bifurcation and Chaos,
Vol. 16,
Issue. 12,
p.
3509.
Homburg, Ale Jan
2006.
Invariant manifolds near hyperbolic fixed points.
Journal of Difference Equations and Applications,
Vol. 12,
Issue. 10,
p.
1057.
Lu, Qiuying
2009.
Non-resonance 3D homoclinic bifurcation with an inclination flip.
Chaos, Solitons & Fractals,
Vol. 42,
Issue. 5,
p.
2597.
Lu, Qiuying
2009.
Codimension 2 bifurcation of twisted double homoclinic loops.
Computers & Mathematics with Applications,
Vol. 57,
Issue. 7,
p.
1127.
Homburg, Ale Jan
and
Sandstede, Björn
2010.
Vol. 3,
Issue. ,
p.
379.
Naudot, Vincent
and
Yang, Jiazhong
2010.
Finite smooth normal forms and integrability of local
families of vector fields.
Discrete & Continuous Dynamical Systems - S,
Vol. 3,
Issue. 4,
p.
667.
Naudot, Vincent
and
Yang, Jiazhong
2013.
Quasi-linearization of parameter-depending germs of vector fields.
Dynamical Systems,
Vol. 28,
Issue. 2,
p.
173.
Aguirre, Pablo
Krauskopf, Bernd
and
Osinga, Hinke M.
2013.
Global Invariant Manifolds Near Homoclinic Orbits to a Real Saddle: (Non)Orientability and Flip Bifurcation.
SIAM Journal on Applied Dynamical Systems,
Vol. 12,
Issue. 4,
p.
1803.
Zhang, Tiansi
Huang, Xiaoxin
and
Zhu, Deming
2013.
Resonant Homoclinic Flips Bifurcation in Principal Eigendirections.
Abstract and Applied Analysis,
Vol. 2013,
Issue. ,
p.
1.
Fontaine, Marcus
Kalies, William
and
Naudot, Vincent
2016.
Chaos near a resonant inclination-flip.
Physica D: Nonlinear Phenomena,
Vol. 334,
Issue. ,
p.
141.
Lu, Qiuying
and
Naudot, Vincent
2016.
Bifurcation Complexity from Orbit-Flip Homoclinic Orbit of Weak Type.
International Journal of Bifurcation and Chaos,
Vol. 26,
Issue. 04,
p.
1650059.
Giraldo, Andrus
Krauskopf, Bernd
and
Osinga, Hinke M.
2017.
Saddle Invariant Objects and Their Global Manifolds in a Neighborhood of a Homoclinic Flip Bifurcation of Case B.
SIAM Journal on Applied Dynamical Systems,
Vol. 16,
Issue. 1,
p.
640.
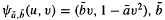 being close to 0.
being close to 0.

