Hostname: page-component-669899f699-8p65j Total loading time: 0 Render date: 2025-04-24T22:12:32.239Z Has data issue: false hasContentIssue false
  • English
  • Français

Shrinking parallelepiped targets for $\beta $-dynamical systems

Part of: Classical measure theory Probabilistic theory: distribution modulo $1$; metric theory of algorithms

Published online by Cambridge University Press:  19 November 2024

YUBIN HE
YUBIN HE*
Affiliation:
Department of Mathematics, Shantou University, Shantou, Guangdong 515063, China
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

For 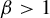$ \beta>1 $, let 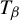$ T_\beta $ be the 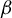$\beta $-transformation on 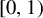$ [0,1) $. Let $ \beta _1,\ldots ,\beta _d>1 $ and let 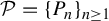$ \mathcal P=\{P_n\}_{n\ge 1} $ be a sequence of parallelepipeds in 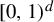$ [0,1)^d $. Define

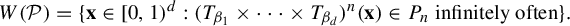$$ \begin{align*}W(\mathcal P)=\{\mathbf{x}\in[0,1)^d:(T_{\beta_1}\times\cdots \times T_{\beta_d})^n(\mathbf{x})\in P_n\text{ infinitely often}\}.\end{align*} $$
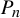When each $ P_n $ is a hyperrectangle with sides parallel to the axes, the ‘rectangle to rectangle’ mass transference principle by Wang and Wu [Mass transference principle from rectangles to rectangles in Diophantine approximation. Math. Ann. 381 (2021) 243–317] is usually employed to derive the lower bound for 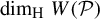$\dim _{\mathrm {H}} W(\mathcal P)$, where 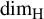$\dim _{\mathrm {H}}$ denotes the Hausdorff dimension. However, in the case where 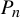$ P_n $ is still a hyperrectangle but with rotation, this principle, while still applicable, often fails to yield the desired lower bound. In this paper, we determine the optimal cover of parallelepipeds, thereby obtaining 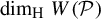$\dim _{\mathrm {H}} W(\mathcal P)$. We also provide several examples to illustrate how the rotations of hyperrectangles affect $\dim _{\mathrm {H}} W(\mathcal P)$.

Keywords

β-expansionHausdorff dimensionparallelepipedshrinking target problem

MSC classification

Primary: 11K55: Metric theory of other algorithms and expansions; measure and Hausdorff dimension
Secondary: 28A80: Fractals
Type
Original Article
Information
Ergodic Theory and Dynamical Systems , First View , pp. 1 - 16
Check for updates
Copyright
© The Author(s), 2024. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Allen, D. and Bárány, B.. On the Hausdorff measure of shrinking target sets on self-conformal sets. Mathematika 67 (2021), 807–839.CrossRefGoogle Scholar
Bárány, B. and Rams, M.. Shrinking targets on Bedford–McMullen carpets. Proc. Lond. Math. Soc. (3) 117 (2018), 951–995.CrossRefGoogle Scholar
Bishop, C. J. and Peres, Y.. Fractals in Probability and Analysis. Cambridge University Press, Cambridge, 2017.Google Scholar
Bugeaud, Y. and Wang, B.. Distribution of full cylinders and the Diophantine properties of the orbits in $\beta$ -expansions. J. Fractal Geom. 1(2) (2014), 221–241.CrossRefGoogle Scholar
Falconer, K.. The Hausdorff dimension of self-affine fractals. Math. Proc. Cambridge Philos. Soc. 103(2) (1988), 339–350.CrossRefGoogle Scholar
Falconer, K.. Sets with large intersection properties. J. Lond. Math. Soc. (2) 49(2) (1994), 267–280.CrossRefGoogle Scholar
Fan, A. and Wang, B.. On the lengths of basic intervals in beta expansions. Nonlinearity 25(5) (2012), 1329–1343.CrossRefGoogle Scholar
He, Y.. Path-dependent shrinking target problems in beta-dynamical systems. Nonlinearity 36 (2023), 6991–7006.CrossRefGoogle Scholar
He, Y.. A unified approach to mass transference principle and large intersection property. Preprint, 2024, arXiv:2402.00513.Google Scholar
Hill, R. and Velani, S.. Metric Diophantine approximation in Julia sets of expanding rational maps. Publ. Math. Inst. Hautes Études Sci. 85 (1997), 193–216.CrossRefGoogle Scholar
Hill, R. and Velani, S.. The shrinking target problem for matrix transformations of tori. J. Lond. Math. Soc. (2) 60 (1999), 381–398.CrossRefGoogle Scholar
Hussain, M. and Wang, W.. Higher-dimensional shrinking target problem for beta dynamical systems. J. Aust. Math. Soc. 114(3) (2023), 289–311.CrossRefGoogle Scholar
Koivusalo, H., Liao, L. and Rams, M.. Path-dependent shrinking targets in generic affine iterated function systems. Preprint, 2022, arXiv:2210.05362.Google Scholar
Li, B., Liao, L., Velani, S., Wang, B. and Zorin, E.. Diophantine approximation and the Mass Transference Principle: incorporating the unbounded setup. Preprint, 2022, arXiv:2410.18578.Google Scholar
Li, B., Liao, L., Velani, S. and Zorin, E.. The shrinking target problem for matrix transformations of tori: revisiting the standard problem. Adv. Math. 421 (2023), Paper no. 108994.CrossRefGoogle Scholar
Li, B., Wang, B., Wu, J. and Xu, J.. The shrinking target problem in the dynamical system of continued fractions. Proc. Lond. Math. Soc. (3) 108(1) (2014), 159–186.CrossRefGoogle Scholar
Li, Y.. Some results on beta-expansions and generalized Thue–Morse sequences. PhD Thesis, Sorbonne Université; South China University of Technology, 2021.Google Scholar
Persson, T. and Reeve, H.. A Frostman type lemma for sets with large intersections, and an application to Diophantine approximation. Proc. Edinb. Math. Soc. (2) 58(2) (2015), 521–542.CrossRefGoogle Scholar
Rényi, A.. Representations for real numbers and their ergodic properties. Acta Math. Hungar. 8 (1957), 477–493.CrossRefGoogle Scholar
Shen, L. and Wang, B.. Shrinking target problems for beta-dynamical system. Sci. China Math. 56 (2013), 91–104.CrossRefGoogle Scholar
Wang, B. and Wu, J.. Mass transference principle from rectangles to rectangles in Diophantine approximation. Math. Ann. 381 (2021), 243–317.CrossRefGoogle Scholar
Wang, B. and Zhang, G.. A dynamical dimension transference principle for dynamical Diophantine approximation. Math. Z. 298 (2021), 161–191.CrossRefGoogle Scholar