Article contents
On the equidistribution of unstable curves for pseudo-Anosov diffeomorphisms of compact surfaces
Published online by Cambridge University Press: 07 December 2021
Abstract
We prove that the asymptotics of ergodic integrals along an invariant foliation of a toral Anosov diffeomorphism, or of a pseudo-Anosov diffeomorphism on a compact orientable surface of higher genus, is determined (up to a logarithmic error) by the action of the diffeomorphism on the cohomology of the surface. As a consequence of our argument and of the results of Giulietti and Liverani [Parabolic dynamics and anisotropic Banach spaces. J. Eur. Math. Soc. (JEMS)21(9) (2019), 2793–2858] on horospherical averages, toral Anosov diffeomorphisms have no Ruelle resonances in the open interval 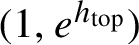 $(1, e^{h_{\mathrm {top}}})$
.
$(1, e^{h_{\mathrm {top}}})$
.
Keywords
- Type
- Original Article
- Information
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press
Footnotes
In memory of Anatole Katok, with admiration and gratitude
References
- 6
- Cited by



