Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-25T14:14:15.174Z Has data issue: false hasContentIssue false
  • English
  • Français

On the conservative pasting lemma

Part of: Smooth dynamical systems: general theory

Published online by Cambridge University Press:  17 October 2018

PEDRO TEIXEIRA
PEDRO TEIXEIRA*
Affiliation:
Centro de Matemática da Universidade do Porto, Rua do Campo Alegre, 687, 4169-007 Porto, Portugal email [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Several perturbation tools are established in the volume-preserving setting allowing for the pasting, extension, localized smoothing and local linearization of vector fields. The pasting and the local linearization hold in all classes of regularity ranging from 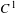$C^{1}$ to 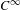$C^{\infty }$ (Hölder included). For diffeomorphisms, a conservative linearized version of Franks’ lemma is proved in the 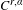$C^{r,\unicode[STIX]{x1D6FC}}$ ($r\in \mathbb{Z}^{+}$, 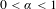$0<\unicode[STIX]{x1D6FC}<1$) and 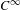$C^{\infty }$ settings, the resulting diffeomorphism having the same regularity as the original one.

Keywords

divergence-free vector fieldlocalized smoothinglocal linearizationvolume-preserving diffeomorphismsFranks’ lemma

MSC classification

Primary: 37C10: Vector fields, flows, ordinary differential equations
Type
Original Article
Information
Ergodic Theory and Dynamical Systems , Volume 40 , Issue 5 , May 2020 , pp. 1402 - 1440
Copyright
© Cambridge University Press, 2018

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Arbieto, A. and Matheus, C.. A pasting lemma and some applications for conservative systems. Ergod. Th. & Dynam. Sys. 27 (2007), 1399–1417.Google Scholar
Arbieto, A. and Matheus, C.. Corrigendum to ‘A pasting lemma and some applications for conservative systems’, July 2, 2013, http://w3.impa.br/∼cmateus/files/pasting-corrigendum.pdf.Google Scholar
Avila, A.. On the regularization of conservative maps. Acta Math. 205(1) (2010), 5–18.Google Scholar
Bonnatti, C. and Crovisier, S.. Récurrence et généricité. Invent. Math. 158 (2004), 33–104.Google Scholar
Csató, G., Dacorogna, B. and Kneuss, O.. The Pullback Equation for Differential Forms (Progress in Nonlinear Differential Equations and their Applications, 83). Birkhäuser/Springer, New York, 2012.Google Scholar
Dacorogna, B. and Moser, J.. On a partial differential equation involving the Jacobian determinant. Ann. Inst. H. Poincaré Anal. Non Linéaire 7(1) (1990), 1–26.Google Scholar
Franks, J.. Necessary conditions for stability of diffeomorphisms. Trans. Amer. Math. Soc. 158 (1971), 301–308.Google Scholar
Gilbarg, D. and Trudinger, N.. Elliptic Partial Differential Equations of Second Order (Classics in Mathematics). Reprint of the 1998 edition. Springer, Berlin, 2001.Google Scholar
Rodriguez Hertz, F., Rodriguez Hertz, M., Tahzibi, A. and Ures, R.. Creation of blenders in the conservative setting. Nonlinearity 23 (2010), 211–223.Google Scholar
Hirsch, M.. Differential Topology (Graduate Texts in Mathematics, 33). Springer, Berlin, 1994, Corrected reprint of the 1976 original.Google Scholar
Lang, S.. Introduction to Differentiable Manifolds (Universitext), 2nd edn. Springer, Berlin, 2002.Google Scholar
Moser, J.. On the volume elements on a manifold. Trans. Amer. Math. Soc. 120 (1965), 286–294.Google Scholar
Pugh, C. and Robinson, C.. The C 1 closing lemma, including Hamiltonians. Ergod. Th. & Dynam. Sys. 3 (1983), 261–313.Google Scholar
Rivière, T. and Ye, D.. Resolutions of the prescribed volume form equation. Nonlinear Differ. Equ. Appl. 3 (1996), 323–369.Google Scholar
Teixeira, P.. Dacorogna–Moser theorem on the Jacobian determinant equation with control of support. Discrete Contin. Dyn. Syst. 37 (2017), 4071–4089.Google Scholar
Teixeira, P.. Gluing conservative diffeomorphisms on manifolds. to appear.Google Scholar
Zuppa, C.. Régularisation C ∞ des champs vectoriels qui préservent l’élément de volume. Bol. Soc. Brasil. Mat. 10(2) (1979), 51–56.Google Scholar