Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-26T01:00:28.807Z Has data issue: false hasContentIssue false
  • English
  • Français

Incomparable actions of free groups

Published online by Cambridge University Press:  12 May 2016

CLINTON T. CONLEY  and
BENJAMIN D. MILLER
CLINTON T. CONLEY
Affiliation:
Department of Mathematical Sciences, Carnegie Mellon University, Pittsburgh, PA 15213, USA email [email protected]
BENJAMIN D. MILLER
Affiliation:
Kurt Gödel Research Center for Mathematical Logic, Universität Wien, Währinger Straße 25, 1090 Wien, Austria email [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

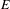Suppose that $X$ is a Polish space, $E$ is a countable Borel equivalence relation on 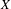$X$, and $\unicode[STIX]{x1D707}$ is an 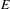$E$-invariant Borel probability measure on 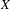$X$. We consider the circumstances under which for every countable non-abelian free group 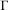$\unicode[STIX]{x1D6E4}$, there is a Borel sequence 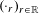$(\cdot _{r})_{r\in \mathbb{R}}$ of free actions of 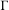$\unicode[STIX]{x1D6E4}$ on 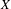$X$, generating subequivalence relations 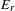$E_{r}$ of 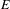$E$ with respect to which $\unicode[STIX]{x1D707}$ is ergodic, with the further property that $(E_{r})_{r\in \mathbb{R}}$ is an increasing sequence of relations which are pairwise incomparable under $\unicode[STIX]{x1D707}$-reducibility. In particular, we show that if 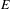$E$ satisfies a natural separability condition, then this is the case as long as there exists a free Borel action of a countable non-abelian free group on $X$, generating a subequivalence relation of 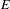$E$ with respect to which $\unicode[STIX]{x1D707}$ is ergodic.

Type
Research Article
Information
Ergodic Theory and Dynamical Systems , Volume 37 , Issue 7 , October 2017 , pp. 2084 - 2098
Copyright
© Cambridge University Press, 2016 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Conley, C. T. and Miller, B. D.. Measure reducibility of countable Borel equivalence relations. Preprint, 2014. Available at https://dl.dropboxusercontent.com/u/47430894/Web/publications/rigidity.pdf.Google Scholar
Dougherty, R., Jackson, S. C. and Kechris, A. S.. The structure of hyperfinite Borel equivalence relations. Trans. Amer. Math. Soc. 341(1) (1994), 193–225.Google Scholar
Feldman, J. and Moore, C. C.. Ergodic equivalence relations, cohomology, and von Neumann algebras. I. Trans. Amer. Math. Soc. 234(2) (1977), 289–324.Google Scholar
Gaboriau, D. and Lyons, R.. A measurable-group-theoretic solution to von Neumann’s problem. Invent. Math. 177(3) (2009), 533–540.Google Scholar
Gaboriau, D. and Popa, S.. An uncountable family of nonorbit equivalent actions of F n . J. Amer. Math. Soc. 18(3) (2005), 547–559.CrossRefGoogle Scholar
Hjorth, G.. Treeable equivalence relations. J. Math. Log. 12(1) (2012), 1250003, 21.Google Scholar
Jackson, S. C., Kechris, A. S. and Louveau, A.. Countable Borel equivalence relations. J. Math. Log. 2(1) (2002), 1–80.Google Scholar
Kechris, A. S.. Classical Descriptive Set Theory (Graduate Texts in Mathematics, 156) . Springer, New York, 1995.Google Scholar
Kechris, A. S. and Miller, B. D.. Topics in Orbit Equivalence (Lecture Notes in Mathematics, 1852) . Springer, Berlin, 2004.Google Scholar
Miller, B. D.. Incomparable treeable equivalence relations. J. Math. Log. 12(1) (2012), 1250004, 11.Google Scholar
Nadkarni, M. G.. Basic Ergodic Theory (Birkhäuser Advanced Texts: Basler Lehrbücher) , 2nd edn. Birkhäuser, Basel, 1998.Google Scholar
Törnquist, A.. Orbit equivalence and actions of F n . J. Symbolic Logic 71(1) (2006), 265–282.Google Scholar
Zimmer, R. J.. Ergodic Theory and Semisimple Groups (Monographs in Mathematics, 81) . Birkhäuser, Basel, 1984.Google Scholar