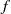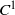Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Arbieto, Alexander
Senos, Laura
and
Sodero, Tatiana
2012.
The specification property for flows from the robust and generic viewpoint.
Journal of Differential Equations,
Vol. 253,
Issue. 6,
p.
1893.
Lee, Manseob
2013.
Symplectic diffeomorphisms with inverse shadowing.
Journal of Inequalities and Applications,
Vol. 2013,
Issue. 1,
Bessa, Mario
2013.
C1-STABLY SHADOWABLE CONSERVATIVE DIFFEOMORPHISMS ARE ANOSOV.
Bulletin of the Korean Mathematical Society,
Vol. 50,
Issue. 5,
p.
1495.
Bessa, Mário
Rocha, Jorge
and
Torres, Maria Joana
2013.
Hyperbolicity and stability for Hamiltonian flows.
Journal of Differential Equations,
Vol. 254,
Issue. 1,
p.
309.
CATALAN, THIAGO
and
TAHZIBI, ALI
2014.
A lower bound for topological entropy of generic non-Anosov symplectic diffeomorphisms.
Ergodic Theory and Dynamical Systems,
Vol. 34,
Issue. 5,
p.
1503.
Ribeiro, Raquel
2014.
Hyperbolicity and types of shadowing for $C^1$ generic vector fields.
Discrete & Continuous Dynamical Systems - A,
Vol. 34,
Issue. 7,
p.
2963.
Lee, Manseob
2014.
Continuum-wise expansive diffeomorphisms and conservative systems.
Journal of Inequalities and Applications,
Vol. 2014,
Issue. 1,
Ahn, Jiweon
Lee, Manseob
and
Oh, Jumi
2015.
Measure expansivity for C1-conservative systems.
Chaos, Solitons & Fractals,
Vol. 81,
Issue. ,
p.
400.
BESSA, Mário
LEE, Manseob
and
WEN, Xiao
2015.
Shadowing, expansiveness and specification for C1-conservative systems.
Acta Mathematica Scientia,
Vol. 35,
Issue. 3,
p.
583.
Lee, Manseob
2016.
The barycenter property for robust and generic diffeomorphisms.
Acta Mathematica Sinica, English Series,
Vol. 32,
Issue. 8,
p.
975.
Lee, Manseob
2017.
Symplectic diffeomorphisms with limit shadowing.
Asian-European Journal of Mathematics,
Vol. 10,
Issue. 02,
p.
1750068.
Lee, Manseob
2019.
Asymptotic orbital shadowing property for diffeomorphisms.
Open Mathematics,
Vol. 17,
Issue. 1,
p.
191.