Hostname: page-component-5cf477f64f-h6p2m Total loading time: 0 Render date: 2025-04-04T00:12:32.247Z Has data issue: false hasContentIssue false
  • English
  • Français

Homogeneous orbit closures and applications

Published online by Cambridge University Press:  28 April 2011

ELON LINDENSTRAUSS  and
URI SHAPIRA
ELON LINDENSTRAUSS
Affiliation:
Einstein Institute of Mathematics, Edmond J. Safra Campus, Givat Ram, The Hebrew University of Jerusalem, Jerusalem, 91904, Israel (email: [email protected])
URI SHAPIRA
Affiliation:
ETH Zürich, Departement Mathematik, Rämistrasse 101, 8092 Zürich, Switzerland (email: [email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

We give new classes of examples of orbits of the diagonal group in the space of unit volume lattices in ℝd for d≥3 with nice (homogeneous) orbit closures, as well as examples of orbits with explicitly computable but irregular orbit closures. We give Diophantine applications to the former; for instance, we show that, for all γ,δ∈ℝ, 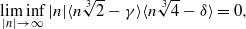where 〈c〉 denotes the distance of a real number c to the integers.

Type
Research Article
Information
Ergodic Theory and Dynamical Systems , Volume 32 , Issue 2: Daniel J. Rudolph – in Memoriam , April 2012 , pp. 785 - 807
Copyright
Copyright © Cambridge University Press 2011

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

[CSD55]Cassels, J. W. S. and Swinnerton-Dyer, H. P. F.. On the product of three homogeneous linear forms and the indefinite ternary quadratic forms. Philos. Trans. R. Soc. Lond. Ser. A 248 (1955), 73–96; MR 0070653(17,14f).Google Scholar
[Dav51]Davenport, H.. Indefinite binary quadratic forms, and Euclid’s algorithm in real quadratic fields. Proc. Lond. Math. Soc. (2) 53 (1951), 65–82; MR 0041883(13,15f).Google Scholar
[EKL06]Einsiedler, M., Katok, A. and Lindenstrauss, E.. Invariant measures and the set of exceptions to Littlewood’s conjecture. Ann. of Math. (2) 164(2) (2006), 513–560; MR 2247967(2007j:22032).Google Scholar
[EL10]Einsiedler, M. and Lindenstrauss, E.. Diagonal actions on locally homogeneous spaces. Homogeneous Flows, Moduli Spaces and Arithmetic (Clay Mathematics Proceedings, 10). American Mathematical Society, Providence, RI, 2010, pp. 155–241.Google Scholar
[EMS97]Eskin, A., Mozes, S. and Shah, N.. Non-divergence of translates of certain algebraic measures. Geom. Funct. Anal. 7(1) (1997), 48–80; MR 1437473(98d:22006).Google Scholar
[LW01]Lindenstrauss, E. and Weiss, B.. On sets invariant under the action of the diagonal group. Ergod. Th. & Dynam. Sys. 21(5) (2001), 1481–1500; MR 1855843(2002j:22009).Google Scholar
[Mar89]Margulis, G. A.. Discrete subgroups and ergodic theory. Number Theory Trace Formulas and Discrete Groups (Oslo, 1987). Academic Press, Boston, MA, 1989, pp. 377–398; MR 993328(90k:22013a).Google Scholar
[Mar97]Margulis, G. A.. Oppenheim conjecture. Fields Medallists’ Lectures (World Scientific Series, 20th Century Mathematics, 5). World Scientific Publishers, River Edge, NJ, 1997, pp. 272–327; MR 1622909(99e:11046).Google Scholar
[Mar00]Margulis, G. A.. Problems and conjectures in rigidity theory. Mathematics: Frontiers and Perspectives. American Mathematical Society, Providence, RI, 2000, pp. 161–174; MR 1754775(2001d:22008).Google Scholar
[Mau]Maucourant, F.. A non-homogeneous orbit closure of a diagonal subgroup. Ann. of Math. (2) 171(1) (2010), 557–570.Google Scholar
[MT96]Margulis, G. A. and Tomanov, G. M.. Measure rigidity for almost linear groups and its applications. J. Anal. Math. 69 (1996), 25–54; MR 1428093(98i:22016).Google Scholar
[PR72]Prasad, G. and Raghunathan, M. S.. Cartan subgroups and lattices in semi-simple groups. Ann. of Math. (2) 96 (1972), 296–317; MR 0302822(46#1965).Google Scholar
[Rat91a]Ratner, M.. On Raghunathan’s measure conjecture. Ann. of Math. (2) 134(3) (1991), 545–607; MR 1135878(93a:22009).Google Scholar
[Rat91b]Ratner, M.. Raghunathan’s topological conjecture and distributions of unipotent flows. Duke Math. J. 63(1) (1991), 235–280; MR 1106945(93f:22012).Google Scholar
[Sha]Shapira, U.. A solution to a problem of Cassels and Diophantine properties of cubic numbers. Ann of Math. (2) 173(1) (2011), 543–557; available on arXiv at http://arxiv.org/abs/0810.4289v2.Google Scholar
[TW03]Tomanov, G. and Weiss, B.. Closed orbits for actions of maximal tori on homogeneous spaces. Duke Math. J. 119(2) (2003), 367–392; MR 1997950(2004g:22006).Google Scholar