Article contents
Hausdorff dimension of divergent diagonal geodesics on product of finite-volume hyperbolic spaces
Published online by Cambridge University Press: 25 September 2017
Abstract
In this paper, we consider the product space of several non-compact finite-volume hyperbolic spaces, 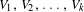 $V_{1},V_{2},\ldots ,V_{k}$ of dimension
$V_{1},V_{2},\ldots ,V_{k}$ of dimension  $n$. Let
$n$. Let  $\text{T}^{1}(V_{i})$ denote the unit tangent bundle of
$\text{T}^{1}(V_{i})$ denote the unit tangent bundle of 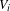 $V_{i}$ and
$V_{i}$ and 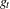 $g_{t}$ denote the geodesic flow on
$g_{t}$ denote the geodesic flow on 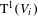 $\text{T}^{1}(V_{i})$ for each
$\text{T}^{1}(V_{i})$ for each 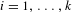 $i=1,\ldots ,k$. We define
$i=1,\ldots ,k$. We define  $$\begin{eqnarray}{\mathcal{D}}_{k}:=\{(v_{1},\ldots ,v_{k})\,\in \,\text{T}^{1}(V_{1})\times \cdots \times \text{T}^{1}(V_{k})\,:\,(g_{t}(v_{1}),\ldots ,g_{t}(v_{k}))\text{ diverges as }t\rightarrow \infty \}.\end{eqnarray}$$
$$\begin{eqnarray}{\mathcal{D}}_{k}:=\{(v_{1},\ldots ,v_{k})\,\in \,\text{T}^{1}(V_{1})\times \cdots \times \text{T}^{1}(V_{k})\,:\,(g_{t}(v_{1}),\ldots ,g_{t}(v_{k}))\text{ diverges as }t\rightarrow \infty \}.\end{eqnarray}$$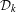 ${\mathcal{D}}_{k}$ is equal to
${\mathcal{D}}_{k}$ is equal to  $k(2n-1)-((n-1)/2)$. This extends a result of Cheung.
$k(2n-1)-((n-1)/2)$. This extends a result of Cheung.
- Type
- Original Article
- Information
- Copyright
- © Cambridge University Press, 2017
References
- 2
- Cited by


