Hostname: page-component-745bb68f8f-kw2vx Total loading time: 0 Render date: 2025-01-11T10:15:38.826Z Has data issue: false hasContentIssue false
  • English
  • Français

Generic properties of homeomorphisms preserving a given dynamical simplex

Part of: Topological dynamics

Published online by Cambridge University Press:  25 October 2021

JULIEN MELLERAY
JULIEN MELLERAY*
Affiliation:
Univ Lyon, Université Claude Bernard Lyon 1, CNRS UMR 5208, Institut Camille Jordan, F-69622 Villeurbanne, France
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Given a dynamical simplex K on a Cantor space X, we consider the set $G_K^*$ of all homeomorphisms of X which preserve all elements of K and have no non-trivial clopen invariant subset. Generalizing a theorem of Yingst, we prove that for a generic element g of 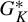$G_K^*$ the set of invariant measures of g is equal to K. We also investigate when there exists a generic conjugacy class in 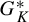$G_K^*$ and prove that this happens exactly when K has only one element, which is the unique invariant measure associated to some odometer; and that in that case the conjugacy class of this odometer is generic in $G_K^*$ .

Keywords

topological dynamicsinvariant measuresminimal homeomorphismsBaire category

MSC classification

Primary: 37B05: Transformations and group actions with special properties (minimality, distality, proximality, etc.)
Secondary: 37B99: None of the above, but in this section
Type
Original Article
Information
Ergodic Theory and Dynamical Systems , Volume 43 , Issue 2 , February 2023 , pp. 646 - 662
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Akin, E.. Good measures on Cantor space. Trans. Amer. Math. Soc. 357(7) (2005), 2681–2722 (electronic).CrossRefGoogle Scholar
Bezuglyi, S., Dooley, A. H. and Kwiatkowski, J.. Topologies on the group of homeomorphisms of a Cantor set. Topol. Methods Nonlinear Anal. 27(2) (2006), 299–331.Google Scholar
Ben Yaacov, I., Melleray, J. and Tsankov, T.. Metrizable universal minimal flows of Polish groups have a comeagre orbit. Geom. Funct. Anal. 27(1) (2017), 67–77.CrossRefGoogle Scholar
Dahl, H.. Cantor minimal systems and AF equivalence relations. PhD Thesis, Norwegian University of Science and Technology, Trondheim (Denmark), 2008.Google Scholar
Dougherty, R., Mauldin, R. D. and Yingst, A.. On homeomorphic Bernoulli measures on the Cantor space. Trans. Amer. Math. Soc. 359(12) (2007), 6155–6166.CrossRefGoogle Scholar
Gao, S.. Invariant Descriptive Set Theory (Pure and Applied Mathematics, 293). CRC Press, Boca Raton, FL, 2009.Google Scholar
Glasner, E. and Weiss, B.. Weak orbit equivalence of Cantor minimal systems. Internat. J. Math. 6(4) (1995), 559–579.CrossRefGoogle Scholar
Ibarlucía, T. and Melleray, J.. Dynamical simplices and minimal homeomorphisms. Proc. Amer. Math. Soc. 145(11) (2017), 4981–4994.CrossRefGoogle Scholar
Melleray, J.. Dynamical simplices and Fraïssé theory. Ergod. Th. & Dynam. Sys. 39(11) (2019), 3111–3126.CrossRefGoogle Scholar
Yingst, A. Q.. A context in which finite or unique ergodicity is generic. Ergod. Th. & Dynam. Sys. 41 (2020), 1–23.Google Scholar