No CrossRef data available.
Article contents
Examples of distorted interval diffeomorphisms of intermediate regularity
Part of:
Smooth dynamical systems: general theory
Low-dimensional dynamical systems
Special aspects of infinite or finite groups
Published online by Cambridge University Press: 08 September 2021
Abstract
We improve a recent construction of Andrés Navas to produce the first examples of
$C^2$
-undistorted diffeomorphisms of the interval that are
$C^{1+\alpha }$
-distorted (for every
${\alpha < 1}$
). We do this via explicit computations due to the failure of an extension to class
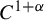$C^{1+\alpha }$
of a classical lemma related to the work of Nancy Kopell.
- Type
- Original Article
- Information
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press
References
Bonatti, C. and Farinelli, É.. Centralizers of
${C}^1$
-contractions of the half line. Groups Geom. Dyn. 9(3) (2015), 831–889.CrossRefGoogle Scholar
${C}^1$
-contractions of the half line. Groups Geom. Dyn. 9(3) (2015), 831–889.CrossRefGoogle ScholarCastro, G., Jorquera, E. and Navas, A.. Sharp regularity for certain nilpotent group actions on the interval. Math. Ann. 359(1–2) (2014), 101–152.CrossRefGoogle Scholar
Deroin, B., Kleptsyn, V. and Navas, A.. Sur la dynamique unidimensionnelle en régularité intermédiaire. Acta Math. 199(2) (2007), 199–262.CrossRefGoogle Scholar
Druck, S. and Firmo, S.. Periodic leaves for diffeomorphisms preserving codimension one foliations. J. Math. Soc. Japan 55(1) (2003), 13–37.CrossRefGoogle Scholar
Eynard-Bontemps, H. and Navas, A.. Mather invariant, conjugates, and distortion for diffeomorphisms of the interval. J. Funct. Analysis 281(9) (2021), 109–149.CrossRefGoogle Scholar
Gromov, M.. Asymptotic invariants of infinite groups. Geometric Group Theory (Sussex, 1991) (London Mathematical Society Lecture Notes Series, 182). Vol. 2. Cambridge University Press, Cambridge, 1993, pp. 1–295.Google Scholar
Kim, S. H. and Koberda, T.. Diffeomorphism groups of critical regularity. Invent. Math. 221(2) (2020), 421–501.CrossRefGoogle Scholar
Kleptsyn, V. and Navas, A.. A Denjoy type theorem for commuting circle diffeomorphisms with derivatives having different Hölder differentiability classes. Mosc. Math. J. 8(3) (2008), 477–492.CrossRefGoogle Scholar
Kopell, N.. Commuting diffeomorphisms. Global Analysis (Berkeley, CA, 1968) (Proceedings of Symposia in Pure Mathematics, XIV). American Mathematical Society, Providence, RI, 1970, pp. 165–184.Google Scholar
Mann, K. and Wolff, M.. Reconstructing maps out of groups. Ann. Sci. Éc. Norm. Supér. (4), to appear.Google Scholar
Navas, A.. Groups of Circle Diffeomorphisms (Chicago Lectures in Mathematics). University of Chicago Press, Chicago, IL, 2011.CrossRefGoogle Scholar
Navas, A.. On centralizers of interval diffeomorphisms in critical (intermediate) regularity. J. Anal. Math. 121 (2013), 1–30.CrossRefGoogle Scholar
Navas, A.. On conjugates and the asymptotic distortion of 1-dimensional
${C}^{1+\mathrm{bv}}$
diffeomorphisms. Preprint, 2021, arXiv:1811.06077.CrossRefGoogle Scholar
${C}^{1+\mathrm{bv}}$
diffeomorphisms. Preprint, 2021, arXiv:1811.06077.CrossRefGoogle ScholarNavas, A.. (Un)distorted diffeomorphisms in different regularities. Israel J. Math., doi: 10.1007/s11856-021-2188-z. Published online 21 August 2021.CrossRefGoogle Scholar
Pixton, D.. Nonsmoothable, unstable group actions. Trans. Amer. Math. Soc. 229 (1977), 259–268.CrossRefGoogle Scholar
Rosendal, C.. Coarse Geometry of Topological Groups. Unpublished book.Google Scholar
Tsuboi, T.. Homological and dynamical study on certain groups of Lipschitz homeomorphisms of the circle. J. Math. Soc. Japan 47 (1995), 1–30.CrossRefGoogle Scholar