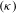Hostname: page-component-f554764f5-44mx8 Total loading time: 0 Render date: 2025-04-11T09:08:37.541Z Has data issue: false hasContentIssue false
  • English
  • Français

Effective results on nonlinear ergodic averages in CAT$(\unicode[STIX]{x1D705})$ spaces

Published online by Cambridge University Press:  21 July 2015

LAURENŢIU LEUŞTEAN Open the ORCID record for LAURENŢIU LEUŞTEAN [Opens in a new window]  and
ADRIANA NICOLAE
LAURENŢIU LEUŞTEAN
Affiliation:
Faculty of Mathematics and Computer Science, University of Bucharest, Academiei 14, P.O. Box 010014, Bucharest, Romania Simion Stoilow Institute of Mathematics of the Romanian Academy, P.O. Box 1-764, RO-014700 Bucharest, Romania email [email protected]
ADRIANA NICOLAE
Affiliation:
Department of Mathematics, Babeş-Bolyai University, Kogălniceanu 1, 400084 Cluj-Napoca, Romania Simion Stoilow Institute of Mathematics of the Romanian Academy, Research group of the project PD-3-0152, P.O. Box 1-764, RO-014700 Bucharest, Romania email [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

In this paper we apply proof mining techniques to compute, in the setting of CAT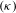$(\unicode[STIX]{x1D705})$ spaces (with 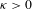$\unicode[STIX]{x1D705}>0$), effective and highly uniform rates of asymptotic regularity and metastability for a nonlinear generalization of the ergodic averages, known as the Halpern iteration. In this way, we obtain a uniform quantitative version of a nonlinear extension of the classical von Neumann mean ergodic theorem.

Type
Research Article
Information
Ergodic Theory and Dynamical Systems , Volume 36 , Issue 8 , December 2016 , pp. 2580 - 2601
Copyright
© Cambridge University Press, 2015 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Avigad, J., Gerhardy, P. and Towsner, H.. Local stability of ergodic averages. Trans. Amer. Math. Soc. 362 (2010), 261–288.CrossRefGoogle Scholar
Avigad, J. and Rute, J.. Oscillation and the mean ergodic theorem for uniformly convex Banach spaces. Ergod. Th. & Dynam. Sys.; available on CJO2014. doi:10.1017/etds.2013.90.Google Scholar
Birkhoff, G.. The mean ergodic theorem. Duke Math. J. 5 (1939), 19–20.CrossRefGoogle Scholar
Bridson, M. and Haefliger, A.. Metric Spaces of Non-positive Curvature. Springer, Berlin, 1999.CrossRefGoogle Scholar
Browder, F. E.. Existence and approximation of solutions of nonlinear variational inequalities. Proc. Natl Acad. Sci. USA 56 (1966), 1080–1086.CrossRefGoogle ScholarPubMed
Browder, F. E.. Convergence of approximants to fixed points of nonexpansive nonlinear mappings in Banach spaces. Arch. Ration. Mech. Anal. 24 (1967), 82–90.CrossRefGoogle Scholar
Bruck, R. E. and Reich, S.. Accretive operators, Banach limits, and dual ergodic theorems. Bull. Acad. Polon. Sci. Sér. Sci. Math. 29 (1981), 585–589.Google Scholar
Goebel, K. and Reich, S.. Uniform Convexity, Hyperbolic Geometry, and Nonexpansive Mappings. Marcel Dekker, Inc., New York and Basel, 1984.Google Scholar
Halpern, B.. Fixed points of nonexpanding maps. Bull. Amer. Math. Soc. (N.S.) 73 (1967), 957–961.CrossRefGoogle Scholar
Kirk, W. A.. Geodesic geometry and fixed point theory. Seminar of Mathematical Analysis (Malaga/Seville, 2002/2003 (Colecc. Abierta, Vol. 64) . Univ. Seville Secr. Publ., Seville, 2003, pp. 195–225.Google Scholar
Kohlenbach, U.. Applied Proof Theory: Proof Interpretations and their Use in Mathematics. Springer, Heidelberg-Berlin, 2008.Google Scholar
Kohlenbach, U.. On quantitative versions of theorems due to F. E. Browder and R. Wittmann. Adv. Math. 266 (2011), 2764–2795.CrossRefGoogle Scholar
Kohlenbach, U.. Some logical metatheorems with applications in functional analysis. Trans. Amer. Math. Soc. 357 (2005), 89–128.CrossRefGoogle Scholar
Kohlenbach, U. and Leuştean, L.. A quantitative mean ergodic theorem for uniformly convex Banach spaces. Ergod. Th. & Dynam. Sys. 29 (2009), 1907–1915 Erratum: Ergod. Th. & Dynam. Sys. 29 (2009) 1995.CrossRefGoogle Scholar
Kohlenbach, U. and Leuştean, L.. Asymptotically nonexpansive mappings in uniformly convex hyperbolic spaces. J. Eur. Math. Soc. 12 (2010), 71–92.CrossRefGoogle Scholar
Kohlenbach, U. and Leuştean, L.. On the computational content of convergence proofs via Banach limits. Philos. Trans. R. Soc. Lond. A 370(1971) (2012), 3449–3463.Google ScholarPubMed
Kohlenbach, U. and Leuştean, L.. Effective metastability of Halpern iterates in CAT(0) spaces. Adv. Math. 231 (2012), 2526–2556.CrossRefGoogle Scholar
Kohlenbach, U. and Leuştean, L.. Addendum to ‘Effective metastability of Halpern iterates in CAT(0) spaces’. Adv. Math. 250 (2014), 650–651.CrossRefGoogle Scholar
Kohlenbach, U. and Safarik, P.. Fluctuations, effective learnability and metastability in analysis. Ann. Pure Appl. Logic 165 (2014), 266–304.CrossRefGoogle Scholar
Kreisel, G.. On the interpretation of non-finitist proofs, part I. J. Symbolic Logic 16 (1951), 241–267.Google Scholar
Kreisel, G.. On the interpretation of non-finitist proofs, part II. J. Symbolic Logic 17 (1952), 43–88.CrossRefGoogle Scholar
Krengel, U.. On the speed of convergence in the ergodic theorem. Monatsh. Math. 86 (1978/79), 3–6.CrossRefGoogle Scholar
Leuştean, L.. Rates of asymptotic regularity for Halpern iterations of nonexpansive mappings. J. Universal Comp. Sci. 13 (2007), 1680–1691.Google Scholar
Leuştean, L.. Proof mining in metric fixed point theory and ergodic theory. Habilitation Thesis, Technische Universität Darmstadt, 2009.Google Scholar
Lorentz, G. G.. A contribution to the theory of divergent series. Acta Math. 80 (1948), 167–190.CrossRefGoogle Scholar
Pia̧tek, B.. Halpern iteration in CAT(𝜅) spaces. Acta Math. Sin. (Engl. Ser.) 27 (2011), 635–646.CrossRefGoogle Scholar
Reich, S.. Almost convergence and nonlinear ergodic theorems. J. Approx. Theory 24 (1978), 269–272.CrossRefGoogle Scholar
Reich, S.. Strong convergence theorems for resolvents of accretive operators in Banach spaces. J. Math. Anal. Appl. 75 (1980), 287–292.CrossRefGoogle Scholar
Saejung, S.. Halpern iterations in CAT(0) spaces. Fixed Point Theory Appl. 2010 (2010), 471781, 13pp.Google Scholar
Schade, K. and Kohlenbach, U.. Effective metastability for modified Halpern iterations in CAT(0) spaces. Fixed Point Theory Appl. 2012(191) (2012), 19pp.CrossRefGoogle Scholar
Shioji, N. and Takahashi, W.. Strong convergence of approximated sequences for nonexpansive mappings in Banach spaces. Proc. Amer. Math. Soc. 125 (1997), 3641–3645.CrossRefGoogle Scholar
Tao, T.. Soft analysis, hard analysis, and the finite convergence principle. Structure and Randomness: Pages from Year One of a Mathematical Blog. American Mathematical Society, Providence, RI, 2008, Essay first posted May 23, 2007.CrossRefGoogle Scholar
Tao, T.. Norm convergence of multiple ergodic averages for commuting transformations. Ergod. Th. & Dynam. Sys. 28 (2008), 657–688.CrossRefGoogle Scholar
Walsh, M.. Norm convergence of nilpotent ergodic averages. Ann. of Math. (2) 175 (2012), 1667–1688.CrossRefGoogle Scholar
Wittmann, R.. Approximation of fixed points of nonexpansive mappings. Arch. Math. 58 (1992), 486–491.CrossRefGoogle Scholar