Hostname: page-component-78c5997874-g7gxr Total loading time: 0 Render date: 2024-11-19T06:11:46.014Z Has data issue: false hasContentIssue false
  • English
  • Français

Dynamics of iterated function systems on the circle close to rotations

Published online by Cambridge University Press:  23 April 2014

PABLO G. BARRIENTOS  and
ARTEM RAIBEKAS
PABLO G. BARRIENTOS
Affiliation:
Departamento de Matemática PUC-Rio, Marquês de São Vicente 225, Gávea, Rio de Janeiro 225453-900, Brazil email [email protected]
ARTEM RAIBEKAS
Affiliation:
Instituto de Matemática e Estatística, UFF Rua Mário Santos Braga s/n–Campus Valonguinhos, Niterói, Brazil email [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We study the dynamics of iterated function systems generated by a pair of circle diffeomorphisms close to rotations in the 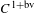$C^{1+\text{bv}}$-topology. We characterize the obstruction to minimality and describe the limit set. In particular, there are no invariant minimal Cantor sets, which can be seen as a Denjoy/Duminy type theorem for iterated systems on the circle.

Type
Research Article
Information
Ergodic Theory and Dynamical Systems , Volume 35 , Issue 5 , August 2015 , pp. 1345 - 1368
Copyright
© Cambridge University Press, 2014 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Barrientos, P. G., Fakhari, A. and Sarizadeh, A.. Ergodicity of expanding minimal actions. Preprint arXiv:1307.6054, 2013.Google Scholar
Barrientos, P. G., Ki, Y. and Raibekas, A.. Symbolic blender-horseshoes and applications. Preprint arXiv:1211.7088, 2012.Google Scholar
Bonatti, C., Díaz, L. J. and Viana, M.. Dynamics Beyond Uniform Hyperbolicity (Encyclopedia of Mathematical Sciences, 102). Springer, Berlin, 2005.Google Scholar
Denjoy, A.. Sur les courbes définies par les équations différentielles à la surface du tore. J. Math. Pures Appl. 11 (1932), 333–375.Google Scholar
Deroin, B., Kleptsyn, V. and Navas, A.. Sur la dynamique unidimensionnelle en régularité intermédiaire. Acta Math. 199(2) (2007), 199–262.CrossRefGoogle Scholar
Duminy, G.. Unpublished manuscript, Université de Lille, 1977.Google Scholar
Ghys, E.. Groups acting on the circle. Enseign. Math. (2) 47(3–4) (2001), 329–407.Google Scholar
Gorodetskiĭ, A. S. and Il’yashenko, Yu. S.. Some new robust properties of invariant sets and attractors of dynamical systems. Funktsional. Anal. i Prilozhen. 33(2) (1999), 16–30 95.CrossRefGoogle Scholar
Gorodetskiĭ, A. S. and Il’yashenko, Yu. S.. Some properties of skew products over a horseshoe and a solenoid. Tr. Mat. Inst. Steklova 231 (Din. Sist., Avtom. i Beskon. Gruppy) 96–118, 2000.Google Scholar
Herman, M.-R.. Sur la conjugaison différentiable des difféomorphismes du cercle à des rotations. Inst. Hautes Études Sci. Publ. Math. 49(49) (1979), 5–233.CrossRefGoogle Scholar
Kleptsyn, V. A. and Nal’skiĭ, M. B.. Convergence of orbits in random dynamical systems on a circle. Funktsional. Anal. i Prilozhen. 38(4) (2004), 36–54 95–96.CrossRefGoogle Scholar
Kleptsyn, V. A. and Volk, D.. Skew products and random walks on intervals. Preprint arXiv:1110.2117, 2013.Google Scholar
Nassiri, M. and Pujals, E. R.. Robust transitivity in Hamiltonian dynamics. Ann. Sci. Éc. Norm. Supér. 45(2) (2012).Google Scholar
Navas, A.. Sur les groupes de difféomorphismes du cercle engendrés par des éléments proches des rotations. Enseign. Math. (2) 50(1–2) (2004), 29–68.Google Scholar
Navas, A.. Groups of Circle Diffeomorphisms (Chicago Lectures in Mathematics). Spanish edn. University of Chicago Press, Chicago, IL, 2011.CrossRefGoogle Scholar
Shinohara, K.. On the minimality of semigroup actions on the interval which are $C^{1}$-close to the identity. Preprint arXiv:1210.0112, 2012.Google Scholar
Shinohara, K.. Some examples of minimal cantor set for IFSs with overlap. Preprint arXiv:1304.5831, 2013.CrossRefGoogle Scholar
Viana, V. and Yang, J.. Physical measures and absolute continuity for one-dimensional center direction. Ann. Inst. H. Poincaré (C) Anal. Non Linéaire 30(5) (2013), 845–877.CrossRefGoogle Scholar