Hostname: page-component-669899f699-7xsfk Total loading time: 0 Render date: 2025-04-24T18:22:40.076Z Has data issue: false hasContentIssue false
  • English
  • Français

Dimension estimates for badly approximable affine forms

Part of: Diophantine approximation, transcendental number theory Measure-theoretic ergodic theory Ergodic theory

Published online by Cambridge University Press:  06 November 2024

TAEHYEONG KIM Open the ORCID record for TAEHYEONG KIM [Opens in a new window] ,
WOOYEON KIM Open the ORCID record for WOOYEON KIM [Opens in a new window]  and
SEONHEE LIM Open the ORCID record for SEONHEE LIM [Opens in a new window]
TAEHYEONG KIM*
Affiliation:
Department of Mathematical Sciences, Seoul National University, Seoul, South Korea
WOOYEON KIM
Affiliation:
Department of Mathematics, ETH Zürich, Zürich, Switzerland (e-mail: [email protected])
SEONHEE LIM
Affiliation:
Department of Mathematical Sciences and Resesarch Institute of Mathematics, Seoul National University, Seoul, South Korea (e-mail: [email protected])
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

For given 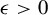$\epsilon>0$ and $b\in \mathbb {R}^m$, we say that a real 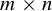$m\times n$ matrix A is 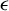$\epsilon $-badly approximable for the target b if

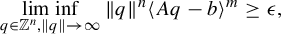$$ \begin{align*}\liminf_{q\in\mathbb{Z}^n, \|q\|\to\infty} \|q\|^n \langle Aq-b\rangle^m \geq \epsilon,\end{align*} $$
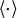where $\langle \cdot \rangle $ denotes the distance from the nearest integral vector. In this article, we obtain upper bounds for the Hausdorff dimensions of the set of 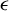$\epsilon $-badly approximable matrices for fixed target b and the set of $\epsilon $-badly approximable targets for fixed matrix A. Moreover, we give a Diophantine condition of A equivalent to the full Hausdorff dimension of the set of 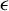$\epsilon $-badly approximable targets for fixed A. The upper bounds are established by effectivizing entropy rigidity in homogeneous dynamics, which is of independent interest. For the A-fixed case, our method also works for the weighted setting where the supremum norms are replaced by certain weighted quasinorms.

Keywords

Diophantine approximationeffectiveHausdorff dimensionbadly approximable

MSC classification

Primary: 11J20: Inhomogeneous linear forms
Secondary: 28D20: Entropy and other invariants 37A17: Homogeneous flows
Type
Original Article
Information
Ergodic Theory and Dynamical Systems , Volume 45 , Issue 5 , May 2025 , pp. 1541 - 1596
Check for updates
Copyright
© The Author(s), 2024. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Athreya, J.. Random affine lattices. Contemp. Math. 639 (2015), 169–174.Google Scholar
Bugeaud, Y., Harrap, S., Kristensen, S. and Velani, S.. On shrinking targets for ${\mathbb{Z}}^{\mathrm{m}}$ actions on tori. Mathematika 56 (2010), 193–202.Google Scholar
Broderick, R. and Kleinbock, D.. Dimension estimates for sets of uniformly badly approximable systems of linear forms. Int. J. Number Theory 11(7) (2015), 2037–2054.Google Scholar
Bugeaud, Y., Kim, D., Lim, S. and Rams, M.. Hausdorff dimension in inhomogeneous Diophantine approximation. Int. Math. Res. Not. IMRN 2021(3) (2021), 2108–2133.CrossRefGoogle Scholar
Bugeaud, Y. and Laurent, M.. On exponents of homogeneous and inhomogeneous Diophantine approximation. Mosc. Math. J. 5 (2005), 747–766.Google Scholar
Bengoechea, P. and Moshchevitin, N.. Badly approximable points in twisted Diophantine approximation and Hausdorff dimension. Acta Arith. 177 (2017), 301–314.CrossRefGoogle Scholar
Cassels, J. W. S.. An Introduction to Diophantine Approximation (Cambridge Tracts in Mathematics and Mathematical Physics, 45). Cambridge University Press, New York, 1957.Google Scholar
Chow, S., Ghosh, A., Guan, L., Marnet, A. and Simmons, D.. Diophantine transference inequalities: weighted, inhomogeneous, and intermediate exponents. Ann. Sc. Norm. Super. Pisa Cl. Sci. (5) 21 (2020), 643–671.Google Scholar
Einsiedler, M. and Lindenstrauss, E.. Diagonal actions on locally homogeneous spaces . Homogeneous Flows, Moduli Spaces and Arithmetic (Clay Mathematics Proceedings, 10). Ed. Einsiedler, M. L., Ellwood, D. A., Eskin, A., Kleinbock, D., Lindenstrauss, E., Margulis, G., Marmi, S. and Yoccoz, J.-C.. American Mathematical Society, Providence, RI, 2010, pp. 155–241.Google Scholar
Einsiedler, M., Lindenstrauss, E. and Ward, T.. Entropy in ergodic theory and homogeneous dynamics. Preprint, to appear. Available at http://www.personal.leeds.ac.uk/~mattbw/entropy.Google Scholar
Einsiedler, M. and Tseng, J.. Badly approximable systems of affine forms, fractals, and Schmidt games. J. Reine Angew. Math. 660 (2011), 83–97.Google Scholar
Einsiedler, M. and Ward, T.. Ergodic Theory with a View Towards Number Theory (Graduate Texts in Mathematics, 259). Springer., London, 2011.CrossRefGoogle Scholar
German, O. N. and Evdokimov, K. G.. A strengthening of Mahler’s transference theorem. Izv. Math. 79(1) (2015), 60–73.Google Scholar
German, O. N.. Transference theorems for Diophantine approximation with weights. Mathematika 66(2) (2020), 325–342.Google Scholar
Groshev, A. V.. Un théorème sur les systémes des formes linéaires (a theorem on a system of linear forms). Dokl. Akad. Nauk SSSR 19 (1938), 151–152 (in Russian).Google Scholar
Harrap, S.. Twisted inhomogeneous Diophantine approximation and badly approximable sets. Acta Arith. 151 (2012), 55–82.Google Scholar
Hussain, M., Kristensen, S. and Simmons, D.. Metrical theorems on systems of small Affine forms. J. Number Theory 213 (2020), 67–100.CrossRefGoogle Scholar
Harrap, S. and Moshchevitin, N.. A note on weighted badly approximable linear forms. Glasg. Math. J. 59 (2017), 349–357.Google Scholar
Kadyrov, S.. Effective uniqueness of Parry measure and exceptional sets in ergodic theory. Monatsh. Math. 178(2) (2015), 237–249.Google Scholar
Khayutin, I.. Large deviations and effective equidistribution. Int. Math. Res. Not. IMRN 10 (2017), 3050–3106.Google Scholar
Kim, W.. Effective equidistribution of expanding translates in the space of affine lattices. Preprint, 2024, arXiv:2110.00706.CrossRefGoogle Scholar
Kadyrov, S., Kleinbock, D., Lindenstrauss, E. and Margulis, G. A.. Singular systems of linear forms and non-escape of mass in the space of lattices. J. Anal. Math. 133 (2017), 253–277.CrossRefGoogle Scholar
Kleinbock, D.. Flows on homogeneous spaces and Diophantine properties of matrices. Duke Math. J. 95(1) (1998), 107–124.Google Scholar
Kleinbock, D.. Badly approximable systems of affine forms. J. Number Theory 79 (1999), 83–102.Google Scholar
Kim, T., Lim, S. and Paulin, F.. On Hausdorff dimension in inhomogeneous Diophantine approximation over global function fields. J. Number Theory 251 (2023), 102–146.Google Scholar
Kleinbock, D. and Margulis, G. A.. Bounded orbits of nonquasiunipotent flows on homogeneous spaces. Trans. Amer. Math. Soc. 171 (1996), 141–172.Google Scholar
Kleinbock, D. and Margulis, G. A.. On effective equidistribution of expanding translates of certain orbits in the space of lattices . Number Theory, Analysis and Geometry. Ed. Goldfeld, D., Jorgenson, J., Jones, P., Ramakrishnan, D., Ribet, K. and Tate, J.. Springer, New York, 2012, pp. 385–396.Google Scholar
Kleinbock, D. and Mirzadeh, S.. Dimension estimates for the set of points with non-dense orbit in homogeneous spaces. Math. Z. 295 (2020), 1355–1383.CrossRefGoogle Scholar
Khalil, O. and Luethi, M.. Random walks, spectral gaps, and Khintchine’s theorem on fractals. Invent. Math. 232(2) (2023), 713–831.CrossRefGoogle Scholar
Kristensen, S., Thorn, R. and Velan, S.. Diophantine approximation and badly approximable set. Adv. Math. 20 (2006), 132–169.CrossRefGoogle Scholar
Kleinbock, D. and Weiss, B.. Modified Schmidt games and Diophantine approximation with weights. Adv. Math. 223(4) (2010), 1276–1298.Google Scholar
Lim, S., de Saxcé, N. and Shapira, U.. Dimension bound for badly approximable grids. Int. Math. Res. Not. IMRN 2019(20) (2019), 6317–6346.Google Scholar
Margulis, G. and Mohammadi, A.. Quantitative version of the Oppenheim conjecture for inhomogeneous quadratic forms. Duke Math. J. 158 (2011), 121–160.CrossRefGoogle Scholar
Polo, F.. Equidistribution in chaotic dynamical systems. PhD Thesis, The Ohio State University, ProQuest LLC, Ann Arbor, MI, 2011.Google Scholar
Pollington, A. and Velani, S.. On simultaneously badly approximable numbers. J. Lond. Math. Soc. (2) 66(1) (2002), 29–40.CrossRefGoogle Scholar
Rühr, R.. Effectivity of uniqueness of the maximal entropy measure on $p$ -adic homogeneous spaces. Ergod. Th. & Dynam. Sys. 36(6) (2002), 1972–1988.Google Scholar
Schmidt, W.. Metrical theorems on fractional parts of sequences. Trans. Amer. Math. Soc. 10 (1964), 493–518.CrossRefGoogle Scholar
Schmidt, W.. Badly approximable numbers and certain games. Trans. Amer. Math. Soc. 110 (1964), 493–518.Google Scholar
Schmidt, W.. Badly approximable systems of linear forms. J. Number Theory 1 (1969), 139–154.Google Scholar
Simmons, D.. A Hausdorff measure version of the Jarník–Schmidt theorem in Diophantine approximation. Math. Proc. Cambridge Philos. Soc. 164(3) (2018), 413–459.Google Scholar
Sprindžuk, V.. Metric Theory of Diophantine Approximation. John Wiley & Sons, New York, 1979.Google Scholar