Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-27T18:23:53.585Z Has data issue: false hasContentIssue false
  • English
  • Français

SEMIPARAMETRIC ESTIMATION OF PARTIALLY LINEAR TRANSFORMATION MODELS UNDER CONDITIONAL QUANTILE RESTRICTION

Published online by Cambridge University Press:  19 December 2014

Zhengyu Zhang
Zhengyu Zhang*
Affiliation:
Shanghai University of Finance and Economics and Shanghai Academy of Social Sciences
*
*Address correspondence to Zhengyu Zhang, Shanghai University of Finance and Economics, 777, Guoding Road, 200433 Shanghai, China; e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

This article is concerned with semiparametric estimation of a partially linear transformation model under conditional quantile restriction with no parametric restriction imposed either on the link functional form or on the error term distribution. We describe for the finite-dimensional parameter a 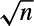$\sqrt n$-consistent estimator which combines the features of Chen (2010)’s maximum integrated score estimator as well as Lee (2003)’s average quantile regression. We show the remaining two infinite-dimensional unknown functions in the model can be separately identified and propose estimators for these functions based on the marginal integration method. Furthermore, a simple approach is proposed to estimate the average partial quantile effect. Two important extensions, i.e., random censoring as well as estimating a transformation model with an endogenous regressor are also considered.

Type
ARTICLES
Information
Econometric Theory , Volume 32 , Issue 2 , April 2016 , pp. 458 - 497
Copyright
Copyright © Cambridge University Press 2014 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Abrevaya, J. (1999) Leapfrog estimation of a fixed-effects model with unknown transformation of the dependent variable. Journal of Econometrics 93, 203–228.Google Scholar
Abrevaya, J. (2003) Pairwise difference rank estimation of the transformation model. Journal of Business and Economic Statistics 21, 437–447.Google Scholar
Abrevaya, J. & Shin, Y. (2011) Rank estimation of partially linear index models. Econometrics Journal 14, 409–437.Google Scholar
Asparouhova, E., Golanski, R., Kasprzyk, K., Sherman, R.P., & Asparouhov, T. (2002) Rank estimators for a transformation model. Econometric Theory 18, 1099–1120.Google Scholar
Box, G.E.P. & Cox, D.R. (1964) An analysis of transformations. Journal of Royal Statistical Society, Series B 26, 211–252.Google Scholar
Cai, J., Fan, J., Jiang, J., & Zhou, H. (2007) Partially linear hazard regression for multivariate survival data. Journal of the American Statistical Association 102, 538–551.Google Scholar
Cai, J., Fan, J., Jiang, J., & Zhou, H. (2008) Partially linear hazard regression with varying coefficients for multivariate survival data. Journal of the Royal Statistical Society, Series B 70, 141–158.CrossRefGoogle Scholar
Carroll, R.J., Fan, J., Gijbels, I., & Wand, M.P. (1997) Generalized partially linear single index models. Journal of the American Statistical Association 92, 477–489.Google Scholar
Cavanagh, C. & Sherman, R.P. (1998) Rank estimators for monotonic index models. Journal of Econometrics 84, 351–381.Google Scholar
Chen, S. (2002) Rank estimation of transformation models. Econometrica 70, 1683–1696.Google Scholar
Chen, S. (2010) An integrated maximum score estimator for a generalized censored quantile regression model. Journal of Econometrics 155, 90–98.Google Scholar
Chen, K., Jin, Z., & Ying, Z. (2002) Semiparametric analysis of transformation models with censored data. Biometrika 89, 659–668.Google Scholar
Fan, J., Gijbels, I., & King, M. (1997) Local likelihood and local partial likelihood in hazard regression. Annals of Statistics 25, 1661–1690.Google Scholar
Fleming, T.R. & Harrington, D.P. (1991) Counting Processes and Survival Analysis. Wiley.Google Scholar
Horowitz, J.L. (1992) A smoothed maximum score estimator for the binary response model. Econometrica 60, 505–531.Google Scholar
Horowitz, J.L. (1996) Semiparametric estimation of a regression model with an unknown transformation of the dependent variable. Econometrica 64, 103–137.Google Scholar
Huang, J. (1999) Efficient estimation of the partially linear additive Cox model. Annals of Statistics 27, 1536–1563.Google Scholar
Huang, J., Kooperberg, C., Stone, C., & Truong, Y. (2000) Functional ANOVA modeling for proportional hazards regression. Annals of Statistics 28, 961–999.Google Scholar
Imbens, G.W. & Newey, W.K. (2009) Identification and estimation of triangular simultaneous equations models without additivity. Econometrica 77, 1481–1512.Google Scholar
Jones, M. & Signorini, D. (1997) A comparison of higher-order bias kernel density estimators. Journal of the American Statistical Association 92, 1063–1073.Google Scholar
Khan, S. & Tamer, E. (2007) Partial rank estimation of duration models with general forms of censoring. Journal of Econometrics 136, 251–280.Google Scholar
Lancaster, T. (1990) The Econometric Analysis of Transition Data. Cambridge University Press.Google Scholar
Lee, S. (2003) Efficient semiparametric estimation of a partially linear quantile regression model. Econometric Theory 19, 1–31.Google Scholar
Lee, S. (2007) Endogeneity in quantile regression models: A control function approach. Journal of Econometrics 141, 1131–1158.Google Scholar
Li, Q. & Racine, J.S. (2007) Nonparametric Econometrics: Theory and Practice. Princeton University Press.Google Scholar
Linton, O., Sperlich, S., & Van Keilegom, I. (2008) Estimation of a semiparametric transformation model. Annals of Statistics 36, 686–718.Google Scholar
Lu, W. & Zhang, H. (2010) On estimation of partially linear transformation models. Journal of the American Statistical Association 105, 683–691.Google Scholar
Ma, S. & Kosorok, M.R. (2005) Penalized log likelihood estimation for partly linear transformation models with current status data. Annals of Statistics 33, 2256–2290.Google Scholar
Manski, C.F. (1985) Semiparametric analysis of discrete response: Asymptotic properties of the maximum score estimator. Journal of Econometrics 27, 313–333.Google Scholar
Marron, J. (1994) Visual understanding of higher-order kernels. Journal of Computational and Graphical Statistics 3, 447–458.Google Scholar
Meyer, B. (1990) Unemployment insurance and unemployment spells. Econometrica 58, 757–782.Google Scholar
Newey, W. & McFadden, D. (1994) Large sample estimation and hypothesis testing. In Engle, R.F. & McFadden, D.L. (eds.), Handbook of Econometrics, vol. 4, pp. 2111–2245. Elsevier.Google Scholar
Newey, W., Powell, J.L., & Vella, F. (1999) Nonparametric estimation of triangular simultaneous equations models. Econometrica 67, 565–603.Google Scholar
Pollard, D. (1995) Uniform ratio limit theorems for empirical processes. Scandinavian Journal of Statistics 22, 271–278.Google Scholar
Powell, J.L., Stock, J.H., & Stoker, T.M. (1989) Semiparametric estimation of weighted average derivatives. Econometrica 57, 1403–1430.Google Scholar
Shin, Y. (2010) Local rank estimation of transformation models with functional coefficients. Econometric Theory 26, 1807–1819.CrossRefGoogle Scholar
Silverman, B.W. (1986) Density Estimation for Statistics and Data Analysis. Chapman and Hall.Google Scholar
Van den Berg, G.J. (2001) Duration models: Specification, identification and multiple durations. In Heckman, J.J. & Leamer, E. (eds.), Handbook of Econometrics, vol. 5. North-Holland.Google Scholar