No CrossRef data available.
Published online by Cambridge University Press: 17 January 2013
1. The symbol 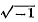 is called an impossible or imaginary quantity, because, in analogy with the received laws of algebraic symbolism, it must mean such a quantity as, being multiplied into itself, gives for a product –1. Assuming, then, that every quantity must be either plus or minus, it follows that the square of every real quantity must be plus; and hence
is called an impossible or imaginary quantity, because, in analogy with the received laws of algebraic symbolism, it must mean such a quantity as, being multiplied into itself, gives for a product –1. Assuming, then, that every quantity must be either plus or minus, it follows that the square of every real quantity must be plus; and hence 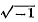 , which gives its square minus, is called an imaginary or impossible quantity.
, which gives its square minus, is called an imaginary or impossible quantity.
page 348 note * This appears to be the view taken by Sir W. Hamilton, in the first of his series of papers on Symbolical Geometry, printed in the Cambridge and Dublin Mathematical Journal. He there says, “This symbolic sum of lines represents the total (or final) effect of all those successive rectilineal motions, or translations in space, which are represented by the several summands.”