Hostname: page-component-78c5997874-v9fdk Total loading time: 0 Render date: 2024-11-03T04:12:39.122Z Has data issue: false hasContentIssue false
  • English
  • Français

Trihyperkähler reduction and instanton bundles on $\mathbb{C}\mathbb{P}^{3}$

Part of: Families, fibrations Cycles and subschemes Global differential geometry

Published online by Cambridge University Press:  27 August 2014

Marcos Jardim  and
Misha Verbitsky
Marcos Jardim
Affiliation:
IMECC - UNICAMP, Departamento de Matemática, Rua Sérgio Buarque de Holanda, 651, 13083-859 Campinas, SP, Brazil email [email protected]
Misha Verbitsky
Affiliation:
Laboratory of Algebraic Geometry, Faculty of Mathematics, NRU HSE, 7 Vavilova Street, Moscow, Russia Institute for the Physics and Mathematics of the Universe, University of Tokyo, 5-1-5 Kashiwanoha, Kashiwa, 277-8583, Japan email [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

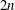A trisymplectic structure on a complex $2n$-manifold is a three-dimensional space ${\rm\Omega}$ of closed holomorphic forms such that any element of 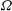${\rm\Omega}$ has constant rank $2n$, $n$ or zero, and degenerate forms in 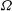${\rm\Omega}$ belong to a non-degenerate quadric hypersurface. We show that a trisymplectic manifold is equipped with a holomorphic 3-web and the Chern connection of this 3-web is holomorphic, torsion-free, and preserves the three symplectic forms. We construct a trisymplectic structure on the moduli of regular rational curves in the twistor space of a hyperkähler manifold, and define a trisymplectic reduction of a trisymplectic manifold, which is a complexified form of a hyperkähler reduction. We prove that the trisymplectic reduction in the space of regular rational curves on the twistor space of a hyperkähler manifold 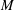$M$ is compatible with the hyperkähler reduction on 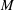$M$. As an application of these geometric ideas, we consider the ADHM construction of instantons and show that the moduli space of rank $r$, charge $c$ framed instanton bundles on 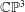$\mathbb{C}\mathbb{P}^{3}$ is a smooth trisymplectic manifold of complex dimension 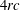$4rc$. In particular, it follows that the moduli space of rank two, charge $c$ instanton bundles on 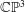$\mathbb{C}\mathbb{P}^{3}$ is a smooth complex manifold dimension 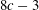$8c-3$, thus settling part of a 30-year-old conjecture.

Keywords

mathematical instantonhyperkähler reductionstable bundletwistor spacehyperkähler manifoldspacial holonomy

MSC classification

Primary: 14C21: Pencils, nets, webs
Secondary: 14D20: Algebraic moduli problems, moduli of vector bundles 53C26: Hyper-Kähler and quaternionic Kähler geometry, “special” geometry 53C28: Twistor methods
Type
Research Article
Information
Compositio Mathematica , Volume 150 , Issue 11 , November 2014 , pp. 1836 - 1868
Copyright
© The Author(s) 2014 

References

Andrada, A. and Dotti, I. G., Double products and hypersymplectic structures on ℝ4n, Comm. Math. Phys. 262 (2006), 1–16.CrossRefGoogle Scholar
Atiyah, M. F., Drinfel’d, V. G. , Hitchin, N. J. and Manin, Yu. I., Construction of instantons, Phys. Lett. A 65 (1978), 185–187.CrossRefGoogle Scholar
Arnold, V. I., The Lagrangian Grassmannian of a quaternion hypersymplectic space, Funct. Anal. Appl. 35 (2001), 61–63.Google Scholar
Atiyah, M. F., Complex analytic connections in fibre bundles, Trans. Amer. Math. Soc. 85 (1957), 181–207.CrossRefGoogle Scholar
Barth, W., Some properties of stable rank-2 vector bundles on Pn, Math. Ann. 226 (1977), 125–150.Google Scholar
Barth, W., Irreducibility of the space of mathematical instanton bundles with rank 2 and c 2= 4, Math. Ann. 258 (1981/82), 81–106.Google Scholar
Beauville, A., Varietes Kähleriennes dont la première classe de Chern est nulle, J. Differential Geom. 18 (1983), 755–782.Google Scholar
Besse, A., Einstein manifolds (Springer, New York, 1987).Google Scholar
Coandă, I., Tikhomirov, A. S. and Trautmann, G. , Irreducibility and smoothness of the moduli space of mathematical 5-instantons over ℙ3, Internat. J. Math. 14 (2003), 1–45.CrossRefGoogle Scholar
Dancer, A. and Swann, A. , Hypersymplectic manifolds, in Recent developments in pseudo-Riemannian geometry, ESI Lectures in Mathematics and Physics (European Mathematical Society, Zürich, 2008), 97–111.Google Scholar
Donaldson, S., Instantons and geometric invariant theory, Comm. Math. Phys. 93 (1984), 453–460.Google Scholar
Ellingsrud, G. and Stromme, S. A., Stable rank 2 vector bundles on ℙ3 with c 1= 0 and c 2= 3, Math. Ann. 255 (1981), 123–135.Google Scholar
Feix, B., Hyperkähler metrics on cotangent bundles, J. Reine Angew. Math. 532 (2001), 33–46.Google Scholar
Frenkel, I. B. and Jardim, M. , Complex ADHM equations, and sheaves on ℙ3, J. Algebra 319 (2008), 2913–2937.CrossRefGoogle Scholar
Grauert, H., On Levi’s problem and the imbedding of real-analytic manifolds, Ann. of Math. (2) 68 (1958), 460–473.CrossRefGoogle Scholar
Hartshorne, R., Stable vector bundles of rank 2 on ℙ3, Math. Ann. 238 (1978), 229–280.CrossRefGoogle Scholar
Hauzer, M. and Langer, A. , Moduli spaces of framed perverse instantons on ℙ3, Glasg. Math. J. 53 (2011), 51–96.CrossRefGoogle Scholar
Henni, A. A., Jardim, M. and Martins, R. V., ADHM construction of perverse instanton sheaves, Glasg. Math. J., to appear, Preprint (2012), arXiv:1201:5657.Google Scholar
Hitchin, N. J., Karlhede, A. , Lindström, U. and Roček, M. , Hyperkähler metrics and supersymmetry, Comm. Math. Phys. 108 (1987), 535–589.Google Scholar
Huybrechts, D., Complex geometry: an introduction, Universitext (Springer, Berlin, 2005).Google Scholar
Jardim, M., Instanton sheaves on complex projective spaces, Collect. Math. 57 (2006), 69–91.Google Scholar
Jardim, M., Atiyah–Drinfeld–Hitchin–Manin construction of framed instanton sheaves, C. R. Acad. Sci. Paris, Ser. I 346 (2008), 427–430.Google Scholar
Jardim, M. and Verbitsky, M. , Moduli spaces of framed instanton bundles on ℂℙ3 and twistor sections of moduli spaces of instantons on ℝ4, Adv. Math. 227 (2011), 1526–1538.CrossRefGoogle Scholar
Kaledin, D., Kaledin, D. and Verbitsky, M., Hyperkähler structures on total spaces of holomorphic cotangent bundles, in Hyperkähler manifolds (International Press, Boston, 2001).Google Scholar
Kaledin, D. and Verbitsky, M. , Non-Hermitian Yang–Mills connections, Selecta Math. (N.S.) 4 (1998), 279–320.Google Scholar
Maciocia, A., Metrics on the moduli spaces of instantons over Euclidean 4-space, Comm. Math. Phys. 135 (1991), 467–482.Google Scholar
Markushevich, D. and Tikhomirov, A. S. , Rationality of instanton moduli, Preprint (2010),arXiv:1012.4132.Google Scholar
Maruyama, M., The Theorem of Grauert–Mülich–Spindler, Math. Ann. 255 (1981), 317–333.Google Scholar
Mumford, D., Fogarty, J. and Kirwan, F., Geometric invariant theory, third edition, Ergebnisse der Mathematik und ihrer Grenzgebiete, vol. 34 (Springer, Berlin, 1994).Google Scholar
Nakajima, H., Lectures on Hilbert schemes of points on surfaces (American Mathematical Society, Providence, RI, 1999).CrossRefGoogle Scholar
Le Potier, J., Sur l’espace de modules des fibrés de Yang et Mills, in Mathematics and physics, Progress in Mathematics, vol. 37 (Birkhäuser, Boston, MA, 1983), 65–137.Google Scholar
Salamon, S., Quaternionic Kähler manifolds, Invent. Math. 67 (1982), 143–171.Google Scholar
Tikhomirov, A. S., Moduli of mathematical instanton vector bundles with odd c 2on projective space, Izv. Math. 76 (2012), 991–1073.CrossRefGoogle Scholar
Verbitsky, M., Hypercomplex Varieties, Comm. Anal. Geom. 7(2) (1999), 355–396.Google Scholar