Hostname: page-component-78c5997874-xbtfd Total loading time: 0 Render date: 2024-11-04T21:31:24.520Z Has data issue: false hasContentIssue false
  • English
  • Français

Symmetric operations for all primes and Steenrod operations in algebraic cobordism

Part of: Differential topology $K$-theory in geometry Homology and cohomology theories

Published online by Cambridge University Press:  22 December 2015

Alexander Vishik
Alexander Vishik*
Affiliation:
School of Mathematical Sciences, University of Nottingham, Nottingham NG7 2RD, UK email [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In this article we construct symmetric operations for all primes (previously known only for 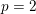$p=2$). These unstable operations are more subtle than the Landweber–Novikov operations, and encode all 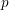$p$-primary divisibilities of characteristic numbers. Thus, taken together (for all primes) they plug the gap left by the Hurewitz map 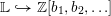$\mathbb{L}{\hookrightarrow}\mathbb{Z}[b_{1},b_{2},\ldots ]$, providing an important structure on algebraic cobordism. Applications include questions of rationality of Chow group elements, and the structure of the algebraic cobordism. We also construct Steenrod operations of tom Dieck style in algebraic cobordism. These unstable multiplicative operations are more canonical and subtle than Quillen-style operations, and complement the latter.

Keywords

algebraic cobordismsymmetric operationsSteenrod operations

MSC classification

Primary: 19E15: Algebraic cycles and motivic cohomology 55N22: Bordism and cobordism theories, formal group laws 57R77: Complex cobordism (${rm U}$- and ${rm SU}$-cobordism)
Type
Research Article
Information
Compositio Mathematica , Volume 152 , Issue 5 , May 2016 , pp. 1052 - 1070
Copyright
© The Author 2015 

References

Brosnan, P., Steenrod operations in Chow theory, Trans. Amer. Math. Soc. 355 (2003), 1869–1903.Google Scholar
tom Dieck, T., Steenrod-Operationen in Kobordismen-Theorien, Math. Z. 107 (1968), 380–401.Google Scholar
Kashiwabara, T., Hopf rings and unstable operations, J. Pure Appl. Algebra 94 (1994), 183–193.Google Scholar
Levine, M. and Morel, F., Algebraic cobordism, Springer Monographs in Mathematics (Springer, 2007).Google Scholar
Levine, M. and Pandharipande, R., Algebraic cobordism revisited, Invent. Math. 176 (2009), 63–130.Google Scholar
Panin, I., Oriented cohomology theories of algebraic varieties, J. K-theory 30 (2003), 265–314.Google Scholar
Panin, I. and Smirnov, A., Push-forwards in oriented cohomology theories of algebraic varieties, K-theory, preprint archive, 459 (2000): http://www.math.uiuc.edu/K-theory/0459/.Google Scholar
Quillen, D., Elementary proofs of some results in cobordism theory using Steenrod operations, Adv. Math. 7 (1971), 29–56.Google Scholar
Rost, M., Notes on the degree formula, Preprint (2001), available at:http://www.math.uni-bielefeld.de/∼rost/degree-formula.html.Google Scholar
Smirnov, A., Orientations and transfers in cohomology of algebraic varieties, St. Petersburg Math. J. 18 (2007), 305–346.Google Scholar
Vishik, A., Symmetric operations, Proc. Steklov Inst. Math. 246 (2004), 79–92.Google Scholar
Vishik, A., Symmetric operations in algebraic cobordism, Adv. Math. 213 (2007), 489–552.CrossRefGoogle Scholar
Vishik, A., Generic points of quadrics and Chow groups, Manuscripta Math. 122 (2007), 365–374.Google Scholar
Vishik, A., Stable and unstable operations in algebraic cobordism, Preprint (2012),arXiv:1209.5793 [math.AG].Google Scholar
Vishik, A., Operations and poly-operations in algebraic cobordism, Preprint (2014),arXiv:1409.0741 [math.AG].Google Scholar
Vishik, A., Algebraic cobordism as a module over the Lazard ring, Math. Ann. 363 (2015), 973–983; doi:10.1007/s00208-015-1190-3.Google Scholar
Voevodsky, V., Reduced power operations in motivic cohomology, Publ. Math. Inst. Hautes Études Sci. 98 (2003), 1–57.CrossRefGoogle Scholar
Wilson, W. S., Brown–Peterson homology: an introduction and sampler, Conference Board of the Mathematical Sciences, Regional Conference Series in Mathematics, vol. 48 (American Mathematical Society, Providence, RI, 1980), 1–86.Google Scholar