No CrossRef data available.
Published online by Cambridge University Press: 27 March 2023
We consider rational representations of a connected linear algebraic group 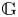 $\mathbb {G}$ over a field
$\mathbb {G}$ over a field  $k$ of positive characteristic
$k$ of positive characteristic 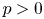 $p > 0$. We introduce a natural extension
$p > 0$. We introduce a natural extension 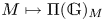 $M \mapsto \Pi (\mathbb {G})_M$ to
$M \mapsto \Pi (\mathbb {G})_M$ to 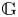 $\mathbb {G}$-modules of the
$\mathbb {G}$-modules of the  $\pi$-point support theory for modules
$\pi$-point support theory for modules 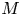 $M$ for a finite group scheme
$M$ for a finite group scheme 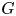 $G$ and show that this theory is essentially equivalent to the more ‘intrinsic’ and ‘explicit’ theory
$G$ and show that this theory is essentially equivalent to the more ‘intrinsic’ and ‘explicit’ theory 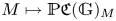 $M \mapsto \mathbb {P}\mathfrak{C}(\mathbb {G})_M$ of supports for an algebraic group of exponential type, a theory which uses
$M \mapsto \mathbb {P}\mathfrak{C}(\mathbb {G})_M$ of supports for an algebraic group of exponential type, a theory which uses 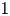 $1$-parameter subgroups
$1$-parameter subgroups 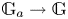 $\mathbb {G}_a \to \mathbb {G}$. We extend our support theory to bounded complexes of
$\mathbb {G}_a \to \mathbb {G}$. We extend our support theory to bounded complexes of  $\mathbb {G}$-modules,
$\mathbb {G}$-modules, 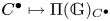 $C^\bullet \mapsto \Pi (\mathbb {G})_{C^\bullet }$. We introduce the tensor triangulated category
$C^\bullet \mapsto \Pi (\mathbb {G})_{C^\bullet }$. We introduce the tensor triangulated category  $\mathit {StMod}(\mathbb {G})$, the Verdier quotient of the bounded derived category
$\mathit {StMod}(\mathbb {G})$, the Verdier quotient of the bounded derived category 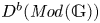 $D^b(\mathit {Mod}(\mathbb {G}))$ by the thick subcategory of mock injective modules. Our support theory satisfies all the ‘standard properties’ for a theory of supports for
$D^b(\mathit {Mod}(\mathbb {G}))$ by the thick subcategory of mock injective modules. Our support theory satisfies all the ‘standard properties’ for a theory of supports for 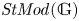 $\mathit {StMod}(\mathbb {G})$. As an application, we employ
$\mathit {StMod}(\mathbb {G})$. As an application, we employ 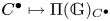 $C^\bullet \mapsto \Pi (\mathbb {G})_{C^\bullet }$ to establish the classification of
$C^\bullet \mapsto \Pi (\mathbb {G})_{C^\bullet }$ to establish the classification of 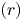 $(r)$-complete, thick tensor ideals of
$(r)$-complete, thick tensor ideals of 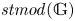 $\mathit {stmod}(\mathbb {G})$ in terms of locally
$\mathit {stmod}(\mathbb {G})$ in terms of locally 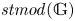 $\mathit {stmod}(\mathbb {G})$-realizable subsets of
$\mathit {stmod}(\mathbb {G})$-realizable subsets of 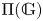 $\Pi (\mathbb {G})$ and the classification of
$\Pi (\mathbb {G})$ and the classification of 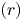 $(r)$-complete, localizing subcategories of
$(r)$-complete, localizing subcategories of  $\mathit {StMod}(\mathbb {G})$ in terms of locally
$\mathit {StMod}(\mathbb {G})$ in terms of locally 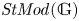 $\mathit {StMod}(\mathbb {G})$-realizable subsets of
$\mathit {StMod}(\mathbb {G})$-realizable subsets of 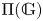 $\Pi (\mathbb {G})$.
$\Pi (\mathbb {G})$.
Dedicated to the memory of Brian Parshall
The author was partially supported by the Simons Foundation.