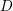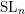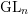Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
de Cataldo, Mark Andrea A.
Heinloth, Jochen
and
Migliorini, Luca
2021.
A support theorem for\break the Hitchin fibration: The case of GL
n
and K
C
.
Journal für die reine und angewandte Mathematik (Crelles Journal),
Vol. 2021,
Issue. 780,
p.
41.
Maulik, Davesh
and
Shen, Junliang
2021.
Endoscopic decompositions and the Hausel–Thaddeus conjecture.
Forum of Mathematics, Pi,
Vol. 9,
Issue. ,
de Cataldo, Mark Andrea A.
Rapagnetta, Antonio
and
Saccà, Giulia
2021.
The Hodge numbers of O'Grady 10 via Ngô strings.
Journal de Mathématiques Pures et Appliquées,
Vol. 156,
Issue. ,
p.
125.
Maulik, Davesh
and
Shen, Junliang
2022.
On the intersection cohomology of the moduli of SLn$\mathrm{SL}_n$‐Higgs bundles on a curve.
Journal of Topology,
Vol. 15,
Issue. 3,
p.
1034.
Li, Li
2023.
Nakajima's quiver varieties and triangular bases of rank-2 cluster algebras.
Journal of Algebra,
Vol. 634,
Issue. ,
p.
97.
Franco, Emilio
and
Peón‐Nieto, Ana
2023.
Branes on the singular locus of the Hitchin system via Borel and other parabolic subgroups.
Mathematische Nachrichten,
Vol. 296,
Issue. 5,
p.
1803.
Maulik, Davesh
and
Shen, Junliang
2023.
Cohomological χ–independence for moduli of
one-dimensional sheaves and moduli of Higgs bundles.
Geometry & Topology,
Vol. 27,
Issue. 4,
p.
1539.
Wu, Ben
2024.
Hodge numbers of O’Grady 6 via Ngô strings.
manuscripta mathematica,
Vol. 174,
Issue. 3-4,
p.
1015.
de Cataldo, Mark Andrea
Herrero, Andres Fernandez
and
Zhang, Siqing
2024.
Geometry of the logarithmic Hodge moduli space.
Journal of the London Mathematical Society,
Vol. 109,
Issue. 1,
Ruan, Yongbin
and
Shu, Cheng
2025.
Mirror of orbifold singularities in the Hitchin fibration: The case (SL ,PGL ).
Advances in Mathematics,
Vol. 461,
Issue. ,
p.
110076.