Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-23T03:29:46.419Z Has data issue: false hasContentIssue false
  • English
  • Français

Stable homotopy refinement of quantum annular homology

Part of: Homotopy theory

Published online by Cambridge University Press:  08 April 2021

Rostislav Akhmechet ,
Vyacheslav Krushkal  and
Michael Willis
Rostislav Akhmechet
Affiliation:
Department of Mathematics, University of Virginia, Charlottesville, VA22904-4137, [email protected]
Vyacheslav Krushkal
Affiliation:
Department of Mathematics, University of Virginia, Charlottesville, VA22904-4137, [email protected]
Michael Willis
Affiliation:
Department of Mathematics, University of California, Los Angeles, CA90095, [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We construct a stable homotopy refinement of quantum annular homology, a link homology theory introduced by Beliakova, Putyra and Wehrli. For each $r\geq ~2$ we associate to an annular link 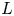$L$ a naive 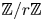$\mathbb {Z}/r\mathbb {Z}$-equivariant spectrum whose cohomology is isomorphic to the quantum annular homology of 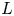$L$ as modules over 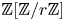$\mathbb {Z}[\mathbb {Z}/r\mathbb {Z}]$. The construction relies on an equivariant version of the Burnside category approach of Lawson, Lipshitz and Sarkar. The quotient under the cyclic group action is shown to recover the stable homotopy refinement of annular Khovanov homology. We study spectrum level lifts of structural properties of quantum annular homology.

Keywords

quantum annular homologyannular linksequivariant stable homotopy typeequivariant Burnside category

MSC classification

Secondary: 55P42: Stable homotopy theory, spectra 55P91: Equivariant homotopy theory
Type
Research Article
Information
Compositio Mathematica , Volume 157 , Issue 4 , April 2021 , pp. 710 - 769
Check for updates
Copyright
© The Author(s) 2021

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

R.A. was supported by NSF RTG Grant DMS-1839968, V.K. was supported by NSF Grant DMS-1612159, and M.W. was supported by NSF FRG Grant DMS-1563615.

References

Asaeda, M., Przytycki, J. H. and Sikora, A., Categorification of the Kauffman bracket skein module of $I$-bundles over surfaces, Algebr. Geom. Topol. 4 (2004), 1177–1210.CrossRefGoogle Scholar
Bar-Natan, D., Khovanov homology for tangles and cobordisms, Geom. Topol. 9 (2005), 1443–1499.CrossRefGoogle Scholar
Beliakova, A., Putyra, K. and Wehrli, S., Quantum link homology via trace functor I, Invent. Math. 215 (2019), 383–492.CrossRefGoogle Scholar
Boerner, J., A homology theory for framed links in I-bundles using embedded surfaces, Topology Appl. 156 (2008), 375–391.CrossRefGoogle Scholar
Borodzik, M., Politarczyk, W. and Silvero, M., Khovanov homotopy type, periodic links and localizations, Preprint (2018), arXiv:1807.08795.Google Scholar
Chen, Y. and Khovanov, M., An invariant of tangle cobordisms via subquotients of arc rings, Fund. Math. 225 (2014), 23–44.CrossRefGoogle Scholar
Cohen, R. L., Jones, J. D. S. and Segal, G. B., Floer's infinite-dimensional Morse theory and homotopy theory, in The Floer memorial volume, Progress in Mathematics, vol. 133 (Birkhäuser, Basel, 1995), 297–325.CrossRefGoogle Scholar
Grigsby, J. E., Licata, T. A. and Wehrli, S. M., Annular Khovanov homology and knotted Schur–Weyl representations, Compos. Math. 154 (2018), 459–502.CrossRefGoogle Scholar
Hu, P., Kriz, D. and Kriz, I., Field theories, stable homotopy theory, and Khovanov homology, Topology Proc. 48 (2016), 327–360.Google Scholar
Hu, P., Kriz, I. and Somberg, P., Derived representation theory of Lie algebras and stable homotopy categorification of $\mathfrak {sl}_k$, Adv. Math. 341 (2019), 367–439.CrossRefGoogle Scholar
Jacobsson, M., An invariant of link cobordisms from Khovanov homology, Algebr. Geom. Topol. 4 (2004), 1211–1251; MR2113903.CrossRefGoogle Scholar
Jones, D., Lobb, A. and Schütz, D., An $\mathfrak {sl}_n$ stable homotopy type for matched diagrams, Adv. Math. 356 (2019), 106816.CrossRefGoogle Scholar
Khovanov, M., A categorification of the Jones polynomial, Duke Math. J. 101 (2000), 359–426.CrossRefGoogle Scholar
Kitchloo, N., Symmetry breaking and link homologies I, Preprint (2019), arXiv:1910.07443.Google Scholar
Lawson, T., Lipshitz, R. and Sarkar, S., The cube and the Burnside category, in Categorification in geometry, topology, and physics, Contemporary Mathematics, vol. 684 (American Mathematical Society, Providence, RI, 2017), 63–85.CrossRefGoogle Scholar
Lawson, T., Lipshitz, R. and Sarkar, S., Khovanov spectra for tangles, Preprint (2017), arXiv:1706.02346.Google Scholar
Lawson, T., Lipshitz, R. and Sarkar, S., Chen–Khovanov spectra for tangles, Preprint (2019), arXiv:1909.12994.Google Scholar
Lawson, T., Lipshitz, R. and Sarkar, S., Khovanov homotopy type, Burnside category, and products, Geom. Topol. 24 (2020), 623–745.CrossRefGoogle Scholar
Lipshitz, R. and Sarkar, S., A Khovanov homotopy type, J. Amer. Math. Soc. 27 (2014), 983–1042.CrossRefGoogle Scholar
Lipshitz, R. and Sarkar, S., A refinement of Rasmussen's s-invariant, Duke Math. J. 163 (2014), 923–952; MR3189434.CrossRefGoogle Scholar
Musyt, J., Equivariant Khovanov homotopy type and periodic links, PhD Thesis, University of Oregon (2019), https://scholarsbank.uoregon.edu/xmlui/handle/1794/24956.Google Scholar
Roberts, L., On knot Floer homology in double branched covers, Geom. Topol. 17 (2013), 413–467.CrossRefGoogle Scholar
Sarkar, S., Scaduto, C. and Stoffregen, M., An odd Khovanov homotopy type, Adv. Math. 367 (2020), 107112.CrossRefGoogle Scholar
Stoffregen, M. and Zhang, M., Localization in Khovanov homology, Preprint (2018), arXiv:1810.04769.Google Scholar
Vogt, R. M., Homotopy limits and colimits, Math. Z. 134 (1973), 11–52.CrossRefGoogle Scholar