Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Beheshti, Roya
2014.
Hypersurfaces with too many rational curves.
Mathematische Annalen,
Vol. 360,
Issue. 3-4,
p.
753.
Browning, Timothy
and
Vishe, Pankaj
2017.
Rational curves on smooth hypersurfaces of low degree.
Algebra & Number Theory,
Vol. 11,
Issue. 7,
p.
1657.
Tseng, Dennis
2019.
A Note on Rational Curves on General Fano Hypersurfaces.
Michigan Mathematical Journal,
Vol. 68,
Issue. 4,
Lehmann, Brian
and
Tanimoto, Sho
2019.
On exceptional sets in Manin’s conjecture.
Research in the Mathematical Sciences,
Vol. 6,
Issue. 1,
Lehmann, Brian
and
Tanimoto, Sho
2019.
Geometric Manin’s conjecture and rational curves.
Compositio Mathematica,
Vol. 155,
Issue. 5,
p.
833.
Riedl, Eric
and
Yang, David
2019.
Kontsevich spaces of rational curves on Fano hypersurfaces.
Journal für die reine und angewandte Mathematik (Crelles Journal),
Vol. 2019,
Issue. 748,
p.
207.
Tseng, Dennis
2020.
Collections of Hypersurfaces Containing a Curve.
International Mathematics Research Notices,
Vol. 2020,
Issue. 13,
p.
3927.
Pan, Xuanyu
2020.
Spaces of Low-Degree Rational Curves on Fano Complete Intersections.
International Mathematics Research Notices,
Vol. 2020,
Issue. 24,
p.
10346.
Beheshti, Roya
and
Riedl, Eric
2020.
Positivity results for spaces of rational curves.
Algebra & Number Theory,
Vol. 14,
Issue. 2,
p.
485.
Furukawa, Katsuhisa
2022.
Dimension of the space of conics on a Fano hypersurface.
Journal of Pure and Applied Algebra,
Vol. 226,
Issue. 6,
p.
106970.
Shimizu, Nobuki
and
Tanimoto, Sho
2022.
The spaces of rational curves on del Pezzo threefolds of degree one.
European Journal of Mathematics,
Vol. 8,
Issue. 1,
p.
291.
Lehmann, Brian
Sengupta, Akash Kumar
and
Tanimoto, Sho
2022.
Geometric consistency of Manin's conjecture.
Compositio Mathematica,
Vol. 158,
Issue. 6,
p.
1375.
Chung, Kiryong
2023.
Desingularization of Kontsevich’s compactification of twisted cubics in $$V_5$$.
manuscripta mathematica,
Vol. 171,
Issue. 1-2,
p.
347.
Beheshti, Roya
Lehmann, Brian
Lian, Carl
Riedl, Eric
Starr, Jason
and
Tanimoto, Sho
2024.
On the asymptotic enumerativity property for Fano manifolds.
Forum of Mathematics, Sigma,
Vol. 12,
Issue. ,
Okamura, Fumiya
2024.
The Irreducibility of the Spaces of Rational Curves on del Pezzo Manifolds.
International Mathematics Research Notices,
Vol. 2024,
Issue. 12,
p.
9893.
Lian, Carl
2025.
Asymptotic Geometric Tevelev Degrees of Hypersurfaces.
Michigan Mathematical Journal,
Vol. 75,
Issue. 1,
 $e$ on a general complete intersection of multidegree
$e$ on a general complete intersection of multidegree 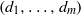 $(d_1, \ldots , d_m)$ in
$(d_1, \ldots , d_m)$ in 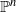 $\mathbb {P}^n$ is irreducible of the expected dimension if
$\mathbb {P}^n$ is irreducible of the expected dimension if 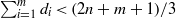 $\sum _{i=1}^m d_i \lt (2n+m+1)/3$ and
$\sum _{i=1}^m d_i \lt (2n+m+1)/3$ and  $n$ is sufficiently large. This generalizes a result of Harris, Roth and Starr [Rational curves on hypersurfaces of low degree, J. Reine Angew. Math. 571 (2004), 73–106], and is achieved by proving that the space of conics passing through any point of a general complete intersection has constant dimension if
$n$ is sufficiently large. This generalizes a result of Harris, Roth and Starr [Rational curves on hypersurfaces of low degree, J. Reine Angew. Math. 571 (2004), 73–106], and is achieved by proving that the space of conics passing through any point of a general complete intersection has constant dimension if 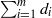 $\sum _{i=1}^m d_i$ is small compared to
$\sum _{i=1}^m d_i$ is small compared to  $n$.
$n$.

