Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Gee, Toby
2011.
On the weights of mod p Hilbert modular forms.
Inventiones mathematicae,
Vol. 184,
Issue. 1,
p.
1.
Emerton, Matthew
Gee, Toby
and
Herzig, Florian
2013.
Weight cycling and Serre-type conjectures for unitary groups.
Duke Mathematical Journal,
Vol. 162,
Issue. 9,
Diamond, Fred
and
Savitt, David
2015.
SERRE WEIGHTS FOR LOCALLY REDUCIBLE TWO-DIMENSIONAL GALOIS REPRESENTATIONS.
Journal of the Institute of Mathematics of Jussieu,
Vol. 14,
Issue. 3,
p.
639.
Chojecki, Przemysław
2015.
On non-abelian Lubin–Tate theory for .
Compositio Mathematica,
Vol. 151,
Issue. 8,
p.
1433.
Le, Daniel
Morra, Stefano
and
Park, Chol
2018.
On mod p local-global compatibility for GL3(Qp) in the non-ordinary case.
Proceedings of the London Mathematical Society,
Vol. 117,
Issue. 4,
p.
790.
Park, Chol
2021.
A classification of Breuil modules.
Israel Journal of Mathematics,
Vol. 242,
Issue. 2,
p.
605.
Hu, Yongquan
and
Wang, Haoran
2024.
On some mod p representations of quaternion algebra over ℚp.
Compositio Mathematica,
Vol. 160,
Issue. 11,
p.
2585.


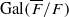 which is modular in the sense that it comes from an automorphic form on a definite quaternion algebra with centre
which is modular in the sense that it comes from an automorphic form on a definite quaternion algebra with centre 