Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Alper, Jarod
Fedorchuk, Maksym
and
Smyth, David Ishii
2017.
Second flip in the Hassett–Keel program: existence of good moduli spaces.
Compositio Mathematica,
Vol. 153,
Issue. 8,
p.
1584.
Grimes, Matthew
2018.
Compactifications of Universal Moduli Spaces of Vector Bundles and the Log-Minimal Model Program on $\overline{M}_{g}$.
International Mathematics Research Notices,
Fedorchuk, Maksym
and
Grimes, Matthew
2020.
VGIT Presentation of the Second Flip of M ¯ 2 , 1.
Michigan Mathematical Journal,
Vol. 69,
Issue. 3,
Deopurkar, Anand
and
Han, Changho
2020.
Stable log surfaces, admissible covers, and canonical curves of genus 4.
Transactions of the American Mathematical Society,
Vol. 374,
Issue. 1,
p.
589.
Johnson, Drew
and
Polishchuk, Alexander
2021.
Birational models of 𝓜2,2 arising as moduli of curves with nonspecial divisors.
Advances in Geometry,
Vol. 21,
Issue. 1,
p.
23.
Codogni, Giulio
Tasin, Luca
and
Viviani, Filippo
2021.
On some modular contractions of the moduli space of stable pointed curves.
Algebra & Number Theory,
Vol. 15,
Issue. 5,
p.
1245.
Blankers, Vance
2022.
Extremality of rational tails boundary strata in $$\pmb {{\overline{\mathscr {M}}}_{g,n}}$$.
European Journal of Mathematics,
Vol. 8,
Issue. 2,
p.
523.
Battistella, Luca
2022.
Modular compactifications of ℳ2,n with
Gorenstein curves.
Algebra & Number Theory,
Vol. 16,
Issue. 7,
p.
1547.
Battistella, Luca
and
Carocci, Francesca
2023.
A smooth compactification of the space of genus two curves in projective space: via logarithmic geometry and Gorenstein curves.
Geometry & Topology,
Vol. 27,
Issue. 3,
p.
1203.
Codogni, Giulio
Tasin, Luca
and
Viviani, Filippo
2023.
ON THE FIRST STEPS OF THE MINIMAL MODEL PROGRAM FOR THE MODULI SPACE OF STABLE POINTED CURVES.
Journal of the Institute of Mathematics of Jussieu,
Vol. 22,
Issue. 1,
p.
145.
Battistella, Luca
2024.
Gorenstein curve singularities of genus three.
ANNALI DELL'UNIVERSITA' DI FERRARA,
Vol. 70,
Issue. 3,
p.
655.
Di Lorenzo, Andrea
Pernice, Michele
and
Vistoli, Angelo
2024.
Stable cuspidal curves and the integral Chow
ring of ℳ2,1.
Geometry & Topology,
Vol. 28,
Issue. 6,
p.
2915.
Liu, Yuchen
and
Zhao, Junyan
2025.
K-moduli of Fano threefolds and genus four curves.
Journal für die reine und angewandte Mathematik (Crelles Journal),
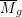 $\overline{M}_{g}$, replacing the locus of curves with a genus
$\overline{M}_{g}$, replacing the locus of curves with a genus 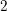 $2$ Weierstrass tail by a locus of curves with a ramphoid cusp. In this paper, for
$2$ Weierstrass tail by a locus of curves with a ramphoid cusp. In this paper, for 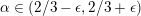 $\unicode[STIX]{x1D6FC}\in (2/3-\unicode[STIX]{x1D716},2/3+\unicode[STIX]{x1D716})$, we introduce new
$\unicode[STIX]{x1D6FC}\in (2/3-\unicode[STIX]{x1D716},2/3+\unicode[STIX]{x1D716})$, we introduce new 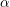 $\unicode[STIX]{x1D6FC}$-stability conditions for curves and prove that they are deformation open. This yields algebraic stacks
$\unicode[STIX]{x1D6FC}$-stability conditions for curves and prove that they are deformation open. This yields algebraic stacks 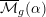 $\overline{{\mathcal{M}}}_{g}(\unicode[STIX]{x1D6FC})$ related by open immersions
$\overline{{\mathcal{M}}}_{g}(\unicode[STIX]{x1D6FC})$ related by open immersions  $\overline{{\mathcal{M}}}_{g}(2/3+\unicode[STIX]{x1D716}){\hookrightarrow}\overline{{\mathcal{M}}}_{g}(2/3){\hookleftarrow}\overline{{\mathcal{M}}}_{g}(2/3-\unicode[STIX]{x1D716})$. We prove that around a curve
$\overline{{\mathcal{M}}}_{g}(2/3+\unicode[STIX]{x1D716}){\hookrightarrow}\overline{{\mathcal{M}}}_{g}(2/3){\hookleftarrow}\overline{{\mathcal{M}}}_{g}(2/3-\unicode[STIX]{x1D716})$. We prove that around a curve  $C$ corresponding to a closed point in
$C$ corresponding to a closed point in 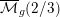 $\overline{{\mathcal{M}}}_{g}(2/3)$, these open immersions are locally modeled by variation of geometric invariant theory for the action of
$\overline{{\mathcal{M}}}_{g}(2/3)$, these open immersions are locally modeled by variation of geometric invariant theory for the action of  $\text{Aut}(C)$ on the first-order deformation space of
$\text{Aut}(C)$ on the first-order deformation space of  $C$.
$C$.