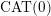Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Groves, Daniel
and
Manning, Jason Fox
2022.
Specializing cubulated relatively hyperbolic groups.
Journal of Topology,
Vol. 15,
Issue. 2,
p.
398.
Einstein, Eduard
and
Groves, Daniel
2022.
Relatively geometric actions on CAT$\operatorname{CAT}$(0) cube complexes.
Journal of the London Mathematical Society,
Vol. 105,
Issue. 1,
p.
691.
Groves, Daniel
and
Manning, Jason Fox
2023.
Hyperbolic groups acting improperly.
Geometry & Topology,
Vol. 27,
Issue. 9,
p.
3387.
Reyes, Eduardo
2023.
On cubulated relatively hyperbolic groups.
Geometry & Topology,
Vol. 27,
Issue. 2,
p.
575.
Bregman, Corey
Groves, Daniel
and
Zhu, Kejia
2024.
Relatively geometric actions of Kähler groups
on CAT(0) cube complexes.
Algebraic & Geometric Topology,
Vol. 24,
Issue. 7,
p.
4127.
Dahmani, François
Meda Satish, Suraj Krishna
and
Mutanguha, Jean Pierre
2025.
Hyperbolic hyperbolic-by-cyclic groups are cubulable.
Geometry & Topology,
Vol. 29,
Issue. 1,
p.
259.
Lafont, Jean‐François
and
Ruffoni, Lorenzo
2025.
Relative cubulation of relative strict hyperbolization.
Journal of the London Mathematical Society,
Vol. 111,
Issue. 4,