Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Lawther, R.
2014.
Sublattices generated by root differences.
Journal of Algebra,
Vol. 412,
Issue. ,
p.
255.
Guralnick, Robert M.
Lübeck, Frank
and
Yu, Jun
2016.
Rational rigidity for F4(p).
Advances in Mathematics,
Vol. 302,
Issue. ,
p.
48.
Gurevich, Shamgar
and
Howe, Roger
2018.
A look at representations of $$SL_{2}({\mathbb {F}}_{q})$$ S L 2 ( F q ) through the lens of size.
São Paulo Journal of Mathematical Sciences,
Vol. 12,
Issue. 2,
p.
252.
Korhonen, Mikko
2018.
Unipotent elements forcing irreducibility in linear algebraic groups.
Journal of Group Theory,
Vol. 21,
Issue. 3,
p.
365.
BURNESS, TIMOTHY C.
and
TESTERMAN, DONNA M.
2019.
-TYPE SUBGROUPS CONTAINING REGULAR UNIPOTENT ELEMENTS.
Forum of Mathematics, Sigma,
Vol. 7,
Issue. ,
Korhonen, Mikko
2020.
Hesselink normal forms of unipotent elements in some representations of classical groups in characteristic two.
Journal of Algebra,
Vol. 559,
Issue. ,
p.
268.
Magaard, Kay
and
Savin, Gordan
2020.
Computing finite Galois groups arising from automorphic forms.
Journal of Algebra,
Vol. 561,
Issue. ,
p.
256.
Gurevich, Shamgar
and
Howe, Roger
2020.
Rank and duality in representation theory.
Japanese Journal of Mathematics,
Vol. 15,
Issue. 2,
p.
223.
Bate, Michael
Martin, Benjamin
and
Röhrle, Gerhard
2022.
Overgroups of regular unipotent elements in reductive groups.
Forum of Mathematics, Sigma,
Vol. 10,
Issue. ,
Guralnick, Robert
and
Navarro, Gabriel
2022.
Real constituents of permutation characters.
Journal of Algebra,
Vol. 607,
Issue. ,
p.
315.
Zywina, David
2023.
The inverse Galois problem for orthogonal groups.
Transactions of the American Mathematical Society, Series B,
Vol. 10,
Issue. 33,
p.
1173.
 ${E}_{8}(p)$
${E}_{8}(p)$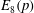 ${E}_{8}(p)$ for good primes
${E}_{8}(p)$ for good primes 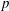 $p$ (i.e.
$p$ (i.e.  $p>5$) thereby showing that these groups occur as Galois groups over the field of rational numbers. We show that these triples arise from rigid triples in the algebraic group and prove that they generate an interesting subgroup in characteristic zero. As a byproduct of the proof, we derive a remarkable symmetry between the character table of a finite reductive group and that of its dual group. We also give a short list of possible overgroups of regular unipotent elements in simple exceptional groups.
$p>5$) thereby showing that these groups occur as Galois groups over the field of rational numbers. We show that these triples arise from rigid triples in the algebraic group and prove that they generate an interesting subgroup in characteristic zero. As a byproduct of the proof, we derive a remarkable symmetry between the character table of a finite reductive group and that of its dual group. We also give a short list of possible overgroups of regular unipotent elements in simple exceptional groups.