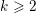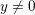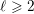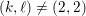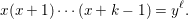Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Das, Pranabesh
Laishram, Shanta
and
Saradha, N.
2018.
VARIANTS OF ERDŐS–SELFRIDGE SUPERELLIPTIC CURVES AND THEIR RATIONAL POINTS.
Mathematika,
Vol. 64,
Issue. 2,
p.
380.
Saradha, N.
2019.
More variants of Erdős-selfridge superelliptic curves and their rational points.
Indian Journal of Pure and Applied Mathematics,
Vol. 50,
Issue. 2,
p.
333.
Edis, Sam
2019.
On a variation of the Erdős–Selfridge superelliptic curve.
Bulletin of the London Mathematical Society,
Vol. 51,
Issue. 4,
p.
633.
Dragović, Vladimir
Gontsov, Renat
and
Shramchenko, Vasilisa
2021.
Triangular Schlesinger systems and superelliptic curves.
Physica D: Nonlinear Phenomena,
Vol. 424,
Issue. ,
p.
132947.
Das, Pranabesh
Laishram, Shanta
Saradha, N.
and
Sharma, Divyum
2023.
Rational solutions to the variants of Erdős–Selfridge superelliptic curves.
International Journal of Number Theory,
Vol. 19,
Issue. 07,
p.
1707.