Published online by Cambridge University Press: 14 August 2017
Let
 $A\rightarrow B$
be a morphism of Artin local rings with the same embedding dimension. We prove that any
$A\rightarrow B$
be a morphism of Artin local rings with the same embedding dimension. We prove that any
 $A$
-flat
$A$
-flat
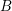 $B$
-module is
$B$
-module is
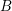 $B$
-flat. This freeness criterion was conjectured by de Smit in 1997 and improves Diamond’s criterion [The Taylor–Wiles construction and multiplicity one, Invent. Math. 128 (1997), 379–391, Theorem 2.1]. We also prove that if there is a nonzero
$B$
-flat. This freeness criterion was conjectured by de Smit in 1997 and improves Diamond’s criterion [The Taylor–Wiles construction and multiplicity one, Invent. Math. 128 (1997), 379–391, Theorem 2.1]. We also prove that if there is a nonzero
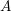 $A$
-flat
$A$
-flat
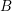 $B$
-module, then
$B$
-module, then
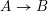 $A\rightarrow B$
is flat and is a relative complete intersection. Then we explain how this result allows one to simplify Wiles’s proof of Fermat’s last theorem: we do not need the so-called ‘Taylor–Wiles systems’ any more.
$A\rightarrow B$
is flat and is a relative complete intersection. Then we explain how this result allows one to simplify Wiles’s proof of Fermat’s last theorem: we do not need the so-called ‘Taylor–Wiles systems’ any more.