Hostname: page-component-586b7cd67f-g8jcs Total loading time: 0 Render date: 2024-11-25T09:02:56.308Z Has data issue: false hasContentIssue false
  • English
  • Français

Parabolic category O, perverse sheaves on Grassmannians, Springer fibres and Khovanov homology

Part of: Low-dimensional topology Lie algebras and Lie superalgebras Rings and algebras arising under various constructions Representation theory of groups Linear algebraic groups and related topics Special varieties

Published online by Cambridge University Press:  01 July 2009

Catharina Stroppel
Catharina Stroppel*
Affiliation:
Department of Mathematics, University of Glasgow, University Gardens, Glasgow G12 8QW, UK (email: [email protected])
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

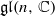For a fixed parabolic subalgebra 𝔭 of we prove that the centre of the principal block 𝒪0𝔭 of the parabolic category 𝒪 is naturally isomorphic to the cohomology ring H*(ℬ𝔭) of the corresponding Springer fibre. We give a diagrammatic description of 𝒪0𝔭 for maximal parabolic 𝔭 and give an explicit isomorphism to Braden’s description of the category PervB(G(k,n)) of Schubert-constructible perverse sheaves on Grassmannians. As a consequence Khovanov’s algebra ℋn is realised as the endomorphism ring of some object from PervB(G(n,n)) which corresponds under localisation and the Riemann–Hilbert correspondence to a full projective–injective module in the corresponding category 𝒪0𝔭. From there one can deduce that Khovanov’s tangle invariants are obtained from the more general functorial invariants in [C. Stroppel, Categorification of the Temperley Lieb category, tangles, and cobordisms via projective functors, Duke Math. J. 126(3) (2005), 547–596] by restriction.

Keywords

Khovanov homologySpringer fibreknot invariantsperverse sheavesKazhdan–Lusztig

MSC classification

Secondary: 16S99: None of the above, but in this section 17B10: Representations, algebraic theory (weights) 14M15: Grassmannians, Schubert varieties, flag manifolds 57M27: Invariants of knots and 3-manifolds 20C30: Representations of finite symmetric groups 20G05: Representation theory 14M17: Homogeneous spaces and generalizations
Type
Research Article
Information
Compositio Mathematica , Volume 145 , Issue 4 , July 2009 , pp. 954 - 992
Copyright
Copyright © Foundation Compositio Mathematica 2009

References

[1]Andersen, H. H. and Lauritzen, N., Twisted Verma modules, in Studies in Memory of Issai Schur, Progress in Mathematics, vol. 210 (Birkhäuser, Basel, 2003), 1–26.Google Scholar
[2]Andersen, H. H. and Stroppel, C., Twisting functors on 𝒪, Represent. Theory 7 (2003), 681–699.CrossRefGoogle Scholar
[3]Beilinson, A., Ginzburg, V. and Soergel, W., Koszul duality patterns in representation theory, J. Amer. Math. Soc. 9 (1996), 473–527.CrossRefGoogle Scholar
[4]Bernstein, J., Frenkel, I. and Khovanov, M., A categorification of the Temperley–Lieb algebra and Schur quotients of via projective and Zuckerman functors, Selecta Math. (N.S.) 5 (1999), 199–241.CrossRefGoogle Scholar
[5]Bernstein, J. N. and Gel’fand, S. I., Tensor products of finite- and infinite-dimensional representations of semisimple Lie algebras, Compositio Math. 41 (1980), 245–285.Google Scholar
[6]Bernstein, I. N., Gel’fand, I. M. and Gel’fand, S. I., A certain category of 𝔤-modules, Funkcional. Anal. i Priložen. 10 (1976), 1–8.Google Scholar
[7]Billey, S. C. and Warrington, G. S., Kazhdan–Lusztig polynomials for 321-hexagon-avoiding permutations, J. Algebraic Combin. 13 (2001), 111–136.CrossRefGoogle Scholar
[8]Braden, T., Perverse sheaves on Grassmannians, Canad. J. Math. 54 (2002), 493–532.CrossRefGoogle Scholar
[9]Brenti, F., Kazhdan–Lusztig and R-polynomials, Young’s lattice, and Dyck partitions, Pacific J. Math. 207 (2002), 257–286.CrossRefGoogle Scholar
[10]Brundan, J., Centers of degenerate cyclotomic Hecke algebras and parabolic category 𝒪, Preprint (2006), math.RT/0607717.Google Scholar
[11]Brundan, J., Symmetric functions, parabolic category 𝒪 and the Springer fiber, Duke Math. J. 143 (2008), 41–79, math.RT0608235.CrossRefGoogle Scholar
[12]De Concini, C. and Procesi, C., Symmetric functions, conjugacy classes and the flag variety, Invent. Math. 64 (1981), 203–219.CrossRefGoogle Scholar
[13]Fiebig, P., Centers and translation functors for the category 𝒪 over Kac–Moody algebras, Math. Z. 243 (2003), 689–717.CrossRefGoogle Scholar
[14]Fulton, W., Intersection theory, Ergebnisse der Mathematik und ihrer Grenzgebiete (3), vol. 2 2nd edition (Springer, Berlin, 1998).CrossRefGoogle Scholar
[15]Fung, F., On the topology of components of some Springer fibers and their relation to Kazhdan–Lusztig theory, Adv. Math. 178 (2003), 244–276.CrossRefGoogle Scholar
[16]Garcia, A. M. and Procesi, C., On certain graded S n modules and q-Kostka polynomials, Adv. Math 94 (1992), 83–138.Google Scholar
[17]Gordon, I., Baby Verma modules for rational Cherednik algebras, Bull. London Math. Soc. 35 (2003), 321–336.CrossRefGoogle Scholar
[18]Hotta, R. and Shimomura, N., The fixed-point subvarieties of unipotent transformations on generalized flag varieties and the Green functions, Math. Ann. 241 (1979), 193–208.CrossRefGoogle Scholar
[19]Hotta, R. and Springer, T. A., A specialization theorem for certain Weyl group representations and an application to the Green polynomials of unitary groups, Invent. Math. 41 (1977), 113–127.CrossRefGoogle Scholar
[20]Hotta, R., Takeuchi, K. and Tanisaki, T., D-modules, perverse sheaves, and representation theory, Progress in Mathematics, vol. 236 (Birkhäuser, Basel, 2008), Translated from the 1995 Japanese edition by Takeuchi.CrossRefGoogle Scholar
[21]Irving, R., Projective modules in the category 𝒪S: self-duality, Trans. Amer. Math. Soc. 291 (1985), 701–732.Google Scholar
[22]Irving, R. S. and Shelton, B., Loewy series and simple projective modules in the category 𝒪S, Pacific J. Math. 132 (1988), 319–342.CrossRefGoogle Scholar
[23]Jantzen, J. C., Einhüllende Algebren halbeinfacher Lie-Algebren, Ergebnisse der Mathematik und ihrer Grenzgebiete (3), vol. 3 (Springer, Berlin, 1983).CrossRefGoogle Scholar
[24]Khomenko, O. and Mazorchuk, V., On Arkhipov’s and Enright’s functors, Math. Z. 249 (2005), 357–386.CrossRefGoogle Scholar
[25]Khovanov, M., A categorification of the Jones polynomial, Duke Math. J. 101 (2000), 359–426.CrossRefGoogle Scholar
[26]Khovanov, M., A functor-valued invariant of tangles, Algebr. Geom. Topol. 2 (2002), 665–741 (electronic).CrossRefGoogle Scholar
[27]Khovanov, M., Crossingless matchings and the cohomology of (n,n) Springer varieties, Commun. Contemp. Math. 6 (2004), 561–577.CrossRefGoogle Scholar
[28]Khovanov, M., Mazorchuk, V. and Stroppel, C., A categorification of integral Specht modules, Proc. Amer. Math. Soc. 136 (2008), 1163–1169.CrossRefGoogle Scholar
[29]Mazorchuk, V., Ovsienko, S. and Stroppel, C., Quadratic duals, Koszul dual functors, and applications, Trans. Amer. Math. Soc. 361 (2009), 1129–1172, math.RT/0603475.CrossRefGoogle Scholar
[30]Mazorchuk, V. and Stroppel, C., Translation and shuffling of projectively presentable modules and a categorification of a parabolic Hecke module, Trans. Amer. Math. Soc. 357 (2005), 2939–2973.CrossRefGoogle Scholar
[31]Mazorchuk, V. and Stroppel, C., Projective–injective modules, Serre functors and symmetric algebras, J. Reine Angew. Math. 616 (2008), 131–165, math.RT0508119.Google Scholar
[32]Murphy, G. E., The representations of Hecke algebras of type A n, J. Algebra 173 (1995), 97–121.CrossRefGoogle Scholar
[33]Naruse, H., On an isomorphism between Specht module and left cell of S n, Tokyo J. Math. 12 (1989), 247–267.CrossRefGoogle Scholar
[34]Rickard, J., Morita theory for derived categories, J. London Math. Soc. (2) 39 (1989), 436–456.CrossRefGoogle Scholar
[35]Rickard, J., Translation functors and equivalences of derived categories for blocks of algebraic groups, in Finite-dimensional algebras and related topics (Ottawa, 1992), NATO Advanced Science Institute Series C: Mathematical and Physical Sciences, vol. 424 (Kluwer, Dordrecht, 1994), 255–264.CrossRefGoogle Scholar
[36]Rocha-Caridi, A., Splitting criteria for 𝔤-modules induced from a parabolic and the Bernstein–Gel’fand–Gel’fand resolution of a finite-dimensional, irreducible 𝔤-module, Trans. Amer. Math. Soc. 262 (1980), 335–366.Google Scholar
[37]Rouquier, R., Categorification of the braid groups, in Trends in Representation Theory of Algebras and Related Topics, Contemporary Mathematics, vol. 406 (American Mathematical Society, Providence, RI, 2006), 137–167.CrossRefGoogle Scholar
[38]Soergel, W., Kategorie 𝒪, perverse Garben und Moduln über den Koinvarianten zur Weylgruppe, J. Amer. Math. Soc. 3 (1990), 421–445.Google Scholar
[39]Soergel, W., The combinatorics of Harish-Chandra bimodules, J. Reine Angew. Math. 429 (1992), 49–74.Google Scholar
[40]Soergel, W., Kazhdan–Lusztig polynomials and a combinatorics for tilting modules, Represent. Theory 1 (1997), 83–114 (electronic).CrossRefGoogle Scholar
[41]Springer, T. A., Trigonometric sums, Green functions of finite groups and representations of Weyl groups, Invent. Math. 36 (1976), 173–207.CrossRefGoogle Scholar
[42]Springer, T. A., A construction of representations of Weyl groups, Invent. Math. 44 (1978), 279–293.CrossRefGoogle Scholar
[43]Stroppel, C., Category 𝒪: gradings and translation functors, J. Algebra 268 (2003), 301–326.CrossRefGoogle Scholar
[44]Stroppel, C., Category 𝒪: quivers and endomorphism rings of projectives, Represent. Theory 7 (2003), 322–345 (electronic).CrossRefGoogle Scholar
[45]Stroppel, C., Categorification of the Temperley–Lieb category, tangles, and cobordisms via projective functors, Duke Math. J. 126 (2005), 547–596.CrossRefGoogle Scholar
[46]Stroppel, C., TQFT with corners and tilting functors in the Kac–Moody case, Preprint (2006), math.RT/0605103.Google Scholar
[47]Tanisaki, T., Defining ideals of the closures of the conjugacy classes and representations of the Weyl groups, Tôhoku Math. J. (2) 34 (1982), 575–585.CrossRefGoogle Scholar