No CrossRef data available.
Published online by Cambridge University Press: 11 September 2024
We introduce the notions of quasi-Laurent and Laurent families of simple modules over quiver Hecke algebras of arbitrary symmetrizable types. We prove that such a family plays a similar role of a cluster in quantum cluster algebra theory and exhibits a quantum Laurent positivity phenomenon similar to the basis of the quantum unipotent coordinate ring 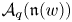 $\mathcal {A}_q(\mathfrak {n}(w))$, coming from the categorification. Then we show that the families of simple modules categorifying Geiß–Leclerc–Schröer (GLS) clusters are Laurent families by using the Poincaré–Birkhoff–Witt (PBW) decomposition vector of a simple module
$\mathcal {A}_q(\mathfrak {n}(w))$, coming from the categorification. Then we show that the families of simple modules categorifying Geiß–Leclerc–Schröer (GLS) clusters are Laurent families by using the Poincaré–Birkhoff–Witt (PBW) decomposition vector of a simple module 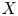 $X$ and categorical interpretation of (co)degree of
$X$ and categorical interpretation of (co)degree of 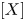 $[X]$. As applications of such
$[X]$. As applications of such 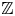 $\mathbb {Z}\mspace {1mu}$-vectors, we define several skew-symmetric pairings on arbitrary pairs of simple modules, and investigate the relationships among the pairings and
$\mathbb {Z}\mspace {1mu}$-vectors, we define several skew-symmetric pairings on arbitrary pairs of simple modules, and investigate the relationships among the pairings and  $\Lambda$-invariants of
$\Lambda$-invariants of 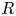 $R$-matrices in the quiver Hecke algebra theory.
$R$-matrices in the quiver Hecke algebra theory.