Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Diamond, Fred
and
Savitt, David
2015.
SERRE WEIGHTS FOR LOCALLY REDUCIBLE TWO-DIMENSIONAL GALOIS REPRESENTATIONS.
Journal of the Institute of Mathematics of Jussieu,
Vol. 14,
Issue. 3,
p.
639.
DEMBÉLÉ, LASSINA
DIAMOND, FRED
and
ROBERTS, DAVID P.
2016.
SERRE WEIGHTS AND WILD RAMIFICATION IN TWO-DIMENSIONAL GALOIS REPRESENTATIONS.
Forum of Mathematics, Sigma,
Vol. 4,
Issue. ,
Calegari, Frank
Emerton, Matthew
Gee, Toby
and
Mavrides, Lambros
2017.
Explicit Serre weights for two-dimensional Galois representations.
Compositio Mathematica,
Vol. 153,
Issue. 9,
p.
1893.
Cais, Bryden
and
Lau, Eike
2017.
Dieudonné crystals and Wach modules for p-divisible groups.
Proceedings of the London Mathematical Society,
Vol. 114,
Issue. 4,
p.
733.
Kalloniatis, Tristan
2019.
On flagged framed deformation problems of local crystalline Galois representations.
Journal of Number Theory,
Vol. 199,
Issue. ,
p.
229.


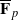 -representations of
-representations of 