Hostname: page-component-cd9895bd7-gbm5v Total loading time: 0 Render date: 2024-12-23T23:10:21.518Z Has data issue: false hasContentIssue false
  • English
  • Français

Cylinders in singular del Pezzo surfaces

Part of: Affine geometry Surfaces and higher-dimensional varieties Birational geometry Cycles and subschemes

Published online by Cambridge University Press:  21 April 2016

Ivan Cheltsov ,
Jihun Park  and
Joonyeong Won
Ivan Cheltsov
Affiliation:
School of Mathematics, The University of Edinburgh, James Clerk Maxwell Building, The King’s Buildings, Mayfield Road, Edinburgh EH9 3JZ, UK Laboratory of Algebraic Geometry, National Research University Higher School of Economics, 7 Vavilova Str., Moscow 117312, Russia email [email protected]
Jihun Park
Affiliation:
Center for Geometry and Physics, Institute for Basic Science (IBS), 77 Cheongam-ro, Nam-gu, Pohang, Gyeongbuk 37673, Korea Department of Mathematics, POSTECH, 77 Cheongam-ro, Nam-gu, Pohang, Gyeongbuk 37673, Korea email [email protected]
Joonyeong Won
Affiliation:
Algebraic Structure and its Applications Research Center, KAIST, 335 Gwahangno, Yuseong-gu, Daejeon 34143, Korea email [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

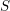For each del Pezzo surface $S$ with du Val singularities, we determine whether it admits a $(-K_{S})$-polar cylinder or not. If it allows one, then we present an effective 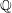$\mathbb{Q}$-divisor 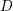$D$ that is 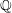$\mathbb{Q}$-linearly equivalent to 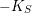$-K_{S}$ and such that the open set 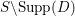$S\setminus \text{Supp}(D)$ is a cylinder. As a corollary, we classify all the del Pezzo surfaces with du Val singularities that admit non-trivial 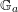$\mathbb{G}_{a}$-actions on their affine cones defined by their anticanonical divisors.

Keywords

affine coneanticanonical divisorcylinderdel Pezzo surfacedu Val singularityGa -actionminimal resolutionlog canonical singularityweak del Pezzo surface

MSC classification

Primary: 14C20: Divisors, linear systems, invertible sheaves 14E05: Rational and birational maps 14J17: Singularities 14J26: Rational and ruled surfaces 14J45: Fano varieties
Secondary: 14R20: Group actions on affine varieties 14R25: Affine fibrations
Type
Research Article
Information
Compositio Mathematica , Volume 152 , Issue 6 , June 2016 , pp. 1198 - 1224
Copyright
© The Authors 2016 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alexeev, V. and Nikulin, V., del Pezzo and K3 surfaces, MSJ Memoirs, vol. 15 (Mathematical Society of Japan, Tokyo, 2006).Google Scholar
Bruce, J. W. and Wall, C. T. C., On the classification of cubic surfaces, J. Lond. Math. Soc. (2) 19 (1979), 245–256.CrossRefGoogle Scholar
Cheltsov, I., del Pezzo surfaces and local inequalities, in Automorphisms in Birational and Affine Geometry (Levico Terme, Italy, 2012), Springer Proceedings in Mathematics and Statistics, vol. 79 (Springer, Berlin, 2014), 83–101.Google Scholar
Cheltsov, I. and Kosta, D., Computing 𝛼-invariants of singular del Pezzo surfaces, J. Geom. Anal. 24 (2014), 798–842.Google Scholar
Cheltsov, I., Park, J. and Won, J., Affine cones over smooth cubic surfaces, J. Eur. Math. Soc. (JEMS), to appear; arXiv:1303.2648.Google Scholar
Coray, D. F. and Tsfasman, M. A., Arithmetic on singular del Pezzo surfaces, Proc. Lond. Math. Soc. (3) 57 (1988), 25–87.CrossRefGoogle Scholar
Demazure, M., Surfaces de del Pezzo, in Séminaire sur les singularités des surfaces, Lecture Notes in Mathematics, vol. 777 (Springer, Berlin, 1980), 23–69.CrossRefGoogle Scholar
Derenthal, U., Singular del Pezzo surfaces whose universal torsors are hypersurfaces, Proc. Lond. Math. Soc. (3) 108 (2014), 638–681.Google Scholar
Hidaka, F. and Watanabe, K., Normal Gorenstein surfaces with ample anti-canonical divisor, Tokyo J. Math. 4 (1981), 319–330.Google Scholar
Keel, S. and Mckernan, J., Rational curves on quasi-projective surfaces, Mem. Amer. Math. Soc. 140(669) (1999).Google Scholar
Kishimoto, T., Prokhorov, Yu. and Zaidenberg, M., Group actions on affine cones, in Affine algebraic geometry, CRM Proceedings & Lecture Notes, vol. 54 (American Mathematical Society, Providence, RI, 2011), 123–163.Google Scholar
Kishimoto, T., Prokhorov, Yu. and Zaidenberg, M., Ga -actions on affine cones, Transform. Groups 18 (2013), 1137–1153.CrossRefGoogle Scholar
Kishimoto, T., Prokhorov, Yu. and Zaidenberg, M., Affine cones over Fano threefolds and additive group actions, Osaka J. Math. 51 (2014), 1093–1112.Google Scholar
Kishimoto, T., Prokhorov, Yu. and Zaidenberg, M., Unipotent group actions on del Pezzo cones, Algebr. Geom. 1 (2014), 46–56.Google Scholar
Kollár, J. and Mori, S., Birational geometry of algebraic varieties, Cambridge Tracts in Mathematics, vol. 134 (Cambridge University Press, Cambridge, 1998).CrossRefGoogle Scholar
Lazarsfeld, R., Positivity in algebraic geometry II, in Positivity for vector bundles, and multiplier ideals, Ergebnisse der Mathematik und ihrer Grenzgebiete. 3, vol. 49 (Springer, Berlin, 2004).Google Scholar
Park, J., A note on del Pezzo fibrations of degree 1, Comm. Algebra 31 (2003), 5755–5768.Google Scholar
Park, J. and Won, J., Log canonical thresholds on Gorenstein canonical del Pezzo surfaces, Proc. Edinb. Math. Soc. (2) 54 (2011), 187–219.CrossRefGoogle Scholar
Pinkham, H. C., Simple elliptic singularities, in Several complex variables, Part 1, Proceedings of Symposia in Applied Mathematics, vol. 30 (American Mathematical Society, Providence, RI, 1977), 69–70.Google Scholar
Urabe, T., On singularities on degenerate del Pezzo surfaces of degree 1, 2, in Singularities, Part 2 (Arcata, CA, 1981), Proceedings of Symposia in Applied Mathematics, vol. 40 (American Mathematical Society, Providence, RI, 1983), 587–591.Google Scholar