Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-23T23:53:06.984Z Has data issue: false hasContentIssue false
  • English
  • Français

A construction of complex analytic elliptic cohomology from double free loop spaces

Part of: Homology and cohomology theories Lie groups

Published online by Cambridge University Press:  26 July 2021

Matthew Spong
Matthew Spong*
Affiliation:
School of Mathematics and Physics, The University of Queensland, St Lucia, QLD4067, [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We construct a complex analytic version of an equivariant cohomology theory which appeared in a paper of Rezk, and which is roughly modelled on the Borel-equivariant cohomology of the double free loop space. The construction is defined on finite, torus-equivariant CW complexes and takes values in coherent holomorphic sheaves over the moduli stack of complex elliptic curves. Our methods involve an inverse limit construction over all finite-dimensional subcomplexes of the double free loop space, following an analogous construction of Kitchloo for single free loop spaces. We show that, for any given complex elliptic curve 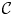$\mathcal {C}$, the fiber of our construction over $\mathcal {C}$ is isomorphic to Grojnowski's equivariant elliptic cohomology theory associated to $\mathcal {C}$.

Keywords

elliptic cohomologydouble loop spacesdouble loop groups

MSC classification

Primary: 55N34: Elliptic cohomology
Secondary: 22E67: Loop groups and related constructions, group-theoretic treatment
Type
Research Article
Information
Compositio Mathematica , Volume 157 , Issue 8 , August 2021 , pp. 1853 - 1897
Check for updates
Copyright
© 2021 The Author(s). The publishing rights in this article are licensed to Foundation Compositio Mathematica under an exclusive licence

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ando, M., Power operations in elliptic cohomology and representations of loop groups, Trans. Amer. Math. Soc. 352 (2000), 5619–5666.10.1090/S0002-9947-00-02412-0CrossRefGoogle Scholar
Atiyah, M. and Bott, R., The moment map and equivariant cohomology, Topology 23 (1984), 1–28.10.1016/0040-9383(84)90021-1CrossRefGoogle Scholar
Berwick-Evans, D. and Tripathy, A., A de Rham model for complex analytic equivariant elliptic cohomology, Adv. Math. 380 (2021), 107575.10.1016/j.aim.2021.107575CrossRefGoogle Scholar
Chen, H., Torus equivariant elliptic cohomology and sigma orientations, PhD thesis (2010), http://hdl.handle.net/2142/16822.Google Scholar
Etingof, P. I. and Frenkel, I. B., Central extensions of current groups in two dimensions, Commun. Math. Phys. 165 (1994), 429–444.10.1007/BF02099419CrossRefGoogle Scholar
Ganter, N., The elliptic Weyl character formula, Compos. Math. 150 (2014), 1196–1234.10.1112/S0010437X1300777XCrossRefGoogle Scholar
Ginzburg, V., Kapranov, M. and Vasserot, E., Elliptic algebras and equivariant elliptic cohomology, Preprint (1995), arXiv:q-alg/9505012.Google Scholar
Grojnowski, I., Delocalized equivariant elliptic cohomology, in Elliptic cohomology: geometry, applications, and higher chromatic analogues, London Mathemetical Lecture Note Series, vol. 342 (Cambridge University Press, Cambridge, 2007), 114–121.10.1017/CBO9780511721489.007CrossRefGoogle Scholar
Kitchloo, N., Quantization of the modular functor and equivariant elliptic cohomology, in Homotopy theory: tools and applications, Contemporary Mathematics, vol. 729 (American Mathematical Society, Providence, RI, 2019), 157–177.10.1090/conm/729/14694CrossRefGoogle Scholar
Lewis, L. G. Jr., May, J. P., Steinberger, M. and McClure, J. E., Equivariant stable homotopy theory, Lecture Notes in Mathematics, vol. 1213 (Springer, Berlin, 1986).10.1007/BFb0075778CrossRefGoogle Scholar
Rezk, C., Looijenga line bundles in complex analytic elliptic cohomology, Tunis. J. Math. 2 (2020), 1–42.10.2140/tunis.2020.2.1CrossRefGoogle Scholar
Rosu, I., Equivariant elliptic cohomology and rigidity, Amer. J. Math. 123 (2001), 647–677.10.1353/ajm.2001.0027CrossRefGoogle Scholar
Rosu, I. and Knutson, A., Equivariant K-theory and equivariant cohomology, Math. Z. 243 (2003), 423–448.10.1007/s00209-002-0447-1CrossRefGoogle Scholar
Spong, M., Comparison theorems for torus-equivariant elliptic cohomology theories, PhD thesis (2019), http://hdl.handle.net/11343/225560.Google Scholar
The Stacks Project Authors, Stacks Project, https://stacks.math.columbia.edu.Google Scholar