Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-23T17:59:02.288Z Has data issue: false hasContentIssue false
  • English
  • Français

Compatible systems and ramification

Part of: Arithmetic algebraic geometry Algebraic number theory: local and $p$-adic fields (Co)homology theory

Published online by Cambridge University Press:  21 October 2019

Qing Lu  and
Weizhe Zheng
Qing Lu
Affiliation:
School of Mathematical Sciences, Beijing Normal University, Beijing 100875, China School of Mathematical Sciences, University of the Chinese Academy of Sciences, Beijing 100049, China email [email protected]
Weizhe Zheng
Affiliation:
Morningside Center of Mathematics and Hua Loo-Keng Key Laboratory of Mathematics, Academy of Mathematics and Systems Science, Chinese Academy of Sciences, Beijing 100190, China University of the Chinese Academy of Sciences, Beijing 100049, China email [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We show that compatible systems of $\ell$-adic sheaves on a scheme of finite type over the ring of integers of a local field are compatible along the boundary up to stratification. This extends a theorem of Deligne on curves over a finite field. As an application, we deduce the equicharacteristic case of classical conjectures on 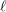$\ell$-independence for proper smooth varieties over complete discrete valuation fields. Moreover, we show that compatible systems have compatible ramification. We also prove an analogue for integrality along the boundary.

Keywords

ℓ-independenceintegralityvaluative criterionlocal fieldwild ramification

MSC classification

Primary: 14F20: Étale and other Grothendieck topologies and (co)homologies
Secondary: 11G25: Varieties over finite and local fields 11S15: Ramification and extension theory
Type
Research Article
Information
Compositio Mathematica , Volume 155 , Issue 12 , December 2019 , pp. 2334 - 2353
Copyright
© The Authors 2019 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

The first author was partially supported by National Natural Science Foundation of China Grants 11371043 and 11501541. The second author was partially supported by National Natural Science Foundation of China Grants 11621061, 11688101 and 11822110 and the National Center for Mathematics and Interdisciplinary Sciences, Chinese Academy of Sciences.

References

Chiarellotto, B. and Lazda, C., Around ℓ-independence , Compositio Math. 154 (2018), 223–248; MR 3719248.Google Scholar
Deligne, P., Les constantes des équations fonctionnelles des fonctions L , in Modular functions of one variable, II, Proc. Int. Summer School, University of Antwerp, Antwerp, 1972, Lecture Notes in Mathematics, vol. 349 (Springer, Berlin, 1973), 501–597 (in French); MR 0349635.Google Scholar
Deligne, P., La conjecture de Weil. II , Publ. Math. Inst. Hautes Études Sci. 52 (1980), 137–252 (in French); MR 601520 (83c:14017).Google Scholar
Deligne, P. and Katz, N., Groupes de monodromie en géométrie algébrique. II, Lecture Notes in Mathematics, vol. 340 (Springer, Berlin–New York, 1973) (in French). In Séminaire de Géométrie Algébrique du Bois-Marie 1967–1969 (SGA 7 II); MR 0354657.Google Scholar
Deligne, P. and Lusztig, G., Representations of reductive groups over finite fields , Ann. of Math. (2) 103 (1976), 103–161; MR 0393266.Google Scholar
Esnault, H., Deligne’s integrality theorem in unequal characteristic and rational points over finite fields , Ann. of Math. (2) 164 (2006), 715–730, with an appendix by Pierre Deligne and Esnault; MR 2247971.Google Scholar
Fujiwara, K., Independence of l for intersection cohomology (after Gabber) , in Algebraic geometry 2000, Azumino (Hotaka), Advanced Studies in Pure Mathematics, vol. 36 (Mathematical Society of Japan, Tokyo, 2002), 145–151; MR 1971515 (2004c:14038).Google Scholar
Fujiwara, K., A proof of the absolute purity conjecture (after Gabber) , in Algebraic geometry 2000, Azumino (Hotaka), Advanced Studies in Pure Mathematics, vol. 36 (Mathematical Society of Japan, Tokyo, 2002), 153–183; MR 1971516.Google Scholar
Grothendieck, A., Classes de Chern et representations linearies des groupes discrets , in Dix exposés sur la cohomologie des schémas, Advanced Studies in Pure Mathematics, vol. 3 (North-Holland, Amsterdam, 1968), 215–305 (in French); MR 265370.Google Scholar
Guo, N., Wildly compatible systems and six operations, Preprint (2018), arXiv:1801.06065.Google Scholar
Illusie, L., Théorie de Brauer et caractéristique d’Euler–Poincaré (d’après P. Deligne) , in The Euler–Poincaré characteristic, Astérisque, vol. 82 (Société Mathématique de France, Paris, 1981), 161–172 (in French); MR 629127.Google Scholar
Illusie, L., An overview of the work of K. Fujiwara, K. Kato, and C. Nakayama on logarithmic étale cohomology , Astérisque 279 (2002), 271–322. In Cohomologies $p$ -adiques et applications arithmétiques, II; MR 1922832.Google Scholar
Illusie, L., Miscellany on traces in ℓ-adic cohomology: a survey , Jpn. J. Math. 1 (2006), 107–136; MR 2261063.Google Scholar
Illusie, L. and Zheng, W., Odds and ends on finite group actions and traces , Int. Math. Res. Not. IMRN 2013 (2013), 1–62; MR 3041694.Google Scholar
Ito, T., Weight–monodromy conjecture over equal characteristic local fields , Amer. J. Math. 127 (2005), 647–658; MR 2141647.Google Scholar
de Jong, A. J., Families of curves and alterations , Ann. Inst. Fourier (Grenoble) 47 (1997), 599–621; MR 1450427 (98f:14019).Google Scholar
Laumon, G., Comparaison de caractéristiques d’Euler–Poincaré en cohomologie l-adique , C. R. Acad. Sci. Paris Sér. I Math. 292 (1981), 209–212 (in French, with English summary);MR 610321.Google Scholar
Laumon, G. and Moret-Bailly, L., Champs algébriques , in Ergebnisse der Mathematik und ihrer Grenzgebiete (3), A Series of Modern Surveys in Mathematics, vol. 39 (Springer, Berlin, 2000); MR 1771927 (2001f:14006).Google Scholar
Lu, Q. and Zheng, W., $\ell$ -independence over Henselian valuation fields, Preprint (2019),arXiv:1904.02324 (appendix to H. Kato, $\ell$ -independence of the trace of local monodromy in a relative case).Google Scholar
Ochiai, T., l-independence of the trace of monodromy , Math. Ann. 315 (1999), 321–340; MR 1715253.Google Scholar
Riou, J., Exposé XVI. Classes de Chern, morphismes de Gysin, pureté absolue , Astérisque 363–364 (2014), 301–349 (in French). In Travaux de Gabber sur l’uniformisation locale et la cohomologie étale des schémas quasi-excellents; MR 3329786.Google Scholar
Grothendieck, A., Revêtements étales et groupe fondamental (SGA 1), Documents Mathématiques (Paris), vol. 3 (Société Mathématique de France, Paris, 2003) (in French). In Séminaire de géométrie algébrique du Bois Marie 1960–1961. [Algebraic Geometry Seminar of Bois Marie 1960–1961]; directed by A. Grothendieck; with two papers by M. Raynaud; updated and annotated reprint of the 1971 original [Lecture Notes in Mathematics, vol. 224, Springer, Berlin; MR0354651 (50 #7129)]; MR 2017446.Google Scholar
Artin, M., Grothendieck, A. and Verdier, J. L. (eds), Séminaire de Géométrie Algébrique du Bois-Marie – 1963–1964 – Théorie des topos et cohomologie étale des schémas (SGA 4). Tome 3, Lecture Notes in Mathematics, vol. 305 (Springer, Berlin–New York, 1973) (in French). With the collaboration of P. Deligne and B. Saint-Donat; MR 0354654.Google Scholar
Grothendieck, A., Séminaire de Géométrie Algébrique du Bois-Marie – 1967–1969 – Groupes de monodromie en géométrie algébrique (SGA 7). Tome I, Lecture Notes in Mathematics, vol. 288 (Springer, Berlin–New York, 1972) (in French). With the collaboration of M. Raynaud and D. S. Rim; MR 0354656.Google Scholar
Saito, T. and Yatagawa, Y., Wild ramification determines the characteristic cycle , Ann. Sci. Éc. Norm. Supér. (4) 50 (2017), 1065–1079 (in English, with English and French summaries);MR 3679621.Google Scholar
Serre, J.-P., Facteurs locaux des fonctions zêta des varietés algébriques (définitions et conjectures) , in Séminaire Delange–Pisot–Poitou. 11e année: 1969/70. Théorie des nombres. Fasc. 1: Exposés 1 à 15; Fasc. 2: Exposés 16 à 24 (Secrétariat Mathématique, Paris, 1970) (in French); MR 3618526.Google Scholar
Serre, J.-P. and Tate, J., Good reduction of abelian varieties , Ann. of Math. (2) 88 (1968), 492–517; MR 0236190.Google Scholar
Terasoma, T., Monodromy weight filtration is independent of $l$ , Preprint (1998),arXiv:math/9802051.Google Scholar
Vidal, I., Théorie de Brauer et conducteur de Swan , J. Algebraic Geom. 13 (2004), 349–391 (in French, with French summary); MR 2047703.Google Scholar
Vidal, I., Courbes nodales et ramification sauvage virtuelle , Manuscripta Math. 118 (2005), 43–70 (in French, with English summary); MR 2171291.Google Scholar
Yatagawa, Y., Having the same wild ramification is preserved by the direct image , Manuscripta Math. 157 (2018), 233–246; MR 3845763.Google Scholar
Zheng, W., Sur la cohomologie des faisceaux l-adiques entiers sur les corps locaux , Bull. Soc. Math. France 136 (2008), 465–503 (in French, with English and French summaries);MR 2415350 (2009d:14015).Google Scholar
Zheng, W., Sur l’indépendance de l en cohomologie l-adique sur les corps locaux , Ann. Sci. Éc. Norm. Supér. (4) 42 (2009), 291–334 (in French, with English and French summaries);MR 2518080 (2010i:14032).Google Scholar
Zheng, W., Six operations and Lefschetz–Verdier formula for Deligne–Mumford stacks , Sci. China Math. 58 (2015), 565–632; MR 3319927.Google Scholar
Zheng, W., Companions on Artin stacks , Math. Z. 292 (2019), 57–81; MR 3968893.Google Scholar