Hostname: page-component-586b7cd67f-dlnhk Total loading time: 0 Render date: 2024-11-25T06:04:34.000Z Has data issue: false hasContentIssue false
  • English
  • Français

Rational cobordisms and integral homology

Part of: Topological manifolds Low-dimensional topology

Published online by Cambridge University Press:  29 October 2020

Paolo Aceto ,
Daniele Celoria  and
JungHwan Park
Paolo Aceto
Affiliation:
Mathematical Institute, University of Oxford, Oxford, [email protected]
Daniele Celoria
Affiliation:
Mathematical Institute, University of Oxford, Oxford, [email protected]
JungHwan Park
Affiliation:
School of Mathematics, Georgia Institute of Technology, Atlanta, GA, [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We consider the question of when a rational homology 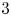$3$-sphere is rational homology cobordant to a connected sum of lens spaces. We prove that every rational homology cobordism class in the subgroup generated by lens spaces is represented by a unique connected sum of lens spaces whose first homology group injects in the first homology group of any other element in the same class. As a first consequence, we show that several natural maps to the rational homology cobordism group have infinite-rank cokernels. Further consequences include a divisibility condition between the determinants of a connected sum of $2$-bridge knots and any other knot in the same concordance class. Lastly, we use knot Floer homology combined with our main result to obstruct Dehn surgeries on knots from being rationally cobordant to lens spaces.

Keywords

lens spacesthree-dimensional homology cobordism groups

MSC classification

Primary: 57N13: Topology of $E^4$, $4$-manifolds 57M27: Invariants of knots and 3-manifolds 57N70: Cobordism and concordance 57M25: Knots and links in $S^3$
Type
Research Article
Information
Compositio Mathematica , Volume 156 , Issue 9 , September 2020 , pp. 1825 - 1845
Check for updates
Copyright
Copyright © The Author(s) 2020

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Aceto, P. and Alfieri, A., On sums of torus knots concordant to alternating knots, Bull. Lond. Math. Soc. 51 (2019), 327–343.10.1112/blms.12228CrossRefGoogle Scholar
Aceto, P. and Golla, M., Dehn surgeries and rational homology balls, Algebr. Geom. Topol. 17 (2017), 487–527.10.2140/agt.2017.17.487CrossRefGoogle Scholar
Aceto, P. and Larson, K., Knot concordance and homology sphere groups, Int. Math. Res. Not. IMRN 2018 (2018), 7318–7334.Google Scholar
Baker, K. L., Grigsby, J. E. and Hedden, M., Grid diagrams for lens spaces and combinatorial knot Floer homology, Int. Math. Res. Not. IMRN 2008 (2008), Art. ID rnm024, 39 pp.10.1093/imrn/rnn024CrossRefGoogle Scholar
Casson, A. and Gordon, C. McA., On slice knots in dimension three, in Algebraic and geometric topology (Proc. Sympos. Pure Math., Stanford University, Stanford, CA, 1976), Part 2 (American Mathematical Society, Providence, RI, 1978), 39–53.Google Scholar
Casson, A. and Gordon, C. McA., Cobordism of classical knots, in A la recherche de la topologie perdue (Birkhäuser, Boston, MA, 1986), 181–199; with an appendix by Gilmer, P. M..Google Scholar
Casson, A. J. and Harer, J. L., Some homology lens spaces which bound rational homology balls, Pacific J. Math. 96 (1981), 23–36.Google Scholar
Donaldson, S. K., The orientation of Yang–Mills moduli spaces and $4$-manifold topology, J. Differential Geom. 26 (1987), 397–428.Google Scholar
Golla, M. and Larson, K., Linear independence in the rational homology cobordism group, J. Inst. Math. Jussieu (2019), doi:10.1017/S1474748019000434.CrossRefGoogle Scholar
Gordon, C. McA., Dehn surgery and satellite knots, Trans. Amer. Math. Soc. 275 (1983), 687–708.10.1090/S0002-9947-1983-0682725-0CrossRefGoogle Scholar
Greene, J. E., The lens space realization problem, Ann. of Math. (2) 177 (2013), 449–511.10.4007/annals.2013.177.2.3CrossRefGoogle Scholar
Greene, J. E., L-space surgeries, genus bounds, and the cabling conjecture, J. Differential Geom. 100 (2015), 491–506.Google Scholar
Hedden, M., Kim, S.-G. and Livingston, C., Topologically slice knots of smooth concordance order two, J. Differential Geom. 102 (2016), 353–393.10.4310/jdg/1456754013CrossRefGoogle Scholar
Hedden, M., Livingston, C. and Ruberman, D., Topologically slice knots with nontrivial Alexander polynomial, Adv. Math. 231 (2012), 913–939.10.1016/j.aim.2012.05.019CrossRefGoogle Scholar
Hodgson, C. and Rubinstein, J. H., Involutions and isotopies of lens spaces, in Knot theory and manifolds (Vancouver, BC, 1983), Lecture Notes in Mathematics, vol. 1144 (Springer, Berlin, 1985), 60–96.Google Scholar
Hom, J. and Wu, Z., Four-ball genus bounds and a refinement of the Ozváth–Szabó tau invariant, J. Symplectic Geom. 14 (2016), 305–323.Google Scholar
Kim, S.-G. and Livingston, C., Nonsplittability of the rational homology cobordism group of 3-manifolds, Pacific J. Math. 271 (2014), 183–211.10.2140/pjm.2014.271.183CrossRefGoogle Scholar
Lisca, P., Lens spaces, rational balls and the ribbon conjecture, Geom. Topol. 11 (2007), 429–472.10.2140/gt.2007.11.429CrossRefGoogle Scholar
Lisca, P., Sums of lens spaces bounding rational balls, Algebr. Geom. Topol. 7 (2007), 2141–2164.10.2140/agt.2007.7.2141CrossRefGoogle Scholar
Litherland, R. A., Signatures of iterated torus knots, in Topology of low-dimensional manifolds (Proc. Second Sussex Conf., Chelwood Gate, 1977), Lecture Notes in Mathematics, vol. 722 (Springer, Berlin, 1979), 71–84.Google Scholar
Moser, L., Elementary surgery along a torus knot, Pacific J. Math. 38 (1971), 737–745.10.2140/pjm.1971.38.737CrossRefGoogle Scholar
Ni, Y. and Wu, Z., Cosmetic surgeries on knots in $S^{3}$, J. Reine Angew. Math. 2015 (2015), 1–17.Google Scholar
Owens, B. and Strle, S., Rational homology spheres and the four-ball genus of knots, Adv. Math. 200 (2006), 196–216.Google Scholar
Ozsváth, P. and Szabó, Z., Absolutely graded Floer homologies and intersection forms for four-manifolds with boundary, Adv. Math. 173 (2003), 179–261.10.1016/S0001-8708(02)00030-0CrossRefGoogle Scholar
Ozsváth, P. and Szabó, Z., Holomorphic disks and knot invariants, Adv. Math. 186 (2004), 58–116.10.1016/j.aim.2003.05.001CrossRefGoogle Scholar
Ozsváth, P. and Szabó, Z., On knot Floer homology and lens space surgeries, Topology 44 (2005), 1281–1300.Google Scholar
Ozsváth, P. and Szabó, Z., Knot Floer homology and rational surgeries, Algebr. Geom. Topol. 11 (2011), 1–68.10.2140/agt.2011.11.1CrossRefGoogle Scholar
Peters, T. D., Computations of Heegaard Floer homology: torus bundles, L-spaces, and correction terms, PhD thesis, Columbia University (2010).Google Scholar
Rasmussen, J. A., Floer homology and knot complements, PhD thesis, Harvard University (2003).Google Scholar
Rasmussen, J., Lens space surgeries and a conjecture of Goda and Teragaito, Geom. Topol. 8 (2004), 1013–1031.CrossRefGoogle Scholar
Rasmussen, J., Lens space surgeries and L-space homology spheres, Preprint (2007), arXiv:0710.2531.Google Scholar
Walker, K., An extension of Casson's invariant, Annals of Mathematics Studies, vol. 126 (Princeton University Press, Princeton, NJ, 1992).10.1515/9781400882465CrossRefGoogle Scholar