Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Hu, Yongquan
and
Paškūnas, Vytautas
2019.
On crystabelline deformation rings of $$\mathrm {Gal}(\overline{\mathbb {Q}}_p/\mathbb {Q}_p)$$ Gal ( Q ¯ p / Q p ) (with an appendix by Jack Shotton).
Mathematische Annalen,
Vol. 373,
Issue. 1-2,
p.
421.
Tung, Shen-Ning
2021.
On the modularity of 2-adic potentially semi-stable deformation rings.
Mathematische Zeitschrift,
Vol. 298,
Issue. 1-2,
p.
107.
Hu, Yong Quan
2021.
A Note on Integral Structures in Some Locally Algebraic Representations of GL2.
Acta Mathematica Sinica, English Series,
Vol. 37,
Issue. 1,
p.
59.
Tung, Shen-Ning
2021.
On the automorphy of 2-dimensional potentially
semistable deformation rings of Gℚp.
Algebra & Number Theory,
Vol. 15,
Issue. 9,
p.
2173.
Dotto, Andrea
and
Le, Daniel
2021.
Diagrams in the mod p cohomology of Shimura curves.
Compositio Mathematica,
Vol. 157,
Issue. 8,
p.
1653.
Gee, Toby
and
Newton, James
2022.
PATCHING AND THE COMPLETED HOMOLOGY OF LOCALLY SYMMETRIC SPACES.
Journal of the Institute of Mathematics of Jussieu,
Vol. 21,
Issue. 2,
p.
395.
Paškūnas, Vytautas
2022.
ON SOME CONSEQUENCES OF A THEOREM OF J. LUDWIG.
Journal of the Institute of Mathematics of Jussieu,
Vol. 21,
Issue. 3,
p.
1067.
Nakamura, Kentaro
2023.
Zeta morphisms for rank two universal deformations.
Inventiones mathematicae,
Vol. 234,
Issue. 1,
p.
171.
Caraiani, Ana
and
Tamiozzo, Matteo
2023.
On the étale cohomology of Hilbert modular varieties with torsion coefficients.
Compositio Mathematica,
Vol. 159,
Issue. 11,
p.
2279.
Banerjee, Debargha
and
Rai, Vivek
2024.
Towards a mod-p Lubin–Tate theory for GL2 over totally real fields.
International Journal of Number Theory,
Vol. 20,
Issue. 01,
p.
199.
Hu, Yongquan
and
Wang, Haoran
2024.
On some mod p representations of quaternion algebra over ℚp.
Compositio Mathematica,
Vol. 160,
Issue. 11,
p.
2585.
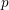 $p$ -adic Langlands program for
$p$ -adic Langlands program for 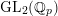 $\operatorname{GL}_{2}(\mathbb{Q}_{p})$
$\operatorname{GL}_{2}(\mathbb{Q}_{p})$ 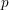 $p$
-adic local Langlands correspondence for
$p$
-adic local Langlands correspondence for
 $\operatorname{GL}_{2}(\mathbb{Q}_{p})$
via the patching method of Taylor–Wiles and Kisin. This construction sheds light on the relationship between the various other approaches to both the local and the global aspects of the
$\operatorname{GL}_{2}(\mathbb{Q}_{p})$
via the patching method of Taylor–Wiles and Kisin. This construction sheds light on the relationship between the various other approaches to both the local and the global aspects of the
 $p$
-adic Langlands program; in particular, it gives a new proof of many cases of the second author’s local–global compatibility theorem and relaxes a hypothesis on the local mod
$p$
-adic Langlands program; in particular, it gives a new proof of many cases of the second author’s local–global compatibility theorem and relaxes a hypothesis on the local mod
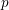 $p$
representation in that theorem.
$p$
representation in that theorem.