Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Jones, Corey
Morrison, Scott
Penneys, David
and
Plavnik, Julia
2022.
Extension Theory for Braided-Enriched Fusion Categories.
International Mathematics Research Notices,
Vol. 2022,
Issue. 20,
p.
15632.
Kalmykov, Artem
and
Safronov, Pavel
2022.
A categorical approach to dynamical quantum groups.
Forum of Mathematics, Sigma,
Vol. 10,
Issue. ,
Décoppet, Thibault D.
2023.
Rigid and separable algebras in fusion 2-categories.
Advances in Mathematics,
Vol. 419,
Issue. ,
p.
108967.
Décoppet, Thibault
2023.
Compact semisimple 2-categories.
Transactions of the American Mathematical Society,
Morrison, Scott
Walker, Kevin
and
Wedrich, Paul
2023.
Invariants of 4–manifolds from Khovanov–Rozansky
link homology.
Geometry & Topology,
Vol. 26,
Issue. 8,
p.
3367.
Negron, Cris
and
Pevtsova, Julia
2023.
Support for Integrable Hopf Algebras via Noncommutative Hypersurfaces.
International Mathematics Research Notices,
Vol. 2023,
Issue. 3,
p.
1882.
Ye, Weicheng
and
Zou, Liujun
2023.
Anomaly of $(2+1)$-dimensional symmetry-enriched topological order from $(3+1)$-dimensional topological quantum field theory.
SciPost Physics,
Vol. 15,
Issue. 1,
Huston, Peter
Burnell, Fiona
Jones, Corey
and
Penneys, David
2023.
Composing topological domain walls and anyon mobility.
SciPost Physics,
Vol. 15,
Issue. 3,
Geiko, Roman
and
Moore, Gregory W.
2024.
When Does a Three-Dimensional Chern–Simons–Witten Theory Have a Time Reversal Symmetry?.
Annales Henri Poincaré,
Vol. 25,
Issue. 1,
p.
673.
Décoppet, Thibault D.
2024.
The 2-Deligne tensor product.
Kyoto Journal of Mathematics,
Vol. 64,
Issue. 1,
Costantino, Francesco
and
Lê, Thang T. Q.
2025.
STATED SKEIN MODULES OF 3-MANIFOLDS AND TQFT.
Journal of the Institute of Mathematics of Jussieu,
p.
1.
Müller, Lukas
and
Woike, Lukas
2025.
Categorified open topological field theories.
Proceedings of the American Mathematical Society,
Décoppet, Thibault D.
2025.
Finite semisimple module 2-categories.
Selecta Mathematica,
Vol. 31,
Issue. 1,
Guu, Jin-Cheng
2025.
Quantum Symmetries.
Vol. 813,
Issue. ,
p.
303.
Costello, Kevin
and
Gwilliam, Owen
2025.
Encyclopedia of Mathematical Physics.
p.
569.
Heinrich, Sebastian
2025.
Module monoidal categories as categorification of associative algebras.
Journal of Pure and Applied Algebra,
Vol. 229,
Issue. 7,
p.
107959.
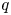 $q$.
$q$.