Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-23T16:10:00.019Z Has data issue: false hasContentIssue false
  • English
  • Français

L-spaces, taut foliations, and graph manifolds

Part of: Low-dimensional topology

Published online by Cambridge University Press:  23 January 2020

Jonathan Hanselman ,
Jacob Rasmussen ,
Sarah Dean Rasmussen  and
Liam Watson
Jonathan Hanselman
Affiliation:
Department of Mathematics, Princeton University, Fine Hall, Washington Road,Princeton, NJ08540, USA email [email protected]
Jacob Rasmussen
Affiliation:
Department of Pure Mathematics and Mathematical Statistics,Centre for Mathematical Sciences, Wilberforce Road, CambridgeCB3 0WB, UK email [email protected]
Sarah Dean Rasmussen
Affiliation:
Department of Pure Mathematics and Mathematical Statistics,Centre for Mathematical Sciences, Wilberforce Road, CambridgeCB3 0WB, UK email [email protected]
Liam Watson
Affiliation:
Department of Mathematics, University of British Columbia, 1984 Mathematics Road, Vancouver, BC, CanadaV6T 1Z2 email [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

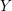If $Y$ is a closed orientable graph manifold, we show that $Y$ admits a coorientable taut foliation if and only if 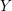$Y$ is not an L-space. Combined with previous work of Boyer and Clay, this implies that $Y$ is an L-space if and only if 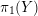$\unicode[STIX]{x1D70B}_{1}(Y)$ is not left-orderable.

Keywords

graph manifoldL-spacetaut foliationHeegaard Floer

MSC classification

Primary: 57M27: Invariants of knots and 3-manifolds
Type
Research Article
Information
Compositio Mathematica , Volume 156 , Issue 3 , March 2020 , pp. 604 - 612
Copyright
© The Authors 2020

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

The first author was partially supported by NSF RTG grant DMS-1148490. The second author was partially supported by EPSRC grant EP/M000648/1. The third author was supported by EPSRC grant EP/M000648/1. The fourth author was partially supported by a Marie Curie Career Integration Grant (HFFUNDGRP).

References

Bowden, J., Approximating C 0-foliations by contact structures, Geom. Funct. Anal. 26 (2016), 1255–1296.10.1007/s00039-016-0387-2CrossRefGoogle Scholar
Boyer, S. and Clay, A., Slope detection, foliations in graph manifolds, and L-spaces, Preprint (2015), arXiv:1510.02378.Google Scholar
Boyer, S. and Clay, A., Foliations, orders, representations, L-spaces and graph manifolds, Adv. Math. 310 (2017), 159–234.10.1016/j.aim.2017.01.026CrossRefGoogle Scholar
Boyer, S., Gordon, C. McA. and Watson, L., On L-spaces and left-orderable fundamental groups, Math. Ann. 356 (2013), 1213–1245.10.1007/s00208-012-0852-7CrossRefGoogle Scholar
Boyer, S., Rolfsen, D. and Wiest, B., Orderable 3-manifold groups, Ann. Inst. Fourier (Grenoble) 55 (2005), 243–288.10.5802/aif.2098CrossRefGoogle Scholar
Gabai, D., Foliations and the topology of 3-manifolds, J. Differential Geom. 18 (1983), 445–503.10.4310/jdg/1214437784CrossRefGoogle Scholar
Gillespie, T., L-space fillings and generalized solid tori, Preprint (2016), arXiv:1603.05016.Google Scholar
Hanselman, J., Bordered Heegaard Floer homology and graph manifolds, Algebr. Geom. Topol. 16 (2016), 3103–3166.10.2140/agt.2016.16.3103CrossRefGoogle Scholar
Hanselman, J. and Watson, L., A calculus for bordered Floer homology, Preprint (2015),arXiv:1508.05445.Google Scholar
Kazez, W. H. and Roberts, R., Approximating C 1, 0-foliations, in Interactions between low-dimensional topology and mapping class groups, Geometry & Topology Monographs, vol. 19, eds Baykur, R. I., Etnyre, J. and Hamenstädt, U. (Mathematical Sciences Publishers, Berkeley, CA, 2015), 21–72.Google Scholar
Kazez, W. H. and Roberts, R., C 0 approximations of foliations, Geom. Topol. 21 (2017), 3601–3657.10.2140/gt.2017.21.3601CrossRefGoogle Scholar
Kronheimer, P. B. and Mrowka, T. S., Monopoles and contact structures, Invent. Math. 130 (1997), 209–255.10.1007/s002220050183CrossRefGoogle Scholar
Lipshitz, R., Ozsvath, P. S. and Thurston, D. P., Bordered Heegaard Floer homology, Mem. Amer. Math. Soc. 254 (2018).Google Scholar
Lisca, P. and Stipsicz, A. I., Ozsváth-Szabó invariants and tight contact 3-manifolds. III, J. Symplectic Geom. 5 (2007), 357–384.10.4310/JSG.2007.v5.n4.a1CrossRefGoogle Scholar
Ozsváth, P. and Szabó, Z., Holomorphic disks and genus bounds, Geom. Topol. 8 (2004), 311–334.10.2140/gt.2004.8.311CrossRefGoogle Scholar
Rasmussen, S. D., L-space intervals for graph manifolds and cables, Compos. Math. 153 (2017), 1008–1049.10.1112/S0010437X16008319CrossRefGoogle Scholar
Rasmussen, J. and Rasmussen, S. D., Floer simple manifolds and L-space intervals, Adv. Math. 322 (2017), 738–805.CrossRefGoogle Scholar