Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-23T02:00:34.016Z Has data issue: false hasContentIssue false
  • English
  • Français

The K3 category of a cubic fourfold

Part of: Surfaces and higher-dimensional varieties (Co)homology theory

Published online by Cambridge University Press:  02 March 2017

Daniel Huybrechts
Daniel Huybrechts*
Affiliation:
Mathematisches Institut, Universität Bonn, Endenicher Allee 60, 53115 Bonn, Germany email [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

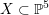Smooth cubic hypersurfaces $X\subset \mathbb{P}^{5}$ (over 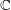$\mathbb{C}$) are linked to K3 surfaces via their Hodge structures, due to the work of Hassett, and via a subcategory 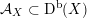${\mathcal{A}}_{X}\subset \text{D}^{\text{b}}(X)$, due to the work of Kuznetsov. The relation between these two viewpoints has recently been elucidated by Addington and Thomas. In this paper, both aspects are studied further and extended to twisted K3 surfaces, which in particular allows us to determine the group of autoequivalences of 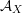${\mathcal{A}}_{X}$ for the general cubic fourfold. Furthermore, we prove finiteness results for cubics with equivalent K3 categories and study periods of cubics in terms of generalized K3 surfaces.

Keywords

cubic fourfoldK3 surfacesHodge theoryderived categories

MSC classification

Primary: 14J28: $K3$ surfaces and Enriques surfaces 14F05: Sheaves, derived categories of sheaves and related constructions
Type
Research Article
Information
Compositio Mathematica , Volume 153 , Issue 3 , March 2017 , pp. 586 - 620
Copyright
© The Author 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Addington, N., On two rationality conjectures for cubic fourfolds , Math. Res. Lett. 23 (2016), 1–13.Google Scholar
Addington, N. and Thomas, R., Hodge theory and derived categories of cubic fourfolds , Duke Math. J. 163 (2014), 1885–1927.CrossRefGoogle Scholar
Bayer, A. and Bridgeland, T., Derived automorphism groups of K3 surfaces of Picard rank 1, Duke Math. J., to appear. Preprint (2013), arXiv:1310.8266.Google Scholar
Ballard, M., Favero, D. and Katzarkov, L., Orlov spectra: bounds and gaps , Invent. Math. 189 (2012), 359–430.CrossRefGoogle Scholar
Beauville, A. and Donagi, R., La variété des droites d’une hypersurface cubique de dimension 4 , C. R. Acad. Sci. Paris Sér. I Math. 301 (1985), 703–706.Google Scholar
Bernardara, M., Macrì, E., Mehrotra, S. and Stellari, P., A categorical invariant for cubic threefolds , Adv. Math. 229 (2012), 770–803.CrossRefGoogle Scholar
Bridgeland, T., Stability conditions on K3 surfaces , Duke Math. J. 141 (2008), 241–291.Google Scholar
Bridgeland, T. and Maciocia, A., Complex surfaces with equivalent derived categories , Math. Z. 236 (2001), 677–697.Google Scholar
Buchweitz, R.-O. and Flenner, H., A semiregularity map for modules and applications to deformations , Compositio Math. 137 (2003), 135–210.CrossRefGoogle Scholar
Buchweitz, R.-O. and Flenner, H., The global decomposition theorem for Hochschild (co-)homology of singular spaces via the Atiyah–Chern character , Adv. Math. 217 (2008), 243–281.Google Scholar
Căldăraru, A., Derived categories of twisted sheaves on Calabi–Yau manifolds, PhD thesis, Cornell (2000).Google Scholar
Căldăraru, A., The Mukai paring II. The Hochschild–Kostant–Rosenberg isomorphism , Adv. Math. 194 (2005), 34–66.Google Scholar
Canonaco, A. and Stellari, P., Twisted Fourier–Mukai functors , Adv. Math. 212 (2007), 484–503.Google Scholar
Charles, F., A remark on the Torelli theorem for cubic fourfolds, Preprint (2012), arXiv:1209.4509.Google Scholar
Charles, F., Birational boundedness for holomorphic symplectic varieties, Zarhin’s trick for K3 surfaces, and the Tate conjecture , Ann. of Math. (2) 184 (2016), 487–526.Google Scholar
Cox, D., Primes of the form x 2 + ny 2 . Fermat, class field theory and complex multiplication (John Wiley & Sons, New York, 1989).Google Scholar
Galkin, S. and Shinder, E., The Fano variety of lines and rationality problem for a cubic hypersurface, Preprint (2014), arXiv:1405.5154.Google Scholar
Hassett, B., Special cubic fourfolds , Compositio Math. 120 (2000), 1–23.Google Scholar
Hille, L. and van den Bergh, M., Fourier–Mukai transforms , in Handbook of tilting theory, London Mathematical Society Lecture Note Series, vol. 332 (Cambridge University Press, Cambridge, 2007), 147–177.Google Scholar
Hosono, S., Lian, B., Oguiso, K. and Yau, S.-T., Fourier–Mukai number of a K3 surface , CRM Proc. Lecture Notes 38 (2004), 177–192.Google Scholar
Huybrechts, D., Generalized Calabi–Yau structures, K3 surfaces, and B-fields , Int. J. Math. 19 (2005), 13–36.Google Scholar
Huybrechts, D., Fourier–Mukai transforms in algebraic geometry , Oxford Mathematical Monographs (Oxford University Press, Oxford, 2006).Google Scholar
Huybrechts, D., The global Torelli theorem: classical, derived, twisted , in Algebraic geometry–Seattle 2005. Part 1, Proceedings of Symposia in Pure Mathematics, vol. 80 (American Mathematical Society, Providence, RI, 2009), 235–258.Google Scholar
Huybrechts, D., Introduction to stability conditions , in Moduli spaces, London Mathematical Society Lecture Notes Series, vol. 411 (Cambridge University Press, Cambridge, 2014), 179–229.CrossRefGoogle Scholar
Huybrechts, D., Lectures on K3 surfaces, Cambridge Studies in Advanced Mathematics (Cambridge University Press, Cambridge, 2016), http://www.math.uni-bonn.de/people/huybrech/K3.html.Google Scholar
Huybrechts, D., Macrì, E. and Stellari, P., Stability conditions for generic K3 categories , Compositio Math. 144 (2008), 134–162.Google Scholar
Huybrechts, D., Macrì, E. and Stellari, P., Derived equivalences of K3 surfaces and orientation , Duke Math. J. 149 (2009), 461–507.Google Scholar
Huybrechts, D. and Stellari, P., Equivalences of twisted K3 surfaces , Math. Ann. 332 (2005), 901–936.Google Scholar
Huybrechts, D. and Stellari, P., Proof of Căldăraru’s conjecture , in Moduli spaces and arithmetic geometry, Advanced Studies in Pure Mathematics, vol. 45, (2006), 31–42.Google Scholar
Huybrechts, D. and Thomas, R., Deformation-obstruction theory for complexes via Atiyah and Kodaira–Spencer classes , Math. Ann. 346 (2010), 545–569.Google Scholar
Kawatani, K., A hyperbolic metric and stability conditions on K3 surfaces with $\unicode[STIX]{x1D70C}=1$ , Preprint (2012), arXiv:1204.1128.Google Scholar
Kneser, M., Quadratische formen (Springer, 2002).Google Scholar
Kuznetsov, A., Derived categories of cubic and V 14 threefolds , Proc. Steklov Inst. Math. 3 (2004), 171–194; arXiv:math/0303037.Google Scholar
Kuznetsov, A., Homological projective duality for Grassmannians of lines, Preprint (2006),arXiv:math.AG/0610957.Google Scholar
Kuznetsov, A., Hochschild homology and semiorthogonal decompositions, Preprint (2009),arXiv:0904.4330.Google Scholar
Kuznetsov, A., Derived categories of cubic fourfolds , in Cohomological and geometric approaches to rationality problems, Progress in Mathematics, vol. 282 (Springer, Berlin, 2010), 219–243.CrossRefGoogle Scholar
Kuznetsov, A., Calabi–Yau and fractional Calabi–Yau categories, Preprint (2015),arXiv:1509.07657.Google Scholar
Kuznetsov, A. and Markushevich, D., Symplectic structures on moduli spaces of sheaves via the Atiyah class , J. Geom. Phys. 59 (2009), 843–860.Google Scholar
Laza, R., The moduli space of cubic fourfolds via the period map , Ann. of Math. (2) 172 (2010), 673–711.Google Scholar
Lieblich, M., Moduli of complexes on a proper morphism , J. Algebraic Geom. 15 (2006), 175–206.Google Scholar
Lieblich, M., Maulik, D. and Snowden, A., Finiteness of K3 surfaces and the Tate conjecture , Ann. Sci. Éc. Norm. Supér. 47 (2014), 285–308.Google Scholar
Looijenga, E., The period map for cubic fourfolds , Invent. Math. 177 (2009), 213–233.CrossRefGoogle Scholar
Markman, E., A survey of Torelli and monodromy results for holomorphic-symplectic varieties , in Complex and differential geometry, Proceedings in Mathematics, vol. 8 (Springer, Berlin, 2011), 257–322.Google Scholar
Macrì, E. and Stellari, P., Infinitesimal derived Torelli theorem for K3 surfaces, (Appendix by S. Mehrotra) , Int. Math. Res. Not. IMRN 2009 (2009), 3190–3220.Google Scholar
Macrì, E. and Stellari, P., Fano varieties of cubic fourfolds containing a plane , Math. Ann. 354 (2012), 1147–1176.Google Scholar
Markman, E. and Mehrotra, S., Integral transforms and deformations of K3 surfaces, Preprint (2015), arXiv:1507.03108.Google Scholar
Mukai, S., On the moduli space of bundles on K3 surfaces. I. Vector bundles on algebraic varieties (Bombay, 1984) , Tata Inst. Fund. Res. Stud. Math. 11 (1987), 341–413.Google Scholar
Nikulin, V., Integer symmetric bilinear forms and some of their geometric applications , Izv. Akad. Nauk SSSR Ser. Mat. 43 (1979), 111–177.Google Scholar
Oguiso, K., K3 surfaces via almost-primes , Math. Res. Lett. 9 (2002), 47–63.Google Scholar
Orlov, D., Equivalences of derived categories and K3 surfaces , J. Math. Sci. 84 (1997), 1361–1381.Google Scholar
Orlov, D., Derived categories of coherent sheaves and triangulated categories of singularities , in Algebra, arithmetic, and geometry: in honor of Yu. I. Manin, Vol. II, Progress in Mathematics, vol. 270 (Springer, Berlin, 2009), 503–531.Google Scholar
Reinecke, E., Autoequivalences of twisted K3 surfaces, Master thesis, Bonn (2014),http://www.math.uni-bonn.de/people/huybrech/ReineckeMA.pdf.Google Scholar
Stellari, P., Some remarks about the FM-partners of K3 surfaces with Picard numbers 1 and 2 , Geom. Dedicata 108 (2004), 1–13.CrossRefGoogle Scholar
Toda, Y., Deformations and Fourier–Mukai transforms , J. Differential Geom. 81 (2009), 197–224.Google Scholar
Toda, Y., Gepner type stability condition via Orlov/Kuznetsov equivalence, Preprint (2013), arXiv:1308.3791.Google Scholar
Toda, Y., Gepner type stability conditions on graded matrix factorizations , Algebr. Geom. 1 (2014), 613–665.Google Scholar
Voisin, C., Théorème de Torelli pour les cubiques de ℙ5 , Invent. Math. 6 (1986), 577–601.Google Scholar
Voisin, C., Correction à : ‘Théorème de Torelli pour les cubiques de ℙ5 ’ , Invent. Math. 172 (2008), 455–458.CrossRefGoogle Scholar
Yoshioka, K., Moduli spaces of twisted sheaves on a projective variety , in Moduli Spaces and Arithmetic Geometry, Advanced Studies in Pure Mathematics, vol. 45 (World Scientific, Singapore, 2006), 1–30.Google Scholar
Yoshioka, K., Stability and the Fourier–Mukai transform. II , Compositio Math. 145 (2009), 112–142.Google Scholar