Published online by Cambridge University Press: 11 September 2018
Boij–Söderberg theory characterizes syzygies of graded modules and sheaves on projective space. This paper continues earlier work with Sam, extending the theory to the setting of  $\text{GL}_{k}$-equivariant modules and sheaves on Grassmannians. Algebraically, we study modules over a polynomial ring in
$\text{GL}_{k}$-equivariant modules and sheaves on Grassmannians. Algebraically, we study modules over a polynomial ring in 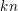 $kn$ variables, thought of as the entries of a
$kn$ variables, thought of as the entries of a 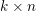 $k\times n$ matrix. We give equivariant analogs of two important features of the ordinary theory: the Herzog–Kühl equations and the pairing between Betti and cohomology tables. As a necessary step, we also extend previous results, concerning the base case of square matrices, to cover complexes other than free resolutions. Our statements specialize to those of ordinary Boij–Söderberg theory when
$k\times n$ matrix. We give equivariant analogs of two important features of the ordinary theory: the Herzog–Kühl equations and the pairing between Betti and cohomology tables. As a necessary step, we also extend previous results, concerning the base case of square matrices, to cover complexes other than free resolutions. Our statements specialize to those of ordinary Boij–Söderberg theory when  $k=1$. Our proof of the equivariant pairing gives a new proof in the graded setting: it relies on finding perfect matchings on certain graphs associated to Betti tables and to spectral sequences. As an application, we construct three families of extremal rays on the Betti cone for
$k=1$. Our proof of the equivariant pairing gives a new proof in the graded setting: it relies on finding perfect matchings on certain graphs associated to Betti tables and to spectral sequences. As an application, we construct three families of extremal rays on the Betti cone for  $2\times 3$ matrices.
$2\times 3$ matrices.
Current address: Mathematics Department, University of Washington, Seattle, WA, USA
The second author was supported by a Rackham Predoctoral Fellowship and by NSERC grant PDF-502633.