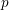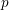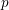Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Hamacher, Paul
and
Kim, Wansu
2019.
l-Adic étale cohomology of Shimura varieties of Hodge type with non-trivial coefficients.
Mathematische Annalen,
Vol. 375,
Issue. 3-4,
p.
973.
Bhatt, Bhargav
Iyengar, Srikanth B.
and
Ma, Linquan
2019.
Regular rings and perfect(oid) algebras.
Communications in Algebra,
Vol. 47,
Issue. 6,
p.
2367.
Howard, Benjamin
2020.
On the Ekedahl–Oort stratification of Shimura curves.
Algebra & Number Theory,
Vol. 14,
Issue. 4,
p.
961.
Bijakowski, Stéphane
and
Hernandez, Valentin
2023.
ON THE GEOMETRY OF THE PAPPAS–RAPOPORT MODELS FOR PEL SHIMURA VARIETIES.
Journal of the Institute of Mathematics of Jussieu,
Vol. 22,
Issue. 5,
p.
2403.
van Hoften, Pol
2024.
Mod p points on shimura varieties of parahoric level.
Forum of Mathematics, Pi,
Vol. 12,
Issue. ,