Hostname: page-component-cd9895bd7-p9bg8 Total loading time: 0 Render date: 2024-12-23T01:57:05.740Z Has data issue: false hasContentIssue false
  • English
  • Français

The Craighero–Gattazzo surface is simply connected

Part of: Families, fibrations Surfaces and higher-dimensional varieties

Published online by Cambridge University Press:  28 February 2017

Julie Rana ,
Jenia Tevelev  and
Giancarlo Urzúa
Julie Rana
Affiliation:
School of Mathematics, University of Minnesota, 127 Vincent Hall, 206 Church Street SE, Minneapolis, MN 55455, USA email [email protected]
Jenia Tevelev
Affiliation:
Department of Mathematics and Statistics, Lederle Graduate Research Tower, 1623D, University of Massachusetts Amherst, 710 North Pleasant Street, Amherst, MA 01003-9305, USA email [email protected]
Giancarlo Urzúa
Affiliation:
Facultad de Matemáticas, Pontificia Universidad Católica de Chile, Campus San Joaquín, Avenida Vicuña Mackenna 4860, Santiago, Chile email [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We show that the Craighero–Gattazzo surface, the minimal resolution of an explicit complex quintic surface with four elliptic singularities, is simply connected. This was conjectured by Dolgachev and Werner, who proved that its fundamental group has a trivial profinite completion. The Craighero–Gattazzo surface is the only explicit example of a smooth simply connected complex surface of geometric genus zero with ample canonical class. We hope that our method will find other applications: to prove a topological fact about a complex surface we use an algebraic reduction mod 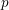$p$ technique and deformation theory.

Keywords

Godeaux surfacesfundamental groupdeformation theorymoduli space

MSC classification

Primary: 14J10: Families, moduli, classification 14J29: Surfaces of general type
Secondary: 14J25: Special surfaces 14D06: Fibrations, degenerations
Type
Research Article
Information
Compositio Mathematica , Volume 153 , Issue 3 , March 2017 , pp. 557 - 585
Copyright
© The Authors 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abramovich, D. and Hassett, B., Stable varieties with a twist , in Classification of algebraic varieties, Proceedings of the 2009 conference at Schiermonnikoog the Netherlands, eds Faber, C., van der Geer, G. and Looijenga, E. J. N. (European Mathematical Society, Zürich, 2011), 1–38.Google Scholar
Artin, M., Algebraization of formal moduli. I , in Global analysis (Papers in Honor of K. Kodaira) (University of Tokyo Press, Tokyo, 1969), 21–71.Google Scholar
Artin, M., Versal deformations and algebraic stacks , Invent. Math. 27 (1974), 165–189.Google Scholar
Ashikaga, T. and Konno, K., Examples of degenerations of Castelnuovo surfaces , J. Math. Soc. Japan 43 (1991), 229–246.Google Scholar
Bǎdescu, L., Algebraic surfaces, Universitext (Springer, Berlin, 2001).CrossRefGoogle Scholar
Barlow, R., A simply connected surface of general type with p g = 0 , Invent. Math. 79 (1985), 293–301.Google Scholar
Bauer, I., Catanese, F. and Pignatelli, R., Surfaces of general type with geometric genus zero: a survey , in Complex and differential geometry, Proceedings in Mathematics, vol. 8 (Springer, New York, 2011), 1–48.Google Scholar
Baykur, I. and Korkmaz, M., Small Lefschetz fibrations and exotic 4-manifolds, Math. Ann. (2016), to appear, doi:10.1007/s00208-016-1466-2. Preprint (2015), arXiv:1510.00089.CrossRefGoogle Scholar
Catanese, F. and Pignatelli, R., On simply connected Godeaux surfaces , in Complex analysis and algebraic geometry (de Gruyter, Berlin, 2000), 117–153.Google Scholar
Cossec, F. R. and Dolgachev, I., Enriques surfaces I , Progress in Mathematics, vol. 76 (Birkhäuser, Boston, MA, 1989).Google Scholar
Craighero, P. C. and Gattazzo, R., Quintic surfaces of ℙ3 having a nonsingular model with q = p g = 0, P 2≠0 , Rend. Sem. Mat. Univ. Padova 91 (1994), 187–198.Google Scholar
Dolgachev, I. and Werner, C., A simply connected numerical Godeaux surface with ample canonical class , J. Algebraic Geom. 8 (1999), 737–764; Erratum: J. Algebraic Geom. 10 (2001) 397.Google Scholar
Grothendieck, A. and Dieudonné, J., Eléments de géometrie algébrique: III. Étude cohomologique des faisceaux cohérents, Première partie , Publ. Math. Inst. Hautes Études Sci. 11 (1961), 5–167.Google Scholar
Grothendieck, A. and Dieudonné, J., Eléments de géométrie algébrique: IV. Étude locale des schémas et des morphismes de schémas, Quatriéme partie , Publ. Math. Inst. Hautes Études Sci. 32 (1966), 5–361.Google Scholar
Fintushel, R. and Stern, R., Families of simply connected 4-manifolds with the same Seiberg–Witten invariants , Topology 43 (2004), 1449–1467.Google Scholar
Freedman, M., The topology of four-dimensional manifolds , J. Differential Geom. 17 (1982), 357–453.Google Scholar
Hacking, P., Compact moduli of plane curves , Duke Math. J. 124 (2004), 213–257.Google Scholar
Kawamata, Y., Moderate degenerations of algebraic surfaces , in Complex algebraic varieties (Bayreuth, 1990), Lecture Notes in Mathematics, vol. 1507 (Springer, Berlin, 1992), 113–132.Google Scholar
Kollár, J., Shafarevich maps and plurigenera of algebraic varieties , Invent. Math. 113 (1993), 177–215.Google Scholar
Kollár, J. and Shepherd-Barron, N. I., Threefolds and deformations of surface singularities , Invent. Math. 91 (1988), 299–338.Google Scholar
LeBrun, C. and Catanese, F., On the scalar curvature of Einstein manifolds , Math. Res. Lett. 4 (1997), 843–854.Google Scholar
Lee, Y., Interpretation of the deformation space of a determinantal barlow surface via smoothings , Proc. Amer. Math. Soc. 130 (2002), 963–969.CrossRefGoogle Scholar
Lee, Y. and Nakayama, N., Simply connected surfaces of general type in positive characteristic via deformation theory , Proc. Lond. Math. Soc. (3) 106 (2013), 225–286.CrossRefGoogle Scholar
Lee, Y. and Park, J., A simply connected surface of general type with p g = 0 and K 2 = 2 , Invent. Math. 170 (2007), 483–505.CrossRefGoogle Scholar
Lyndon, R. C. and Schupp, P. E., Combinatorial group theory (Springer, New York, 1977).Google Scholar
Mumford, D., Abelian varieties (Tata Institute of Fundamental Research, Bombay, 1970).Google Scholar
Park, H., Park, J. and Shin, D., A simply connected surface of general type with p g = 0 and K 2 = 3 , Geom. Topol. 13 (2009), 743–767.CrossRefGoogle Scholar
Park, H., Park, J. and Shin, D., A simply connected surface of general type with p g = 0 and K 2 = 4 , Geom. Topol. 13 (2009), 1483–1494.CrossRefGoogle Scholar
Park, H., Shin, D. and Urzúa, G., A simply connected numerical Campedelli surface with an involution , Math. Ann. 357 (2013), 31–49.Google Scholar
Rana, J., A boundary divisor in the moduli space of stable quintic surfaces, Internat. J. Math., to appear. Preprint (2014), arXiv:1407.7148.Google Scholar
Ribes, L. and Zalesskii, P., Profinite groups , Ergebnisse der Mathematik und ihrer Grenzgebiete. 3. Folge [A Series of Modern Surveys in Mathematics], vol. 40, second edition (Springer, Berlin, 2010).Google Scholar
Schlessinger, M., Functors of Artin rings , Trans. Amer. Math. Soc. 130 (1968), 208–222.Google Scholar
Grothendieck, A. and Raynaud, M., Revêtements etales et groupe fondamental: séminaire de géométrie algébrique de Bois-Marie 1960/61 (SGA1), Lecture Notes in Mathematics, vol. 224 (Springer, Berlin, 1971).CrossRefGoogle Scholar
Stern, A. and Urzúa, G., KSBA surfaces with elliptic quotient singularities, 𝜋1 = 1, p g = 0, and K 2 = 1, 2 , Israel J. Math. 214 (2016), 651–673, doi:10.1007/s11856-016-1350-5.Google Scholar
Wahl, J., Equisingular deformations of normal surface singularities , Ann. of Math. (2) 104 (1976), 325–356.CrossRefGoogle Scholar
Wahl, J., Smoothings of normal surface singularities , Topology 20 (1981), 219–246.Google Scholar
Xiao, G., 𝜋1 of elliptic and hyperelliptic surfaces , Internat. J. Math. 2 (1991), 599–615.Google Scholar