Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Sun, Shi
Huang, Ziping
and
Wang, Cheng
2018.
Weak Galerkin finite element method for a class of quasilinear elliptic problems.
Applied Mathematics Letters,
Vol. 79,
Issue. ,
p.
67.
Sun, Jiguang
Zhang, Qian
and
Zhang, Zhimin
2019.
A curl-conforming weak Galerkin method for the quad-curl problem.
BIT Numerical Mathematics,
Vol. 59,
Issue. 4,
p.
1093.
Zhao, Lina
Park, Eun-Jae
and
Chung, Eric T.
2020.
Staggered discontinuous Galerkin methods for the Helmholtz equation with large wave number.
Computers & Mathematics with Applications,
Vol. 80,
Issue. 12,
p.
2676.
Hu, Qingjie
He, Yinnian
Li, Tingting
and
Wen, Jing
2020.
A Mixed Discontinuous Galerkin Method for the Helmholtz Equation.
Mathematical Problems in Engineering,
Vol. 2020,
Issue. ,
p.
1.
Al-Taweel, Ahmed
and
Wang, Xiaoshen
2020.
The lowest-order stabilizer free weak Galerkin finite element method.
Applied Numerical Mathematics,
Vol. 157,
Issue. ,
p.
434.
Liu, Ying
and
Nie, Yufeng
2021.
A priori and a posteriori error estimates of the weak Galerkin finite element method for parabolic problems.
Computers & Mathematics with Applications,
Vol. 99,
Issue. ,
p.
73.
Mu, Lin
2021.
Weak Galerkin finite element with curved edges.
Journal of Computational and Applied Mathematics,
Vol. 381,
Issue. ,
p.
113038.
AL-Taweel, Ahmed
and
Mu, Lin
2021.
A new upwind weak Galerkin finite element method for linear hyperbolic equations.
Journal of Computational and Applied Mathematics,
Vol. 390,
Issue. ,
p.
113376.
Xie, Yingying
and
Zhong, Liuqiang
2021.
Convergence of Adaptive Weak Galerkin Finite Element Methods for Second Order Elliptic Problems.
Journal of Scientific Computing,
Vol. 86,
Issue. 1,
Lafontaine, D.
Spence, E. A.
and
Wunsch, J.
2022.
A sharp relative-error bound for the Helmholtz h-FEM at high frequency.
Numerische Mathematik,
Vol. 150,
Issue. 1,
p.
137.
Xie, Yingying
Zhong, Liuqiang
and
Zeng, Yuping
2022.
Convergence of an adaptive modified WG method for second-order elliptic problem.
Numerical Algorithms,
Vol. 90,
Issue. 2,
p.
789.
Tang, Ming
Zhong, Liuqiang
and
Xie, Yingying
2023.
A modified weak Galerkin method for (curl)-elliptic problem.
Computers & Mathematics with Applications,
Vol. 139,
Issue. ,
p.
224.
Gu, Shanshan
Chai, Shimin
Zhou, Chenguang
and
Zhou, Jinhui
2023.
Weak Galerkin finite element method for linear poroelasticity problems.
Applied Numerical Mathematics,
Vol. 190,
Issue. ,
p.
200.
Yingying, Xie
Cao, Shuhao
Chen, Long
and
Zhong, Liuqiang
2023.
Convergence and optimality of an adaptive modified weak Galerkin finite element method.
Numerical Methods for Partial Differential Equations,
Vol. 39,
Issue. 5,
p.
3847.
Gu, Yiqi
and
Shen, Jie
2023.
A Fictitious Domain Spectral Method for Solving the Helmholtz Equation in Exterior Domains.
Journal of Scientific Computing,
Vol. 94,
Issue. 3,
Li, Buyang
Li, Yonglin
and
Yang, Zongze
2024.
An optimized CIP-FEM to reduce the pollution errors for the Helmholtz equation on a general unstructured mesh.
Journal of Computational Physics,
Vol. 511,
Issue. ,
p.
113120.
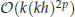 under mesh condition k7/2h2≤C0 or (kh)2+k(kh)p+1≤C0, which coincides with the phase error of the finite element method obtained by existent dispersion analyses. Here h is the mesh size, p is the order of the approximation space and C0 is a constant independent of k and h. Furthermore, numerical tests are provided to verify the theoretical findings and to illustrate the great capability of the WG-FEM in reducing the pollution effect.
under mesh condition k7/2h2≤C0 or (kh)2+k(kh)p+1≤C0, which coincides with the phase error of the finite element method obtained by existent dispersion analyses. Here h is the mesh size, p is the order of the approximation space and C0 is a constant independent of k and h. Furthermore, numerical tests are provided to verify the theoretical findings and to illustrate the great capability of the WG-FEM in reducing the pollution effect.